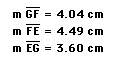Assignment #9

By Nikki Masson

Pedal Triangles

Part 1: Constructing a Pedal Triangle:

Step 1: Draw a triangle.

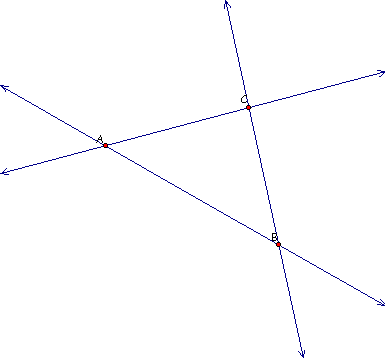
Step 2: Pick a point anywhere and name that the Pedal Point, the point can be inside or outside of the triangle.

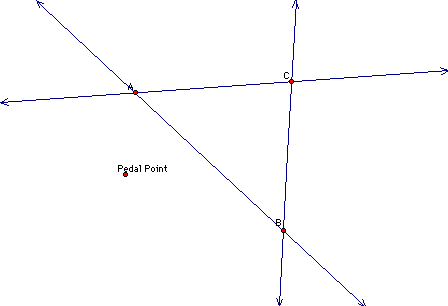
Step 3: Draw perpendicular lines from the pedal point to the three sides of the triangle and mark the intersection points.

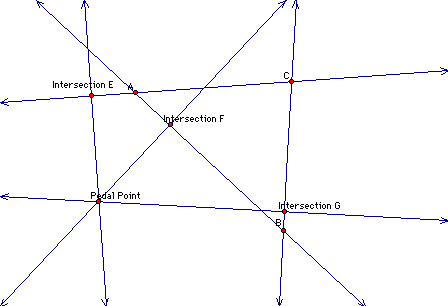
Step 4: The pedal triangle is constructed using the three intersection points.

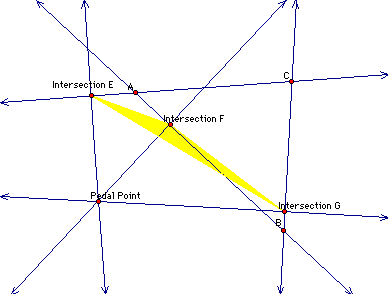
So Triangle EFG is the Pedal Triangle for the Pedal Point.

Part 2: Explore the Pedal Triangle:

To explore the Pedal Triangle and how is changes as you move the Pedal Point, use the Pedal Triangle GSP script tool.

Explorations:

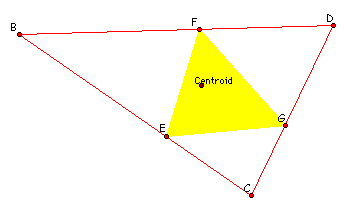
1. Construct the centroid of the triangle and explore the Pedal Triangle when the Pedal Point is merged with the centroid.

 

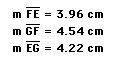
I merged the Centroid with the Pedal Point and in the above picture, the yellow triangle is the Pedal Triangle. It appears that the Pedal Triangle may be an equilateral triangle, but when we measure the sides of the triangle, it is not an equilateral triangle.

2. Construct the Orthocenter and explore the Pedal Triangle when the Pedal Point is merged with the Orthocenter.

The yellow triangle is the Pedal Triangle when the original triangle is obtuse.

The Pedal Triangle becomes a line when the original triangle is a right triangle and the orthocenter is merged with the Pedal Point.

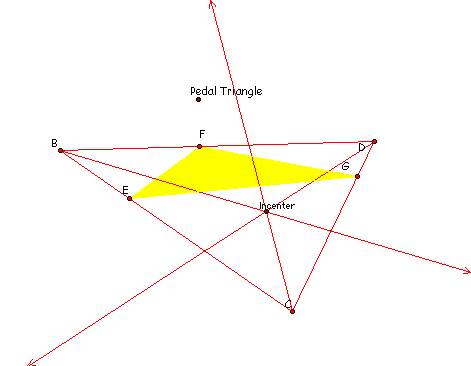
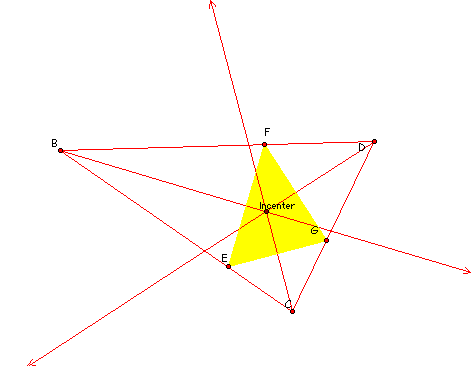
3. Construct the Incenter and explore the Pedal Triangle when the Pedal Point is merged with the Incenter.

Below, the Incenter is merged with the Pedal Point.

It looks like the Pedal Triangle may be equilaterial, so let's measure the lengths of the side.

So the triangle is not equilaterial.

Return