By using Geometer's Sketchpad, we can explore
different ratios of segments and their relationships in triangles.
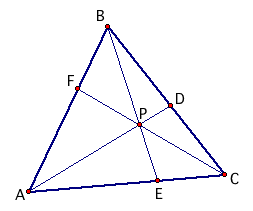
Consider any triangle ABC. Select a point P inside the traingle and draw lines AP, BP, and CP extended to their intersections with the opposite sides in points D, E, and F respectively. Explore (AF)(BD)(CE) and (FB)(DC)(EA) for various traingles and various locations of P.
Let's draw our triangle to begin our exploration.

Now, let's calculate the lengths of different segments on our triangle and explore (AF)(BD)(CE) and (FB)(DC)(EA).
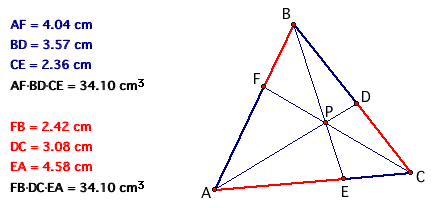
Notice that our values for (AF)(BD)(CE) and (FB)(DC)(EA) are the same, 34.10 cubic centemeters. This is true for any triangle ABC as well as any point P inside that triangle. Click here to change the size and shape of triangle ABC, or the location of point P, and see what happens to our calculated values.
Regarless of the size or shape of triangle
ABC, and when point P lies inside that triangle, (AF)(BD)(CE)=(FB)(DC)(EA).
In other words, 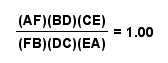
Conjecture? Prove it. Can the results be generalized (using lines rather than segments to construct triangle ABC) so that point P can be outside the triangle?
From the calculations above we have shown that
(AF)(BD)(EC) = (FB)(DC)(EA), or: 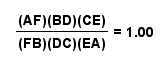
In order to show that the ratio above is equal to 1.00, we need to look at various ratio relationships for all of the sides of triangle ABC. For that, it is helpful to investigae to similar triangles.
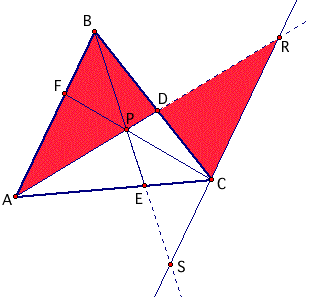
First, let's draw a line at point C that is parallel to line segment AB. Then, extend the line segments AD and BE to intersect with our new parallel line. Where these lines intersect, label the points R and S respectively.
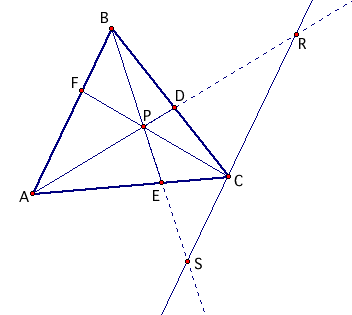
Now we can see some similar triangles that will be useful for our calculations:
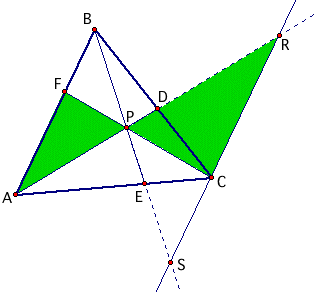 |
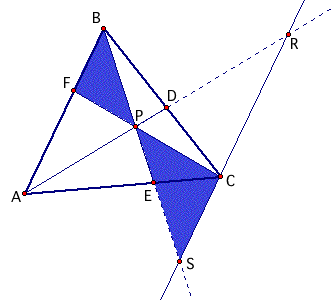 |
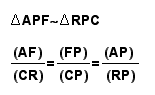 |
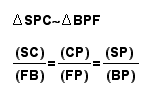 |
And even more similar triangles:
 |
 |
 |
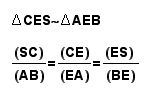 |
Now, consider our equation:
We can begin substituting some of the equivalent ratios above in order to simplify our equation. Our goal is to get like terms to cancel, so we can see that our equation does in fact equal 1.00.
First, rewrite the equation to be ready for substitution:
Using the ratios above, we can begin our substitutions. The values of (BD)/(DC) is found in the red similar triangles above. The values of (CE)/(EA) is found in the yellow similar triangles above. By substitution, we have:
Cancel the common term AB from the numerator and denominator and rewrite the equation. So:
In order to get more useful ratios for substitution, we must switch the denominators. We are able to do this since we are multiplying terms, and multiplication is commutative here. Hence, we have:
Now we have another ratio that can be substituted into our equation. The value of (AF)/(CR) can be found in the green similar triangles above. Also, value for (SC)/(FB) is found in the blue similar triangles. By subtitution, we have:
Notice that the numerator and the denominator are the same. By cancelling like terms we can see that:
Therefore, we have proved that:
Can the results be generalized so that point P can lie outside the triangle? Click here to see another page of exploration.
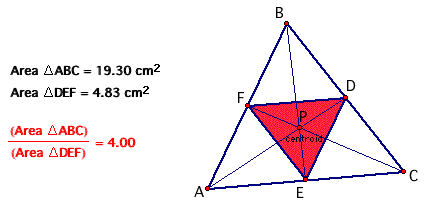
Show that when P is inside traingle ABC, the ratio of the areas of triangle ABC and triangle DEF is always greater than or equal to 4. When is it equal to 4?
Let's draw our triangle to begin our exploration.

Calculating the areas of triangle ABC and triangle DEF shows us that the ratio of areas is always greater than or equal to 4.00. This is true for when P is inside of the triangle, as shown in both examples below.
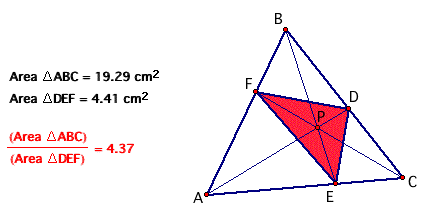

Explore the ratio of the areas by moving point P around inside the triangle. Can you find a location for P where the ratio of the areas of our triangle will be less than 4? At what point P does the ratio of the areas of our triangles exactally equal 4?
The ratio of our triangle areas will exactally equal 4 at the centroid of triangle ABC. Why do you think this is the case? It is helpful to remember how the centroid of a triangle is constructed. Explore the ratio of areas by moving P to the centroid here. Then, change the size of traingle ABC and move P back to the centroid. So, regardless of the shape and size of triangle ABC, when point P is at the centroid, the ratio of the areas of our triangles will always be 4!