Assignment 8 Problems 1 to 8, 9, 10, 11 Cristina Aurrecoechea Fall 2005

(Problem 9 was addressed in Assignment 4.)

Exploring triangles HAB, HBC, HAC, their orthocenters, and their nine point circles

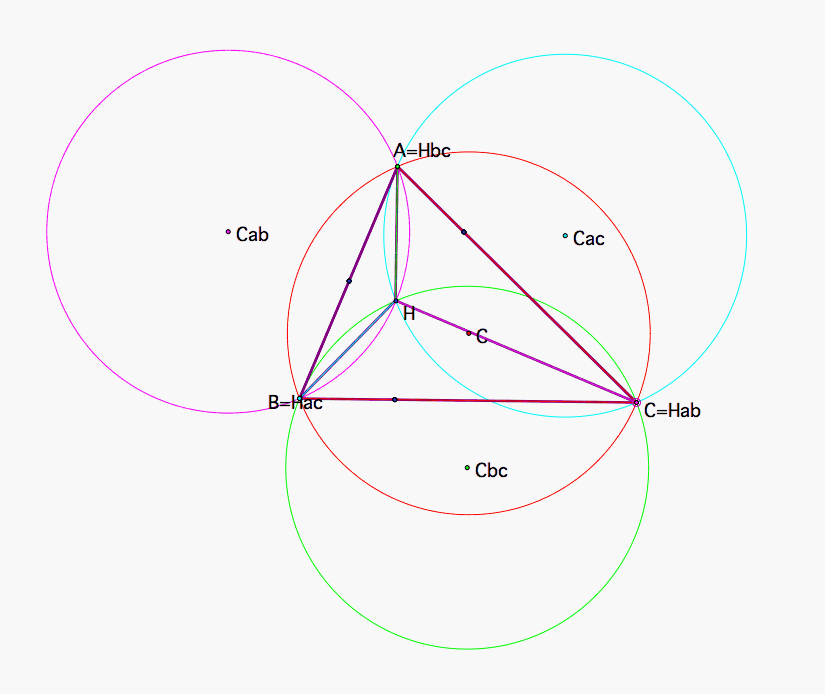
Figure 1 shows an acute triangle, its circumcircle at center C, and the three triangles formed between its orthocenter H and its vertices, These triangles HAB, HAC, HBC are shown, their corresponding cicumcircles at centers Cab, Cac, Cbc and their orthocenters Hab, Hac, Hbc.

Observations:

• Orthocenter Hab of triangle HAB coincides with the vertex C in the original triangle
• Orthocenter Hbc of triangle HBC coincides with the vertex A in the original triangle
• Orthocenter Hac of triangle HAC coincides with the vertex B in the original triangle
• All four circumcircles have same radius.

The triangle with vertices the circumcenters is similar to the original; its orthocenter is the circumcenter C of the original triangle, its circumcenter is the orthocenter H of the original triangle. Here is the gsp file to explore these triangles.

If the original triangle is acute, all three interior H-triangles are obtuse, reason why their orthocenters exist outside of them, at a distance that depends on how obtuse is the angle. In Figure 1, if you make the original triangle obtuse by pushing vertex A down, its orthocenter H will move upwards, while Hbc will move downwards as the triangle HBC becomes acute.

If we trace the nine point circle for these triangles, we observe that the four triangles share the same nine point circle, which seems obvious when you realize that the four triangles share the same nine points.

Exploring the triangle formed by the points where the extended altitudes meet the circumcircle

Figure 2 shows this new triangle PQR and the orthic triangle DEF.

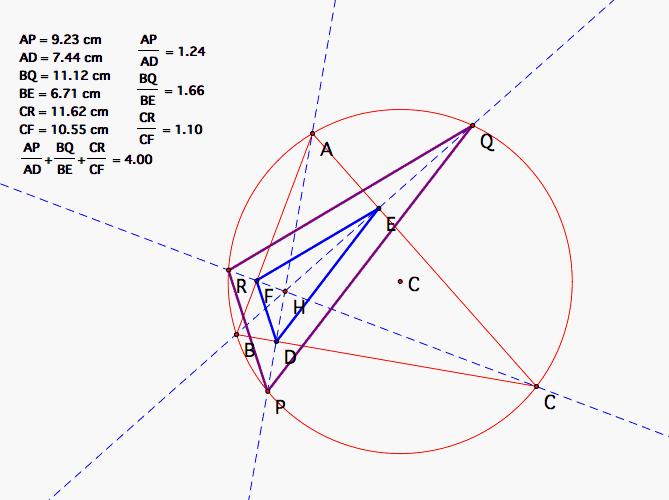
Observations:

• Both triangles are similar, as long as the original triangle is acute.
• The orthocenter in original triangle becomes the incenter of both triangles, that is, the altitudes become angle bisectors.
• It is obvious that the triangle PQR shares circumcircle (and circumcenter) with the original triangle.

The relationship between segments shown above (sum equals 4) do not hold if the original triangle is obtuse. Check with this gsp file. It is easy to see why the sum is 4 when the triangle is equlilateral as in Figure 3.

In an equlateral triangle AH=BH=CH=circumradius R, is sqrt(3)/3 while HD=HE=HF=inradius r, is sqrt(3)/6, therefore R=2r. Therefore DP=HD, EQ=HE and FR=HF. So each proportion calculated above is 4/3, and the sum is 4. To prove that this relationship holds for any acute triangle, we need to find how the relationships R/r varies as the angle is other than PI/3 and varies in each vertex.

Return to Cristina's page with all the assignments.