Transformation of Parabolas

by

Jeffrey R. Frye

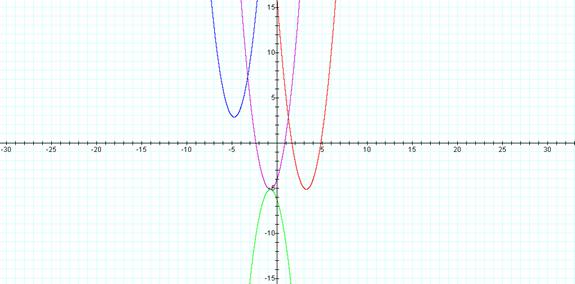
This discussion and illustration will show how changing the equation will change the display of the graph. The basic equation will be adjusted by a constant that will change the position of the graph relative to the x-axis and then a different constant that will change the position relative to the y-axis. The graph will then be reflected about the original vertex. The original equation is:

When the graph is adjusted by substituting (x-4) for x, the graph is translated 4 units to the right. This is the red graph.

In order to move the graph to the 2^nd quadrant the value (x+4) is substituted for x and a constant of 4 replaces the -4. The +4 translates the graph up 4 units and the (x+4) translates the original graph 4 units to the left. This is the blue graph.

After changing the equation to vertex form, it is easier to see that changing the sign of the coefficient preceding the x will reflect the graph about the original vertex. The original equation in vertex form is shown below. Changing the sign to negative (-2) results in the reflection. This is the green graph.

The graphs show the effects of the above transformations.

To explore these transformations using Graphing Calculator, click here.

Return to Frye main page.