Final Assignment

Jeffrey R. Frye

Part Two

Bouncing Barney

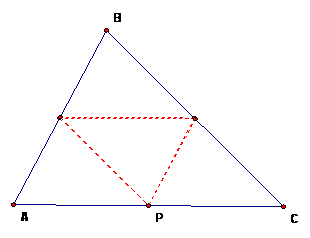
In this write up I used GSP to explore some of the different possibilities for Barney in the triangular room. In case 1, I constructed the triangle and had Barney start at the midpoint of one side. When he begins at the midpoint, he will hit each midpoint and touch each side once for a total of three times. What occurs in this illustration is that Barney walks along the midsegment each time. The result of this drawing is that it appears that four congruent triangles are formed. This special case is shown below. Barney starts at point P and travels along the midsegments shown by the dotted path.

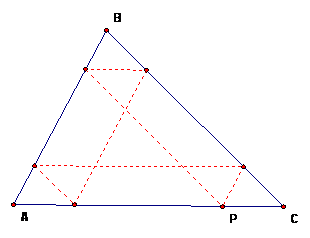
In the general condition where Barney starts at any given point on one side, and then travels parallel to one wall until he reaches another wall, he will make contact at six points and then continually repeat that path. This illustration is shown below, where Barney starts at point P. It appears that congruent triangles are formed again. In one case the triangles appear to be all equal, nine congruent triangles. In another, the smaller triangles nearer each vertex appear to be congruent. Compare the illustrations below.

In another exploration, Barney starts outside the room and follows the same approach to meeting the walls six times. In another example, if Barney does not walk parallel to a wall on his first try, but follows with the parallel walk, he will connect six times before repeating. The difference is that he does not return to his original starting point. See the illustrations below.

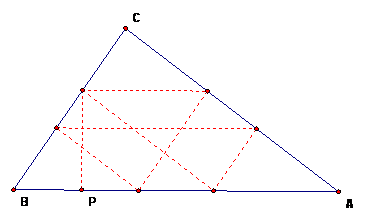
Except for the special conditions shown, based upon the constructions shown, Barney will return to his original starting point on the sixth contact.

Do your own exploration on GSP.

Multiple Solutions

In this problem an exploration using graphing calculator was done to find possible solutions to the two equations. One approach is to solve equations of the form shown below.

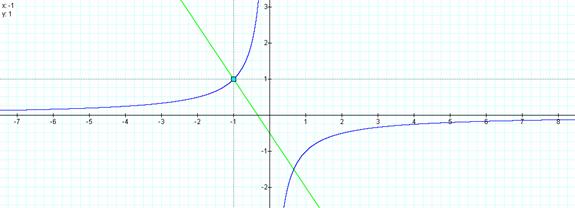
Using graphing calculator and varying the value of c, we can see that for values except there will be two different solutions. The value of c will determine whether x or y is positive or negative. When c is negative, either x or y must also be negative and the other must be positive. An all integer solution occurs when c=-4, x=-1, and y=1.

Explore using graphing calculator.

Another interesting graph can be explored using graphing calculator using a three dimensional view.

Explore this graph using graphing calculator.

Stamp problem

This problem was previously considered in assignment 12 covering spreadsheets. By adding the new price increase of 2006 to $.39, the analysis is changed to the updated values shown below.

1919	2
1932	3
1958	4
1963	5
1968	6
1971	8
1974	10
1975	13
1978	15
1981	20
1985	22
1988	25
1991	29
1994	32
1997	33
1999	34
2002	37
2006	39

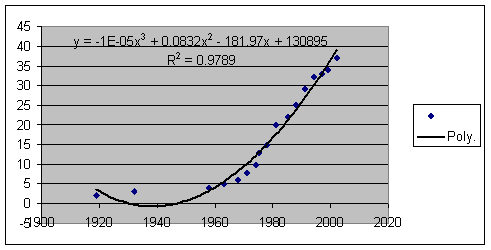
The curve still has a high correlation value. The prediction made by this exploration is that the cost of the stamp will be $.74 in 2037, and the cost will be $1.00 in 2068. The curve predicts an increase in 2007 of $.03. The model saying that postage rates doubled every ten years seems to not hold true. Even in the early years of the data, the price did not hold to this model. During the years from 1961 to 1981, this doubling every ten years seemed to hold true. An analysis using the model above could provide insight into the direction of future increases. However, since it is based upon past data, the future may hold something completely different. It may be a good starting point.

Return to main page