The circumcenter is defined as the point at which the three perpendicular bisectors of a triangle meet. Let's prove that the three perpendicular bisectors of any triangle really do meet in a single point.
First, let's find equations for the perpendicular bisectors of a triangle.
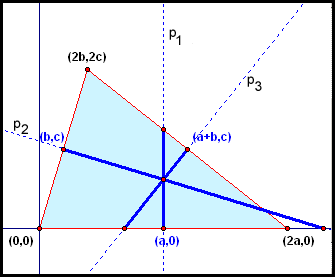
By our calculations for the orthocenter, we know
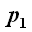 is vertical,
is vertical,
the slope of 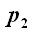 is
is 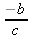 ,
,
and the slope of  is
is  .
.
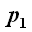 passes through the point (a, 0), so
passes through the point (a, 0), so 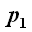 is the line described by
is the line described by
x = a.
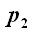 passes through the point (b, c), so
passes through the point (b, c), so 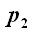 is the line described by
is the line described by
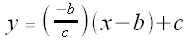
 passes through the point (a + b, c), so
passes through the point (a + b, c), so  is the line described by
is the line described by
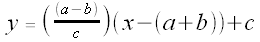
Let's find the point  at which
at which 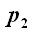 and
and  intersect, and then we can determine whether
intersect, and then we can determine whether  lies on
lies on 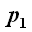 as well. If it does, we will have shown that all three perpendicular bisectors intersect in a single point.
as well. If it does, we will have shown that all three perpendicular bisectors intersect in a single point.
We need to find a point  that is on both
that is on both 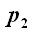 and
and 
The y-coordinate of both lines must be the same at x = 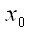 .
.
So we must have:

And

Does our point  lie on
lie on 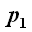 ? Let's find out.
? Let's find out.
The x-coordinate of any point on 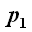 is a.
is a.
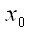 = a, so
= a, so  does indeed lie on
does indeed lie on 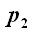 .
.
|
So for any triangle, all three perpendicular bisectors intersect at a single point, the circumcenter.
For a triangle whose vertices are (0,0), (2a,0), and (2b,2c), the coordinates of the circumcenter are

|
<< Previous (Orthocenter)
Return to my page
Return to EMAT 6680 page


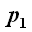 is vertical,
is vertical,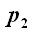 is
is 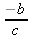 ,
, is
is  .
.
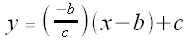
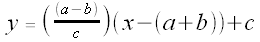
 at which
at which 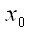 .
.


