A conic or conic section, is the locus of points that is formed when a plane intersects a cone. The types of conics that we investigate include circles, ellipses, parabolas, and hyperbolas. The general form for a conic can be given by the equation Ax^2 + Bxy + Cy^2 + Dx + Ey + F = 0. The type of conic that is formed can be determined by the coefficients. That is to say, if B^2 - 4AC < 0 then the equation is that of an ellipse or a circle. If B^2 - 4AC > 0 the conic formed would be a hyperbola or two intersecting lines. Finally, if B^2 - 4AC = 0 a parabola or two parallel lines would be the conic.
Knowing this information, when I came across the equation
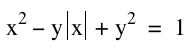
I immediately thought of the general form of a conic with A = 1, B = -1, C = 1, D = E = 0, and F = -1. Furthermore, it seemed to me that the graph should resemble an ellipse since (-1)^2 - 4(1)(1) = -3 < 0. But what would the absolute value do?
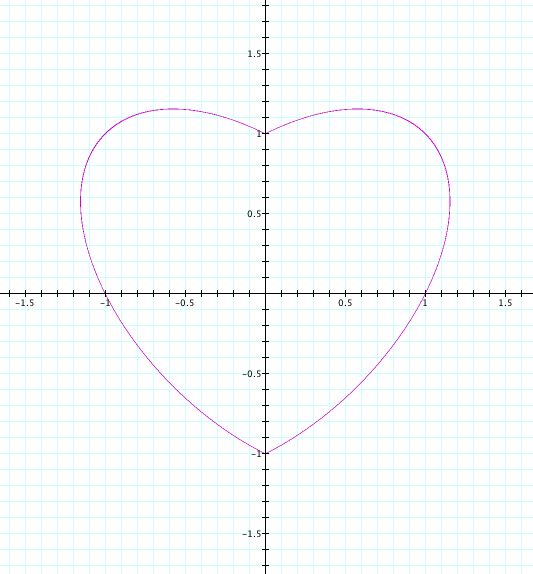
Once I saw this graph, it made perfect sense! The x values would always be positive, so the graph in the second and third quadrants would simply be mirror image of the graph in the first and fourth quadrants, hence making a heart. Using this as my starting point, I began to vary A, B, and C.
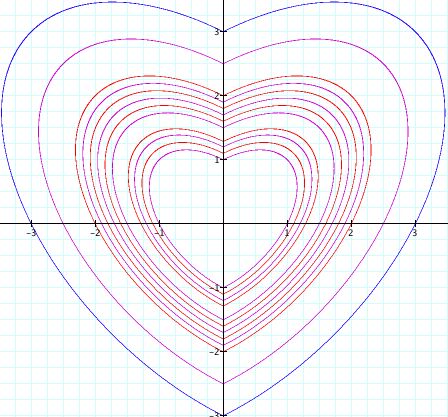
The first one that I experimented with was B. When B = 0, the graph is a circle of radius 1 with the center at the origin. As B became larger, the circle begins to look more and more like a heart. Finally, when B = 2, there were two parallel sets of lines reflected across the y-axis. Below is a graph with B = .25 , .5, .75, 1, 1.25, 1.5, 1.75, and 2.

Why did our heart become parallel lines? If the absolute value is removed, the equation would become x^2 - 2xy + y^2 = 1, which can factor into (x - y)^2 = 1. This implies that x - y = 1 or x - y = -1, two parallel lines! But what if B > 2? In this case, B^2 - 4AC > 0 so we would expect to see some hyperbolas. Notice in the graphs on the left, I have left out the absolute value so that the hyperbola is more clearly seen. The graph on the right shows our original equation (with the absolute value) for B = 2, 2.5, 3, 4, and 5.
 |
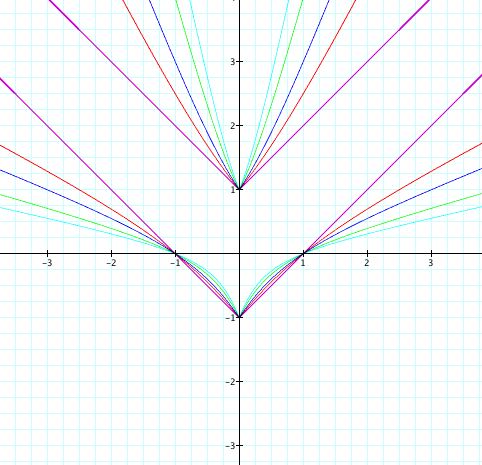 |
(Note: If B < 0, the same phenomenon would occur with the exception that all images would be reflected over the x-axis).
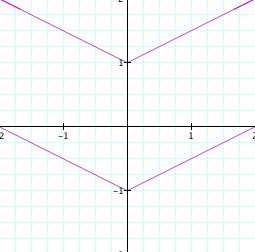
Next I investigated what would happen as A varied. As long as I kept B = -1, the only things that changed from the original heart were the x-intercepts. That is to say, the y-intercepts remained fixed at (0,1) and (0,-1) while the x-intercepts varied as (1/A,0) and (-1/A,0). So it stood to reason that as A approached 0, the x-intercepts would approach infinity. Furthermore, if A = 0.25, the equation could be factored into (0.5x - y)^2 = 1 so there would again be two lines instead of a heart at this point. Finally, following the same logic from the B investigation, I noticed that when 0 < A < 0.25, hyperbolas would form since B^2 - 4AC > 0. Below are some graphs of these conclusions but I encourage you to get on Graphing Calculator to see it for yourself.
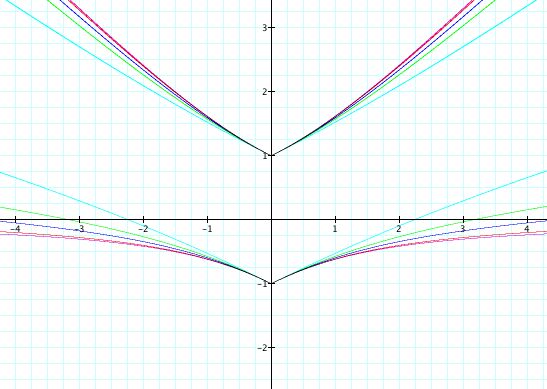 |
 |
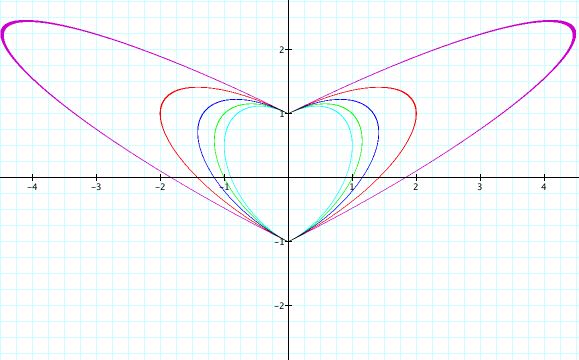 |
But what happens when A < 0? t turns out that we still have hyperbolas since B^2 - 4AC > 0. These hyperbolas however have steeper slant asymptotes and hence start to look much different. Below I have included graphs of the general conic as well as the equation with the absolute value of x for A = 0, -1, -2, -3, and -4.
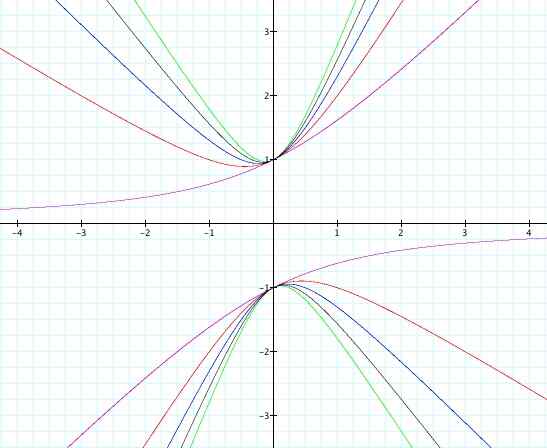 |
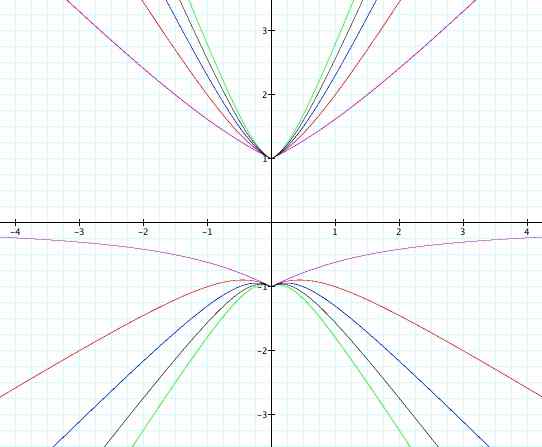 |
A very similar analysis can be done for changes in C. The only difference would be that the x-intercepts would remain fixed and the y-intercepts would be changing. Furthermore, when C = 0.25 we have the two parallel line phenomenon as above.
My final investigation was to vary F. In the original equation, F = -1. I found that if F > 0, there would be no graph. Furthermore, the x- and y-intercepts were determined by the square root of |F|. When I noticed this I was able to make a pretty picture that I was thinking of using as this years Valentine's day card. Feel free to steal my idea for your own sweetheart.