

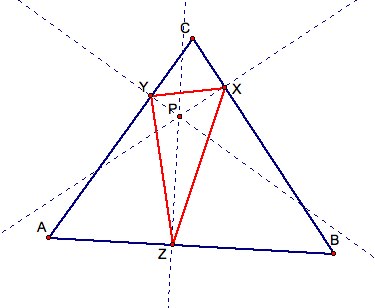
Given any triangle ABC and any point P in the plane, the triangle formed by the three points of intersection between the perpendiculars to the sides of ABC (extended if necessary) through P is the Pedal Triangle for Pedal Point P. Below is a diagram of pedal triangle XYZ.
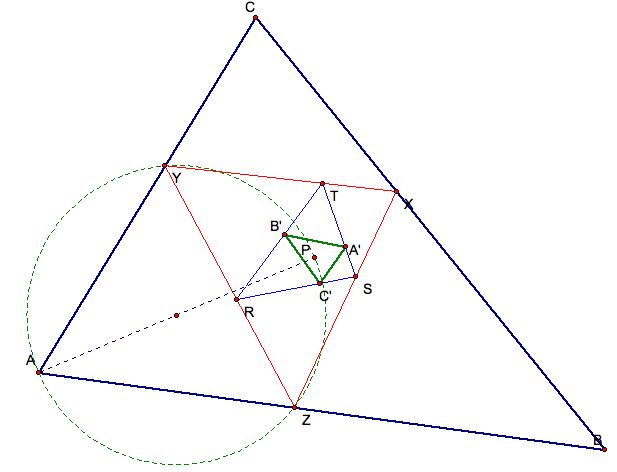
An interesting fact about pedal triangles is that the pedal triangle of the pedal triangle of the pedal triangle of a point is similar to the original triangle. In the diagram below, that means that triangle ABC is similar to triangle A'B'C'.
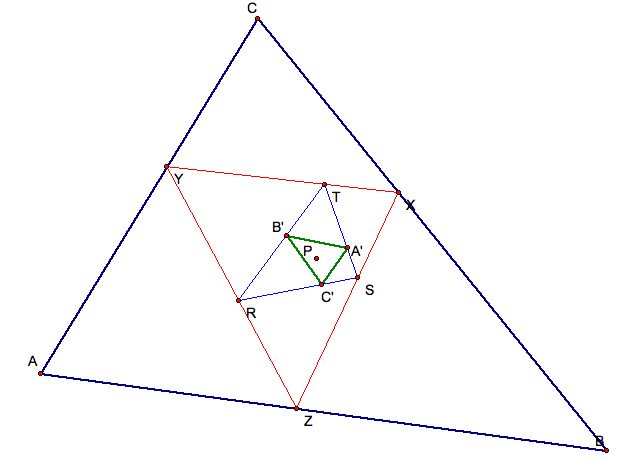
To prove this, I will use many circles and corresponding arc so show that angle B'A'C' is congruent to angle BAC. A similar argument can then be used to show that angles A'B'C' and A'C'B' are congrent to angles ABC and ACB respectively.
Construct the circle whose center is the midpoint of segment PT that passes through points P, T, A' and B'. Notice that angle PA'B' is congruent to angle PTB' since they subtend arc PB'. Next construct the circle whose center is the midpoint of segment PY that passes through points P, Y, T, and R. Angle PTR (which is the same as angle PTB') is congruent to angle PYR since they both subtend arc PR. Construct the circle whose center is the midpoint of segment AP that passes through the points A, Y, P, and Z. Notice that angle PYZ (which is the same as angle PYR) is congruent to angle PAZ since they subtend arc PZ. Using this chain of congruent angles, it is easy to see that angle PA'B' is congruent to angle PAZ.
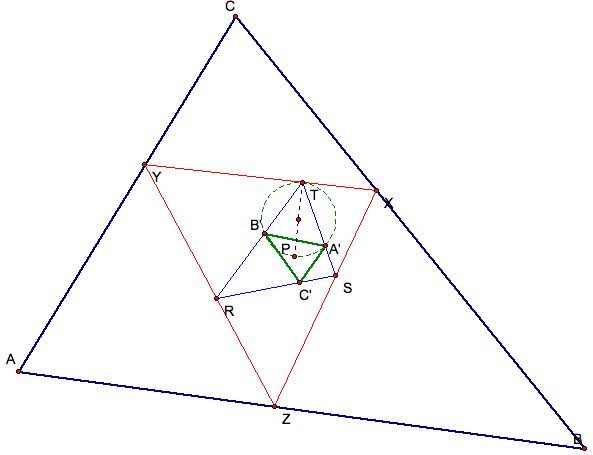
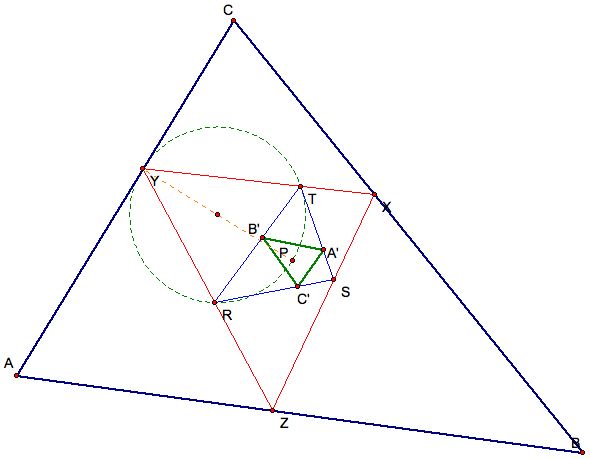
Notice that this only provides us with part of the angles that we need to show are congruent. But it is a straight forward process to use the same argument with other circles to complete the proof.
Now construct the circle whose center is the midpoint of segment PS that passes through points P, A', C', and S. Angles PA'C' and PSC' subtent arc PC' so they are congruent. Construct the circle whose center is the midpoint of segment PZ that passes through points P, R, Z, and S. Angles PSR (which is the same as angle PSC') and PZR subtent arc PR so they are congrent. Finally, going back to the circle shown in the last image above, angles PZY (which is the same as angle PZR) and PAY subtent arc PY so they are also congruent. Thus we can conclude that angles PA'C' and PAY are congruent.


Finally, since angle PA'B' + angle PA'C' = angle B'A'C' and angle PAZ + angle PAY = angle BAC, we can conclude that angle B'A'C' is congruent to angle BAC! Without going through the other two arguments, hopefully this is enough to prove to you that the triangles ABC and A'B'C' are similar since their angles are congruent.
There are also many interesting facts about pedal triangles when special points are used for the pedal point P. For example, if the pedal point is the circumcenter, then the pedal triangle is the medial triangle. That is to say, the vertices of the pedal triangle are the midpoints of the sides. If the pedal point is the orthocenter, then the pedal triangle is the orthic triangle. Hence, the vertices of the pedal triangle are the points of intersection for the altitudes. If the point is the incenter, then the pedal triangle is the intouch triangle. Therefore, the vertices of the pedal triangle are the points of tangency of the incircle.
To go a step further, you could investigate the pedal triangle for other special points, including points on the sides of the triangle, the vertices, the centroid, or the center of the nine-point circle. There are tons of fun activities with these pedal triangles and I hope that this introduction has sparked your curiosity!