
Assignment 7 Write-up

Given two circles and a given point on one of the circles, find a tangent circle to both circles that passes through the given point.
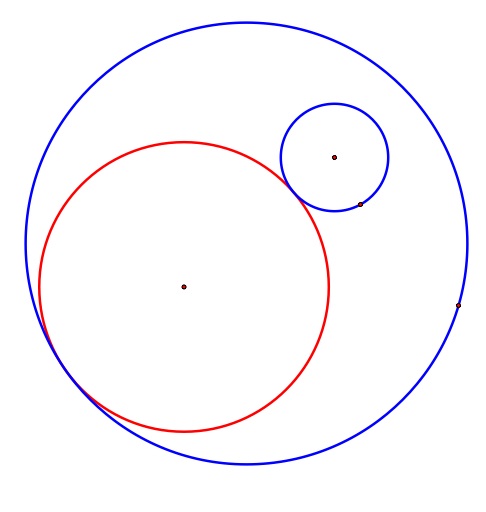 For
a script tool, click here.
For
a script tool, click here.
Notice that if you trace the center of the tangent circle while doing the animation, it creates an ellipse.
Here is another kind of tangent circle.
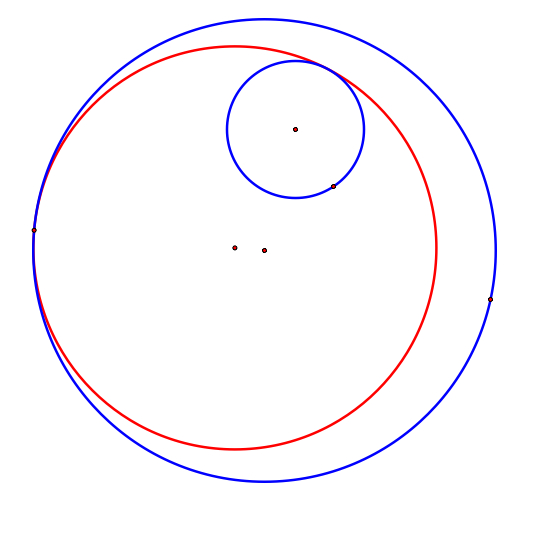
In this example the smaller circle is internal to the tangent circle, whereas in the first example, it was external to the tangent circle. Once again, if you trace the center of the tangent circle while doing the animation, an ellipse will be created that has the centers of the non-tangent circles as the loci.
For a script tool, click here.
What if the given point is on the smaller of the two circles?

For a script tool where the smaller circle is external to the tangent circle, click here.
For a script tool where the smaller circle is internal to the tangent circle, click here.
What if the two given circles intersect?
Use the first script and you will see that the tangent circle seems to "weave" in and out of the given circles. Use the second script and you will find that the tangent circle acts even crazier! Also, try tracing the center of the tangent circle while doing animation and you will see another conic section form--the hyperbola!
What if the two given circles are disjoint?
Using both scripts will once again show you that the tangent circle can range from quite large to quite small. Also, when traced, the center of the tangent circle creates a hyperbola with the two centers of the given circles as its foci.