Let triangle ABC be any triangle. Then if P is any point in the plane, then the triangle formed by constructing perpendiculars to the sides of ABC (extended if necessary) locate three points R, S, and T that are the intersections. Triangle RST is the Pedal Triangle for Pedal Point P.  Here is an example of a pedal triangle.

Here is a GSP script for the pedal triangle.

Now, let's do some explorations, shall we?

What if pedal point P is the centroid of triangle ABC?

When I first started playing with this sketch, I thought that the pedal triangle would always stay fully inside triangle ABC.  However, I began to notice that if triangle ABC possessed a very small angle and the opposite side of the angle possessed a small measurement, then part of the pedal triangle could be seen outside of the ABC triangle.

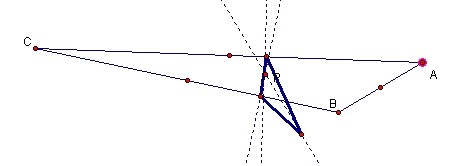Therefore, the only thing I can conclude about this sketch is that the pedal point P will always lie inside the triangle since the centroid always lies inside the triangle.  Also, whenever part of the pedal triangle "leaves" the original triangle, it always leaves by way of one of the three vertices.

What if pedal point P is the incenter of triangle ABC?

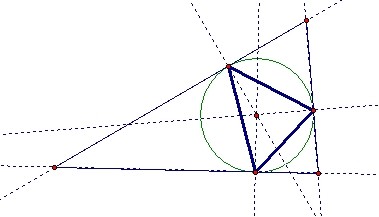One can see that each of the vertices of the pedal triangle is tangent to triangle ABC.  Now since the pedal triangle is constructed by connecting the intersections of the original triangle and its perpendiculars, and since the incenter is equidistant from each side of the triangle, we know that the radius of the inscribed circle equals the distance from P to each vertex of the pedal triangle.

What if pedal point P is the orthocenter of triangle ABC?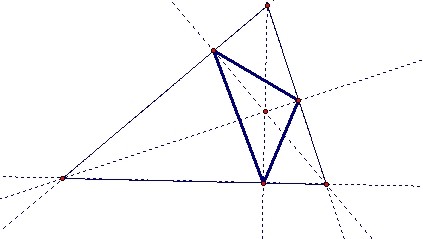Nothing much to see here; it looks like R, S, and T once again are tangent to triangle ABC.  Also, the pedal triangle is actually by definition an orthic triangle.  However, look at what happens when P/orthocenter lies outside the triangle. . .

Two of the vertices of the pedal triangle lie on the altitudes of ABC!  (That's about all I can come up with.)

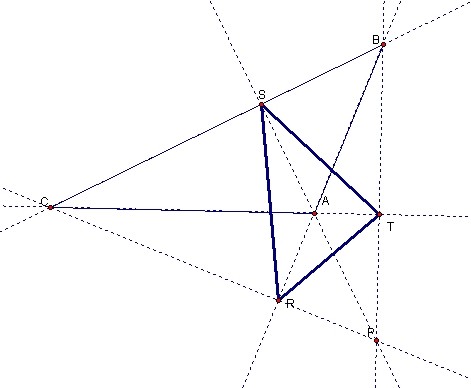
Last but not least, I want to look at what happens if point P lies on one of the sides of triangle ABC.

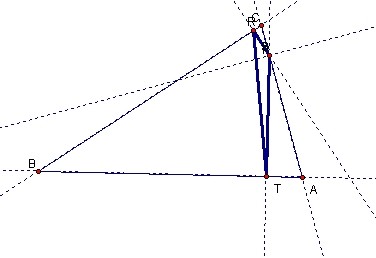As you can see, point P collapses onto one of the three vertices R, S, or T.  This is because P is the intersection of all three perpendiculars, and when P is put onto one of the sides, it must still be "on the line" perpendicular to that particular side and that point just happens to be R, S, or T.  Looking at this picture makes me wonder about something.  If P lies on a side, will angle P always be obtuse?  Well don't look at me for the answer!  Click here to investigate for yourself.

Return