

Polar Equations
Polar coordinates are another way of describing the location of a point in a two dimensional plane. Like the Cartesian plane, a system using polar coordinates has two variables, r and theta. r describes the distance of the point A from the origin, while theta describes the measure of the angle, in standard position, whose terminal side contains A.
Equations written in terms of r and theta can be used to describe curves in much the same way as equations that use the variables x and y. Below is a discussion of polar equation of two forms:
![]()
While the equations use polar variables, the values of a, b, and k still act in a transformational capacity.
a
In similar equations that utilize the Cartesian variables of x and y, any change in the value of a will cause the curve to dilate. With polar equations, this is still the case. Below are several similar curves, differing only by the value of a.
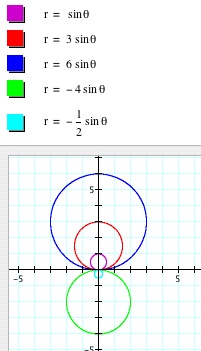
Notice that the graphs are symmetric about the angle theta = pi/2. This is where the value of sine equals 1.
b
On the Cartesian plane, the value of b would represent a vertical translation, affecting the y-coordinate. In a polar system, the effect is similar, though the value of b will cause a translation with respect to the r-coordinate. Refer to the graphs shown in the figure below:
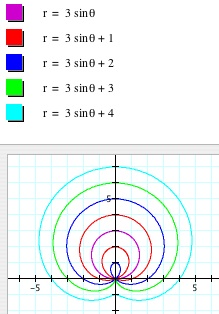
Notice that, when b is non-zero, the circular form is lost. Click Here to open a Graphing Calculator file that animates the changes in the curve as b changes. What do you observe? What values of b cause the second loop occur? Why does the second loop occur at these values?
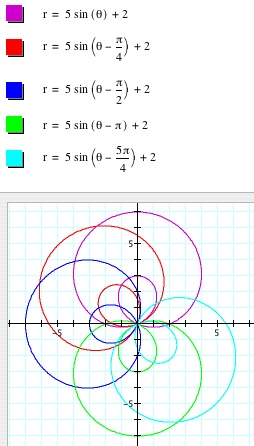
k
In any function uses x and y, the position of k indicates that is will cause a horizontal translation of the curve, affecting the x-coordinate. In this case, k will cause a translation of theta, that has a rotational effect. In the figure below, notice how the graphs change.
Graphs with Cosine
Graphs with the cosine function behave similarly to graphs with the sine function. Look at the figure below:
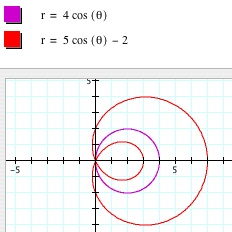
Notice that the graphs are symmetric about the angle theta = 0. This is where the value of cosine equals 1.
Challenge
Can you predict the equation for each of the graphs below? Using sine? Using cosine? How many different equations can you come up with?
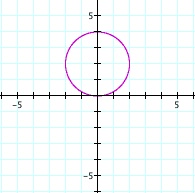
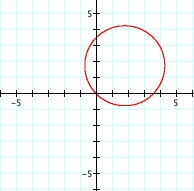
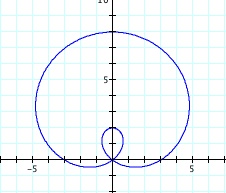
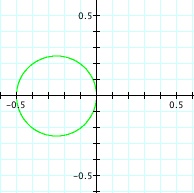
Click Here to view one solution for each graph (solutions are color coded).