
Investigating Pedal Triangles
by Margaret Trandel
This activity is designed for my current Geometry tutorial students - Meg, Anand, Will and Drew.
I would like you all to explore the questions below regarding pedal triangles. Please work through the GSP activity on your own before looking at the explanations! I think you will enjoy learning about pedal triangles through this GSP investigation.
Questions:
1. What is a Pedal Triangle?
2. Construct a Pedal Triangle, ABC and a point P in the plane of ABC. Construct a Pedal triangle RST using Point P.
3. What do you observe when Pedal Point P is the centroid of triangle ABC?
4. What do you observe when Pedal Point P is the incenter of triangle ABC?
5. What do you observe when Pedal Point P is the circumcenter of triangle ABC?. ..even if outside triangle ABC.
6. What if pedal point P is the center of the Nine Point circle for triangle ABC?
7. What if P is on a side of the triangle?
8. What if P is one of the vertices of triangle ABC?
9. What if P is the Orthocenter of the triangle?
STOP
Now that you have read the eight questions, please explore using GSP to answer the questions. Upon completion, please email your responses and GSP files to me. I hope you have had fun using GSP to explore pedal triangles!!
Ms. Trandel's Observations re: Pedal Triangles
Please NO PEEKING until you have completed your own investigation!
Questions:
1. What is a Pedal Triangle?
Let ABC be any triangle. If P is any point in the plane of triangle ABC, then the triangle formed by constructing perpendiculars (from P) to the sides of ABC (extended if necessary) locate three points R,S, and T. Triangle RST is called the Pedal Triangle for Pedal Point P.
2. Construct a Pedal Triangle, ABC and a point P in the plane of ABC. Construct a Pedal triangle RST using Point P.
This is one example. Your triangle probably looks different.
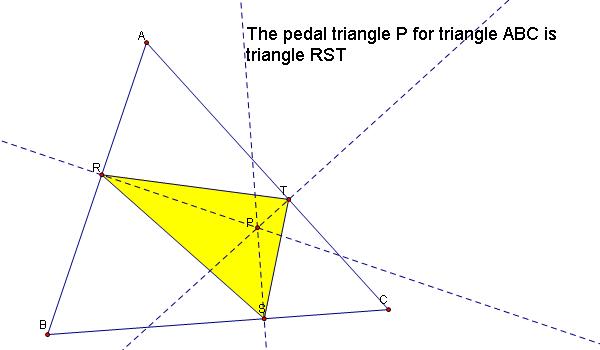
Open up this GSP file and move P around both inside and outside the triangle and observe what happens to the pedal triangle.
3. What do you observe when Pedal Point P is the centroid of triangle ABC?
Let's take a look. Remember, the centroid (G) is our Pedal Point and G is the point of concurrence of the medians in a Triangle. The centroid will always lie in the interior of the triangle, so the pedal triangle must also always lie in the interior of the original triangle.

4. What do you observe when Pedal Point P is the incenter of triangle ABC?
First be reminded that the incenter of a triangle is the point of concurrency of the angle bisectors, so the incenter is equidistant from the three sides (remember, equidistant means equal perpendicular distance) With the incenter remember we can create the incircle - the circle that is tangent to the triangle's three sides. The pedal triangle is constructed from the points where the incenter intersects the sides of the original triangle. The distance from the incenter to the sides is the same. P is therefore the circumcenter to the pedal triangle.
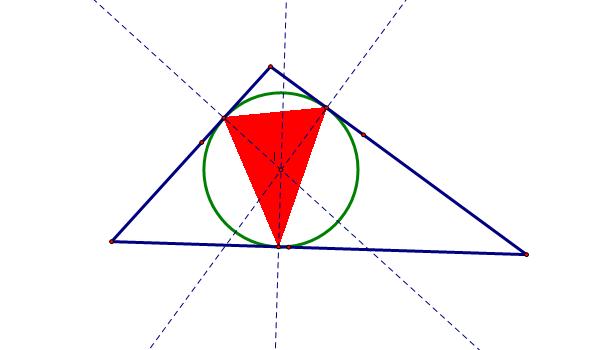
5. What do you observe when Pedal Point P is the circumcenter of triangle ABC? ...even if outside triangle ABC
Remember that the circumcenter is formed by finding where the perpendicular bisectors of each side concur. Since the perpendicular from the circumcenter to a side is at the midpoint of the side, the pedal triangle is the same as the medial triangle, because the medial triangle is also made up of the line segments connecting the midpoints of the sides.
6. What if pedal point P is the center of the Nine Point circle for triangle ABC?
When the pedal point P is the center of the nine point circle the Pedal Triangle can be in the interior of the original triangle, outside the triangular region or even a degenerate triangle.
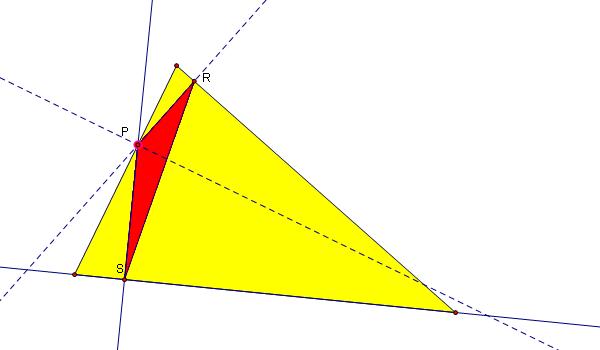
7. What if P is on a side of the triangle?
Below is one possibility, but to really observe what happens, open up this GSP File and slide P up and down the side of the triangle.
8. What if P is one of the vertices of triangle ABC?
You probably discovered from part 7 that the triangle does not exist if P is on a triangle vertex. If this is still confusing to you, open this GSP file and move P up and down the side of the triangle. To see that the pedal triangle doesn't exist when P is on a side of the triangle, open up GSP file 8 for another example. Again, slide P back and forth between the vertices and notice the triangle disappears when P becomes a vertex. The Pedal Triangle, when it exists is black and the main triangle is green.
9. What if P is the Orthocenter of the triangle?
If you know your vocabulary, they you know that the pedal triangle is the same as the orthic triangle. The orthic triangle consists of the intersection points of the altitudes of the original triangle. This is precisely the same set of points that create the pedal triangle.
Meg, Will, Anand, and Drew,
Thanks for participating in this extension Geometry Activity! I hope you enjoyed the experience!!You all are awesome and I wish you the best of luck on your final exams!!!See you in the New Year!!!Ms. Trandel