---

Assignment 9: Pedal Triangles

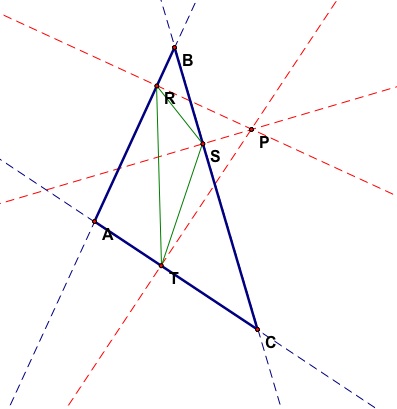
Now we will explore pedal triangles. Pedal triangles are dependent on a given triangle and a given point. Perpendicular lines are drawn to each side of the triangle such that they all pass through the given point. The pedal triangle is formed by connecting the intersections of the sides of the triangles with these perpendicular lines.

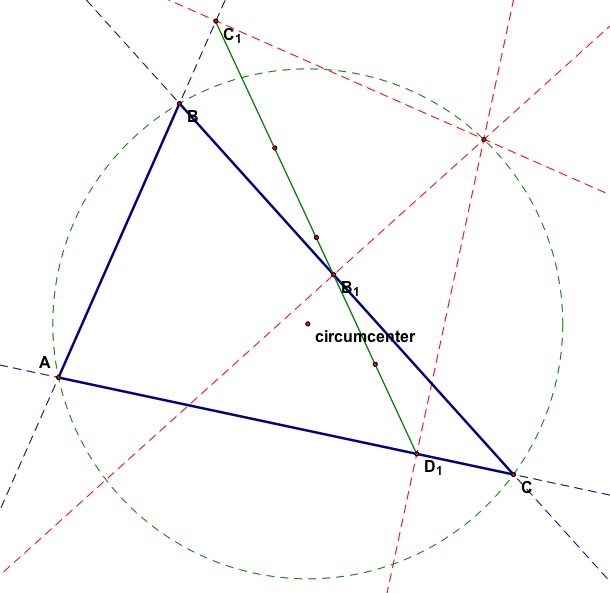
One interesting characteristic of pedal triangles is that the vertices become collinear whenever the given point, P, is on the circumcircle of the original triangle. When this happens, the triangle is said to be degenerate and the line segment is called the Simson Line.

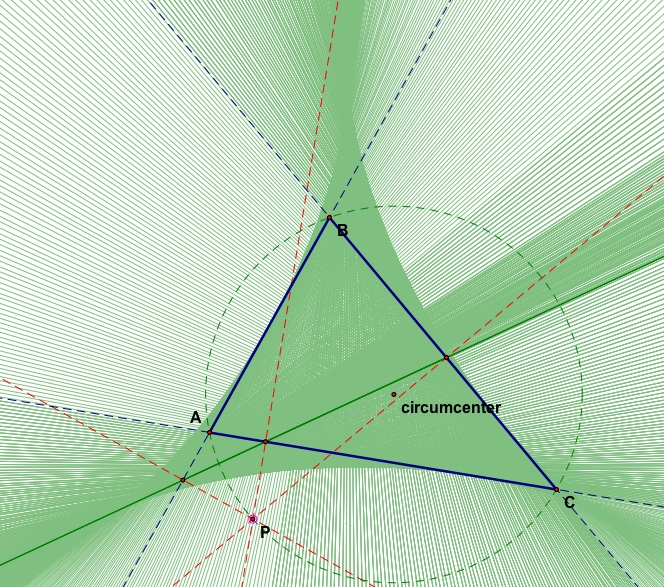
If we trace the lines containing the sides of the pedal triangle as point P moves around the circumcircle we get the following picture:

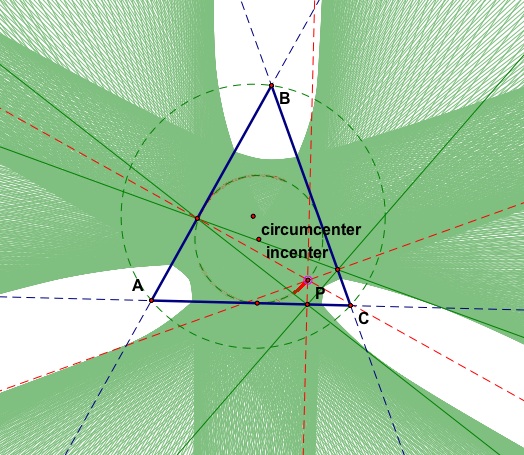
If we trace the lines again, but this time as P moves around the incircle we get the following picture:

When we trace the lines with P moving along the Euler line, three parabolic shapes seem to appear.

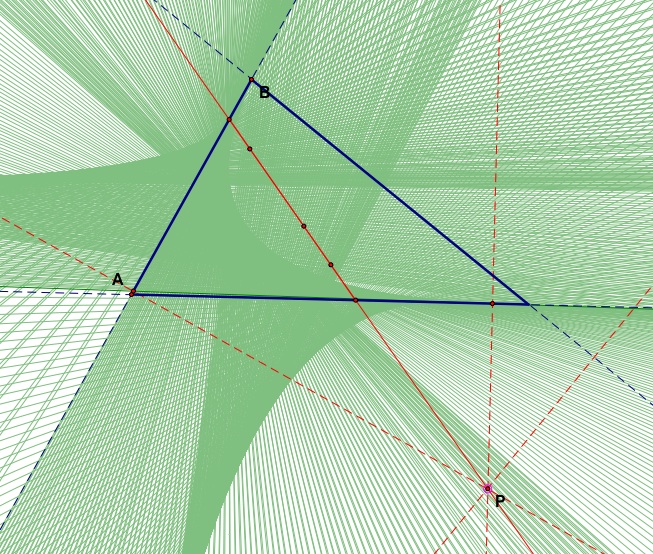
And lastly, if we just trace the midpoints of the sides of the pedal triangle as P moves along the circumcircle, the paths seems to form three interlacing ellipses:

Return to Katy's Homepage