

A young man was going through the attic of his grandfather's house and found a paper describing the location of a buried treasure on an particular Island. The note said that on the island one would find a gallows, an oak tree, and a pine tree. To locate the treasure one would begin at the gallows, walk to the pine tree, turn right 90 degrees and walk the same number of paces away from the pine tree. A spike was to be driven at that point. Then return to the gallows, walk to the oak tree and turn left 90 degrees and walk the same number of paces away from the oak tree. Drive a second spike in the ground. The midpoint of a string drawn between the two spikes would locate the treasure.
The young man and his friends mounted an expedition to the island but found the oak tree and the pine tree but no gallows. It had eliminated years ago without a trace. They returned home with the map and no treasure.
Show them where to look for the treasure.
Solution
To begin the problem first I made an exploration in GSP to discover the locus of the mid point. Surprinsinly I discovered that the locus does not change. Explore here. No matter where I choose the gallows, the mid point always is the same. So, the problem is not a problem anymore.
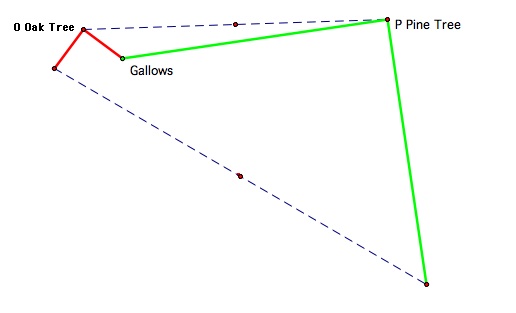
The young men only have to go the island again and choose an arbitrary point for the Gallows and then follow the rest of the instructions to find the treasure.
To prove this discovery, let us draw perpendiculars from each spike to the line passing through the trees. Consider the trapezoid SHKR.
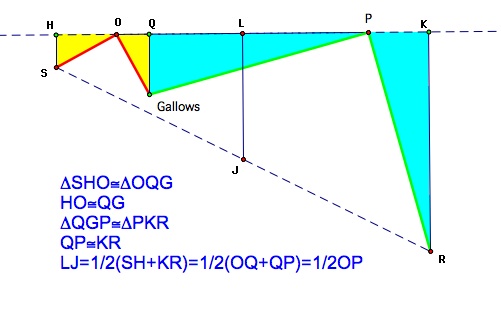
1. The sum of the lengths of SH and KR is equal to the length of OP.
2. The length of LJ is the arithmetic mean of the lengths of SH and KR.
3. As a consequence, LJ is one-half the length of OP.
4. So, the point J is fixed with respect
to OP and that location is independent of the location of the
gallows.