

1. Find as many solutions as possible for A, B, and C that satisfy both equations:
What observations can you make about your results? Again, we discussed some approaches to this one in class. Your task is to prepare a write-up that explores this task. (Not required, but you might want to consider how to explore this one with a spreadsheet as well as with graphing tools.).
2. Create another set of equations that also
yield a useful exploration.
Before any solution be due let us analyze some details.
If we consider ABC=4 neither A, B nor C could be 0
If A, B and C are negative values the equation does not have values that satisfies it.
If only one number is negative, there is not solution for the system.
Two of them could be negative if the third one is positive.
We know that a system of equations with two equations and three variables is an imbalance system and it does not have a unique solution. As a consequence, we should hope infinity solutions.
3A + 2B - C = 3 is the equation of a plane in the space and ABC=4 is a conic in the space, so the possible set of solutions is the set of points where the plane meets with the conic.
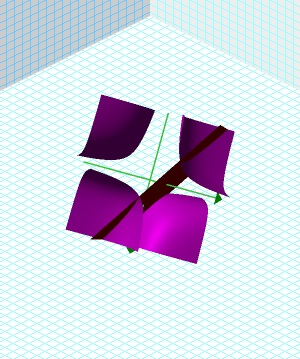
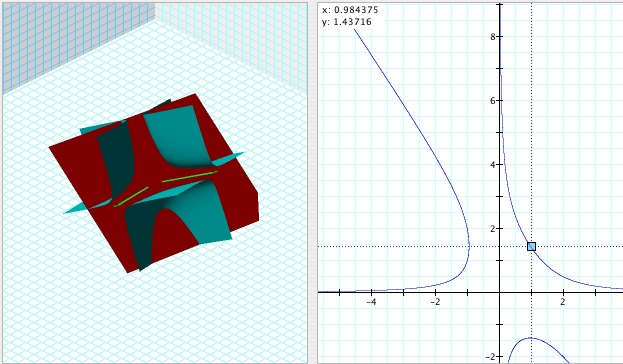
Then we can find any values for A, B, C
For example let us assume that A=1, using our equation AB(3A+2B-3)= 4 we can find that B=1.4 and then we go back to ABC=4 to find C=2.9. Next chart shows some solution for our system, you can try others

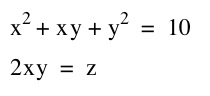
What observations can you make about your results?