
Tangent Circles
by
Chad Crumley
This
investigation begins with the following problem.
Given two circles
and a point on one of the circles.
Construct a circle tangent to the two circles with one point of tangency
being the designated point.
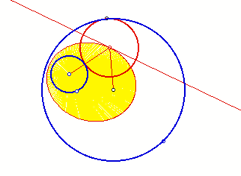
But, what are tangent circles?
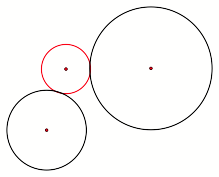
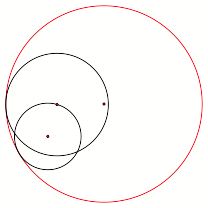
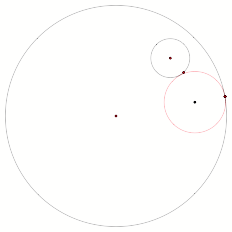
For any two given circles, a tangent circle is a
circle tangent to both given circles.
Below are some possibilities (given circles in black, tangent circle in
red):
Many explorations can be done with tangent
circles. But I will focus on the
hyperbola and the ellipse. Here is a trace of a hyperbola by moving point
A. Click
here for the hyperbola exploration.
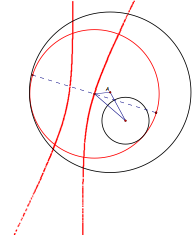
A hyperbola is the set of all points in the plane the
differences of whose distances from two fixed points is some constant.
The ellipse is formed below (the black trace). Click here for
the ellipse exploration.
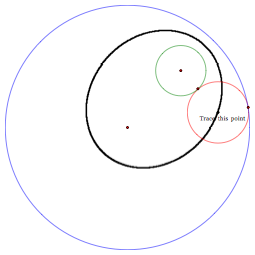
An ellipse is the set of all points in the plane such
that the sum of the distances from to two fixed points is some constant. The two fixed points are called the
foci (plural for focus).