Polar Equations

Karyn Carson

Investigate

Note:

• When a and b are equal, and k is an integer, this is one textbook version of the " n-leaf rose."
• Compare with

for various k. What if .. . cos( ) is replaced with sin( )?

If k = 1, then we getthis graph:

Now, let’s change thevalue of k to 2, 3,4:

It would seem that kdetermines the number of petals that the flower has. Another thing that’s interesting is that they all cross thex-axis at (2,0). Also, there seemsto be a limit of 2 and -2 for the graphs that have more than one petal.

Now, let’s keep kconstant and vary a and b. First,let’s make a = 1 and b=2:

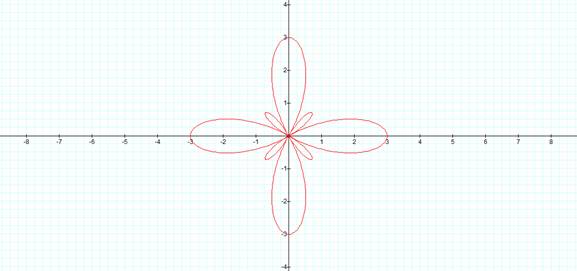
We get a doublefour-petaled flower. Now the limithas gone to 3 and -3. When wechange b to 3,

It’s still a doublefour-petaled flower, but the limit has grown to 4 and -4. It appears as though the limit isdetermined by the sum of a and b and its inverse.

Now let’s keep the valuesof b and k constant and vary the value of a.

r=1+cos((4*theta))and r=2+cos((4*theta))

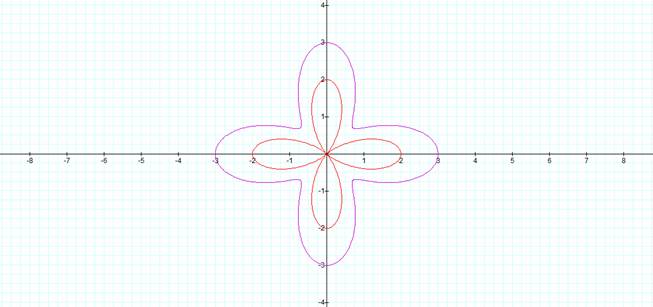
The graph no longer intersectsat the origin and I think that the graph will continue to enlarge, going towarda circle.

If we change a to 3, 4, 5 and 6:

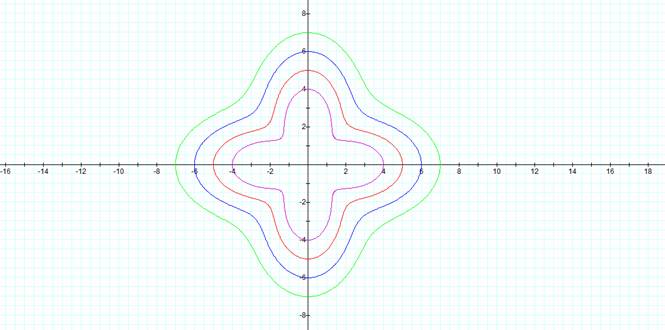
Let’s make a=10…

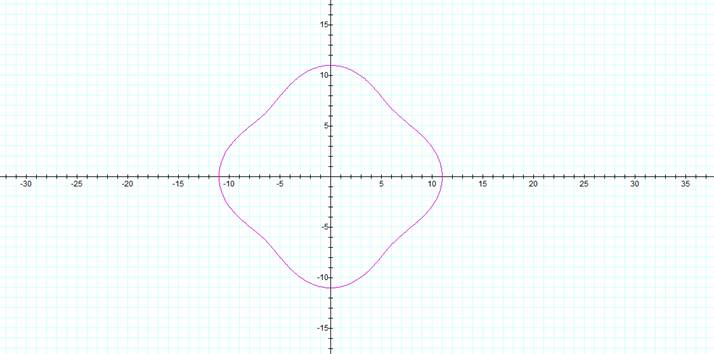
Well, I’m not sure that it will ever be a circle, but what I do notice is that my conjecture about the sum of a and b and its inverse being the limits of the graph seems to be true.

How would the graph bedifferent if we took away a?

Let k = 1, 2, and 3 in the equation …

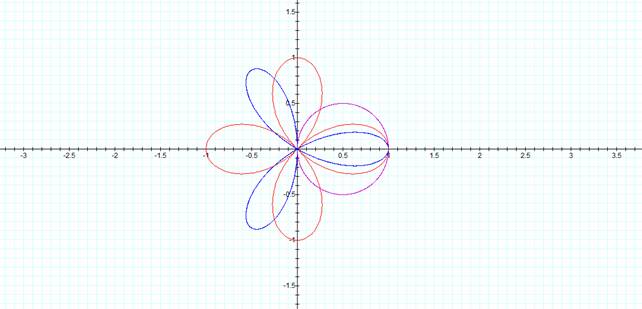
K still determines thenumber of petals, but it appears as if k is odd, k=the number of petals and ifit’s even, 2k=the number of petals. Let’s continue the investigation by changing k to 4 and 5:

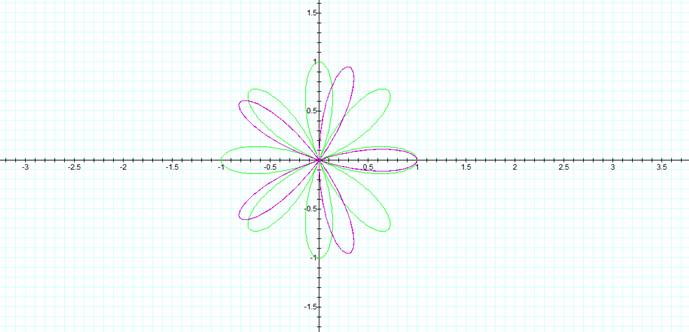
Yes, if k=4, then thenumber of petals that are produced is 8 and if k=5, the number of petals is 5.

If we change cos to sin(using the original equation ), andvary k from 1 to 3, then the graph turns and is no longer focused on thex-axis, but seems to be centered on the y-axis.

Back toKaryn’s Homepage