Instructional Unit on Concepts of Measurement

by

Gayle Gilbert and Tiffany Keys

DAY 1:

 

Goal:     Students will derive formulas for area using GSP sketches.

 

Open discussion and lecture:

á      Discuss area.

á      Students will begin a series of activities using GSP to find formulas for the areas of various figures.

 

Sample Activity:

á      Students will be given a variety of shapes to look at on the SMART Board and will be required to find the area of each of the figure shown.

á      Students should be able to find the areas for all the figures given the unit of area.

á      Students will also be given specific areas and asked to create a rectangular, triangular, and a figure of their choice on GSP.

á      The link to the GSP Area Discovery Activity.

 

 

DAY 2:

 

Goal:     Students will extend their knowledge of area.

 

Open discussion and lecture:

á      Students will be introduced to formal definitions and formulas of area rectangles, triangles, parallelograms, and trapezoids.

á      Students will also introduced to the altitude of a triangle.

á      The formula for the area of a triangle will be proved.

á      Allow students to make conjectures for the formulas for the areas of parallelogram and trapezoids.

 

Proof:

Given a triangle with altitude h and base a, construct a rectangle inscribing the triangle with length a and width h. 

This rectangle is made up of two smaller rectangles of areas  and  .  The legs of the triangle, b and c, bisect the areas of the rectangles, so the area of these triangles is  and  .  Thus, the total area of the given triangle is the sum of these two smaller triangles: .  Thus, the area of a triangle is given by the formula area is equal to half the base times the height. 

After demonstrating this proof, ask students what would happen if the triangle was obtuse instead of acute. 

 

DAY 3:

 

Goal:     Students will review Trig ratios and use them to solve problems.

 

Open discussion and lecture:

á 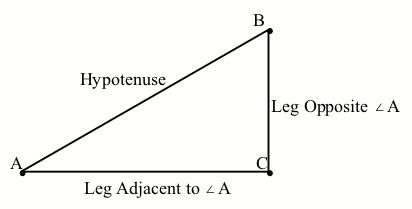     Today we are going to work with right triangles.

á      Review trigonometric ratios (SOH CAH TOA). 

 

 

o    

o    

o        

 

á      Students will also review the cotangent, secant, and cosecant ratios.

o    

o    

o    

 

á      Discuss properties of the trigonometric ratios. 

á      Individually, students will find the altitude of some given triangles trigonometric ratios.  Some examples are given:

 

 

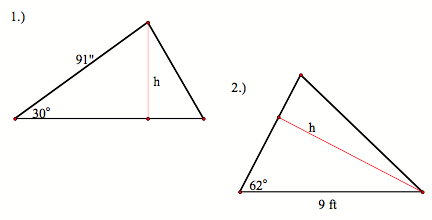
á      Students will work on application problems in groups.  Students should display their work on a poster and present their results to the class.

o     A group of students were using shadows to find the heights of trees near their school.  They used the diagram shown here to represent the general situation.  Let  represent the angle of elevation of the sun and represent the length of the treeÕs shadow. 

¤      In one case, the students found  and .  What is the height of the tree?

¤      Later that day, with a different tree, they got  and .  What is the height of that tree?

¤      Develop a general expression for the height of the tree in terms of  and .  Make sure to draw a diagram to help explain.

o     A sailboat is in trouble, and the people on board are considering trying to swim to shore.  A lookout station on shore is able to tell them they are 2.3 miles from the station and the line from the station to the boat forms an angle of  with the shoreline.  Assume the shoreline is straight.

¤      If the people are capable of swimming 1.5 miles, will they be able to make it to shore or should they call for help?  Explain.

¤      The lookout station officer would like to be able to tell people in such situations their actual distance from shore.  Find a general formula the officer can use to find this distance.  Your formula should express this distance in terms of the distance from the station to the boat and the angle between the line to the boat and the shoreline.

 

DAY 4:

 

Goal:     Students will derive and prove the Pythagorean Theorem given a few GSP sketches.

 

Open discussion and lecture:

á      Students will be introduced to the Pythagorean Theorem.

o     Given a right triangle, the area of the square on its hypotenuse is equal to the sum of the areas of the squares on its other sides.

o     Students will be asked to derive a formula from the below GSP sketch.

 

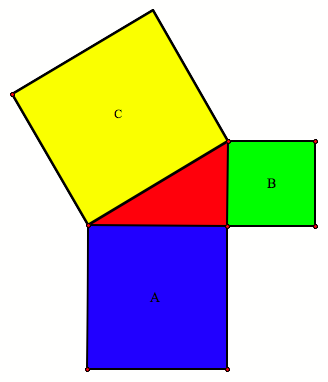
á      For further clarification, the students will be shown two additional GSP sketches that provide proof of the Pythagorean Theorem.

o     Students will be asked to explain why these sketches prove the theorem.

o     Students will also be asked how the below sketches are either similar or different from the above sketch.

 

 

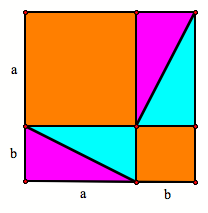
DAY 5:

 

Goal:     Students will apply the Pythagorean Theorem to real world applications.

 

Class Activity:

á      Students will be put into five groups of four to work on the following real world problems.

 

1.    An 8-foot ladder is leaning against a wall, as shown in this diagram.  The bottom of the ladder is 2 feet from the wall.  How high up from the wall does the ladder reach?

 

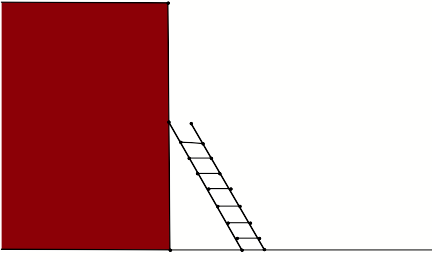
2.   Mary wants to check that her doorframe makes right angles at the corners.  The door is 8.5 feet high and 5 feet wide.  How long should the diagonal of the door be if the corners are right angles?

 

3.    Bobby was doing one of her favorite trick billiard shots.  Her shot started at one corner of the table, hit the exact center of the back cushion, and rebounded into the other corner.  Draw an accurate diagram of BobbyÕs shots on the pool table.  How far did her billiard ball travel if the table is 8'x5'?

 

4.  Chris and David decide to race from one corner of an open field to the other.  The field is rectangular in shape, 60 meters long and 80 meters wide.  Since Chris is older and faster, he's going to run along the outside of the field, and David will take the diagonal route.  If David can run at a rate of 5 meters per second, how fast will Chris have to run to get there at the same time as David?

 

5.  Leslie, the landscape architect, has made a design for a flowerbed for a very important client.  The flowerbed will be in the shape of a triangle, with sides of lengths 13, 14, and 15 feet.  Leslie needs to know the area of the flowerbed so she can order the correct amount of fertilizer.  Suppose you are Leslie's assistant.  In order to find the area, you need to find the length of the altitude.  

(a.) Find the length of the altitude.  

(b.) Calculate the area for Leslie.

 

Open discussion and lecture:

á      Upon completion of the problems, students will be asked to present the solution to one of the problems and also address any questions their classmates have for that particular problem. They will also ask their classmates if they came upon the correct answer using a different strategy.

 

DAY 6:

 

Goal:     Students will work on maximizing area.

 

Class Activity:

á      Students will investigate the following scenario in groups:

o     Farmer Greene is building a corral to keep his horses in.  He decides that he can afford to buy 300 feet of fencing.  For aesthetic reasons, he decides that the corral should be built in the shape of a rectangle.  He wants to build the corral such that there is as much space as possible inside it for the horses to move around.  Students should find a formula to find the area of the corral using w as the width.

á      After students work on the problem, a graphical representation should be shown.

 

 

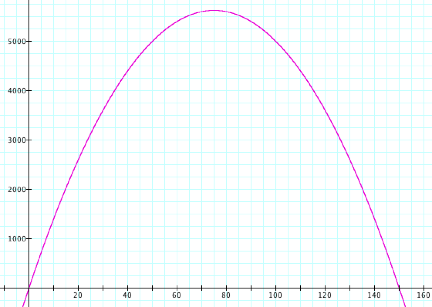
á      Ask students what triangle with perimeter of 300 feet has the biggest area. 

á      Now, have a discussion about what shape the corral should be if Farmer GreeneÕs only concern is that the horses have the most area with a perimeter of 300.  After discussion, have students fill out a chart for the area with various regular polygons.  Finally, students should develop a formula for the area of the corral in terms of n, where n is the number of sides of a polygon.

 

DAY 7:

 

Goal:     Students will construct a box with the biggest volume possible.

 

Class Activity

á      As students come in they will be put into groups of pairs and given scissors, tape and construction paper.

á      We explain that a box is a container with four rectangular sides including a rectangular bottom and an open top.

á      Students will use cereal to judge how much their box can hold. In order to measure the amount of cereal in their boxes, the students will use a separate container in which they will pour the cereal from their boxes into it and mark the level.

 

 

Class discussion:

á      Have students line up finished boxes from.

á      Have students to compare boxes and discuss why oneÕs box can hold more or less than anotherÕs.

á      Does the shape of a container determine how much it can hold?

 

Homework:

Look around your home for containers of different shapes and sizes. Take note of the units of measurement that are used to describe the contents inside these containers.

 

DAY 8:

 

Goal:     Students will compare and contrast different shapesÕ volumes.

 

Open discussion and lecture:

á      Compile a list of the different types of container that the students observed in their homes.

á      Discuss the different units of measurement in the different containers and how the units matched the product inside the container.

á      Ask what criteria students think are important when companies are designing containers.

á      Students will be introduced to the formal definition and formulas of volume and surface area.

á      Students will then explore the volumes of the different containers they observed at home, and also of other shapes.

á      After the class activity, students will discuss what they think is the best shape for maximizing volume.

 

Class Activity:

á      Students will work toward solving the following problems.

o     Farmer Greene has a drinking trough for his animals.  The trough is in the shape of a triangular prism.  The triangle that forms the base of this prism is a right triangle whose legs are 1 foot long.  The trough is 5 feet long.  How much water will the trough hold when it is full?

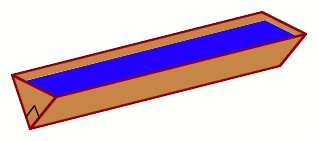
o     The farmer wants to build a barn.  The barn will be in the form of a prism with a base thatÕs a regular polygon.  Of course, the sides will be vertical.  He wants the base of the prism to have a perimeter of 300 feet.  He is trying to decide if he should make the barn floor in the shape of a regular octagon, decagon, or dodecagon.  He wants to paint the outside of the barn, so he decides to look for the shape that gives the barn the least lateral surface area.  The barnÕs walls will be 10 feet tall.  What would you suggest to Farmer Greene?

 

 

DAY 9:

 

Goal:     Students will clarify their knowledge of the material that has been covered.

 

Open discussion and lecture:

á      Students will take part in an oral and written review of the different types of measurement concepts that will be highlighted in their future assessment.

á      As students are completing the review of measurement concepts, they will have to opportunity to ask their peers and the teacher questions in order to clarify any misconceptions or misunderstandings they have had.

 

DAY 10:

 

Goal:     Students will display their knowledge of the material that has been covered.

 

Assessment

á      Students will be given an assessment that includes different measurement concepts. In addition to multiple choice and fill-in-the-blank questions, students will also be asked to complete words problems applying these concepts to the real world applications.

 

 

References:

 

Fendel, D., Resek, D., Alper, L., & Fraser, S. (1998). Do Bees Build It Best? Interactive Mathematics Program. Berkeley, CA: Key Curriculum Press.

 

 

 

Return