Eric Gold

Assignment 1, Part 3:

Tangential Linear Equations

Find two linear functions f(x) and g(x) such that their product, h(x) = f(x)*g(x), is tangent to each of f(x) and g(x) at two distinct points.

Consider the simplest case. For the both lines to be tangential to the curve, they must have negative reciprocal slopes. This allows the vertex of the parabola and the intersection of the lines to be collinear. That is to say, the vertical line through the intersection of the 2 lines is the axis of symmetry of the parabola. The simplest such lines are f(x) = x and g(x) = -x:

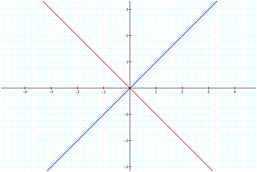
Graph A

Taking the product gives me h(x) = -x^2,, in Graph B.

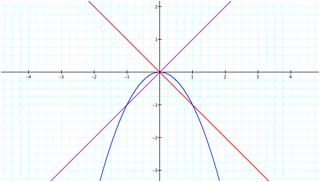
Graph B

Note that either the linear functions or the parabola need to be shifted in order for f and g to be tangential to h. It makes sense to try and raise the linear graphs above the parabola by changing the y-intercepts, first raising them by 1 as follows:

f(x) = x + 1

g(x) = -x + 1

h(x) = (x + 1)(-x + 1)

By observation of Graph C, this is too much, so consider splitting the difference between the 2 intercepts, lowering them by ½:

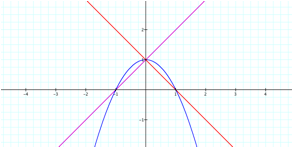
Graph C

This results in Graph D.

f(x) = x + ½

g(x) = -x + ½

h(x) = (x + ½)(-x + ½)

Note that the curve h appears to be tangent to the functions f and g. The vertical shifts in the graphs have maneuvered the lines in such as way that have left us with the linear functions apparently tangent to the graph of the parabola that is the product of them at the roots of the parabola as seen in Graph D, immediately below.

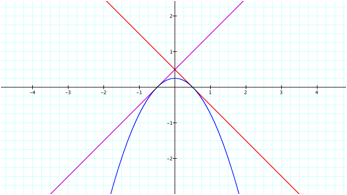
Graph D

This can be verified by increasing the scale and looking more closely at the closed interval for x [ -1, 1]:

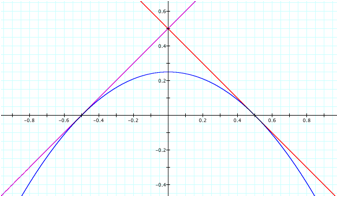
Graph E

Observe:

By manipulating the product of a sum and a difference, we were able to manipulate the linear functions up and down the y-axis until we reached the desired condition, specifically that the linear functions both be tangential to the function that is their product.

We could continue to work with the function and manipulate it in such ways that we shift the graph horizontally, that we stretch or compress the graph, i.e. alter how fast the parabola changes, or any combination of all three features of the graph.

Return to problem page