Carisa
Lindsay
EMAT
6690
Rational
Functions
Rational
functions are defined as the quotient of two polynomials. With a basic understanding of polynomials,
one can see there are many possible explorations regarding various degrees and
quotients.
First,
let’s consider the quotient of two linear functions.
I
will define 
and
.
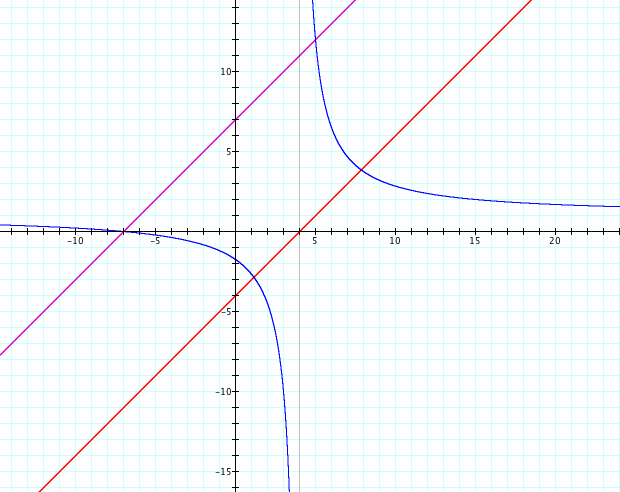
The
most interesting portion of this graph is the presence of a vertical asymptote
at x =4. The asymptote exists here because the graph
is undefined when x =4 and the graph
will bend away from this number which makes the denominator zero. Furthermore, there is a horizontal asymptote
at y =1 because the degree of the
numerator is the same as the degree of the denominator.
It
is also interesting to note that the x-intercept
of the quotient of f and g, from here on referred to as h(x), is x=-7. This is precisely the same as the x-intercept for f. Perhaps we should verify
this with another quotient of linear functions to see whether it holds
true. It should, seeing that the values
which make y =0 are the same for f and h.
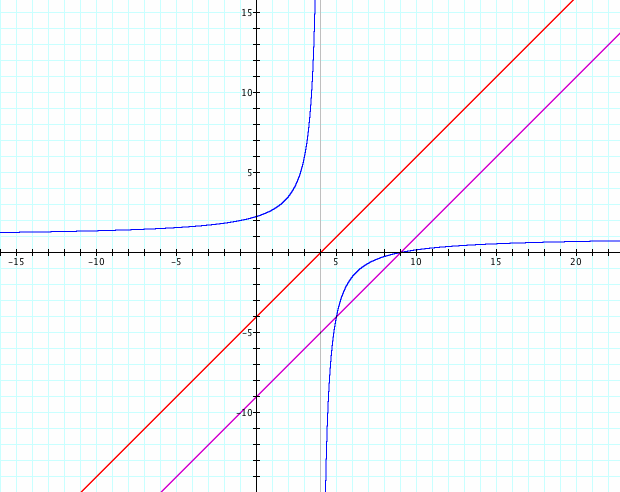
As
you can see, it holds true. Through
basic algebraic manipulation, we can see that it will always be true.
Now,
let’s consider the quotient of two quadratic functions. Suppose 
and 
. The
quotient of these two functions is interesting because there is a vertical
asymptote, a hole, and a horizontal asymptote.
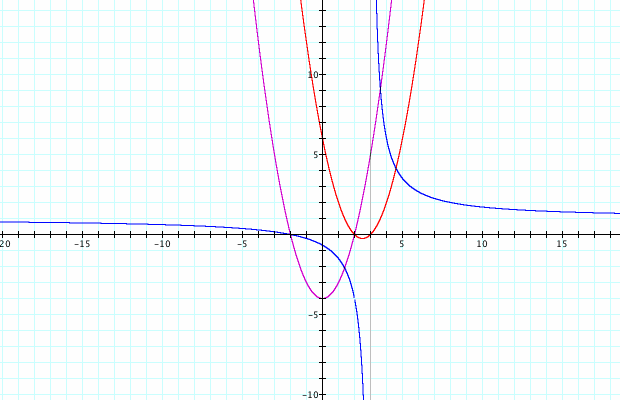
Through
factoring and other algebraic manipulation, we can see there is a vertical
asymptote at x =3 and a hole at x =2 (the coordinate (2,-4) since it is
very difficult to see!). It may not be
as obvious that there is also a horizontal asymptote at y =1. In order to find x and y-intercepts, we simply need to substitute x =0 and y =0. It turns out that the x-intercept is the same for f(x)
and h(x) like it has been in every
example thus far. This is because y =0 on the x-axis and the only way for this to be true is if the numerator (or
f(x)) is equal to zero. If the denominator (or g(x)) is equal to zero, then we will have an undefined function at
this location (also known as the asymptote).
Now
we can again consider the quotient of two quadratic functions. Let 
and
.
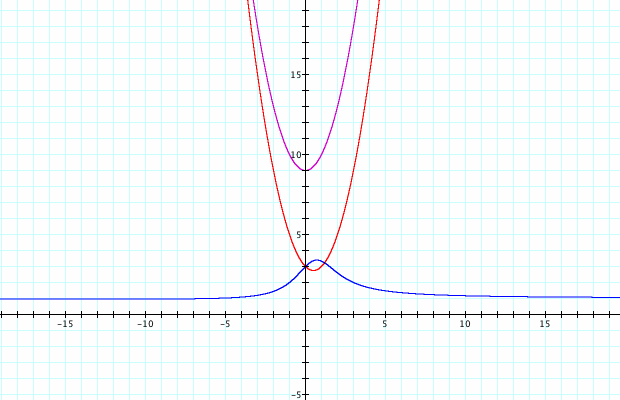
These graphs are particularly interesting because f(x) and g(x) only have
complex roots. Their quotient does not
behave in a similar way as the examples above for this reason. This rational function lacks true asymptotes
and holes because there is not a place where the graph is undefined in the real
number system. Instead we see an
interesting result of no vertical asymptotes.
However, we do see a horizontal asymptote at y =1. It is also interesting
to note that the y-intercept is the
same for g(x) and h(x) (y =3).
What
about the quotient of two cubic functions?
According
to the pattern we have seen so far, we can predict a horizontal asymptote at y =1 (since we know the degrees of the
numerator and denominator will be the same).
We can also predict that f(x)
and h(x) will have the same x-intercepts. The presence of a hole or a vertical
asymptote is dependent on the numeric values of each function. Let’s consider 
and
. Let
their quotient be h(x).
Our
predictions are correct. Firstly, we
have a horizontal asymptote at y =1
(again, because the degrees are the same, we take the ratio of the leading
coefficients to find the horizontal asymptote).
We also see the x-intercepts
are the same for f(x) and h(x).
More specifically, we see vertical asymptotes at x =1 and x =-6. In addition, we should also see a hole at x =0.
What
about the quotient of two quartic functions?
More
than likely, we will see the same pattern with quartic
polynomials as with cubics, quadratics, and
linear. We should have the same
horizontal asymptote arising from the ratio of the leading coefficients since
the degrees will be the same, and f(x)
and h(x) should have the same
x-intercepts. Let’s verify this is true
with an example.
Let
and
.
Again, allow h(x) to represent
the quotient of these two polynomials.
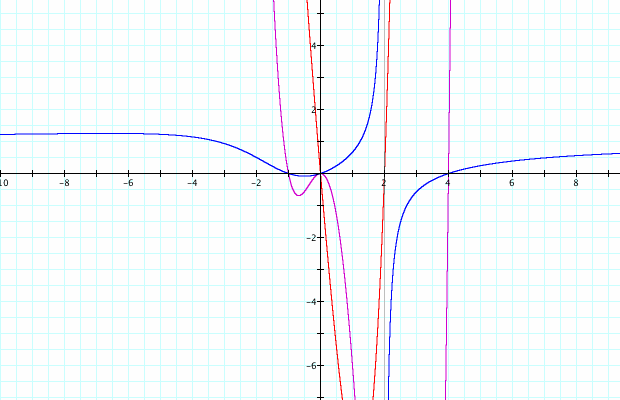
This
is an interesting graph because the horizontal asymptote is not as clear in
this example. Much like the other
rational functions, this one has a vertical asymptote at x =2 and contains a hole at x
=0. Furthermore, f(x) and h(x) have the
same x-intercepts (x =-1 and x =4).
So
far, we have only investigated the quotient of functions of the same
degree. What happens if the degree of
the function in the numerator is higher than the degree of the
denominator?
Let’s
suppose 
and
.
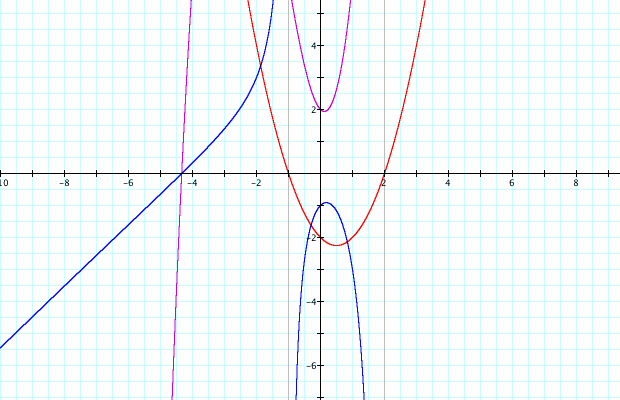
Again,
we see that f(x) and h(x) have the same x-intercepts and the vertical asymptotes are determined by g(x) =0.
The horizontal asymptote is missing from this picture- which is exactly
what one should expect if the numerator degree is greater than the denominator’s
degree.
Perhaps
we should also consider the quotient of polynomials in which the degree of the
numerator is less than the degree of the denominator.
Suppose
we take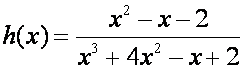 
this time.
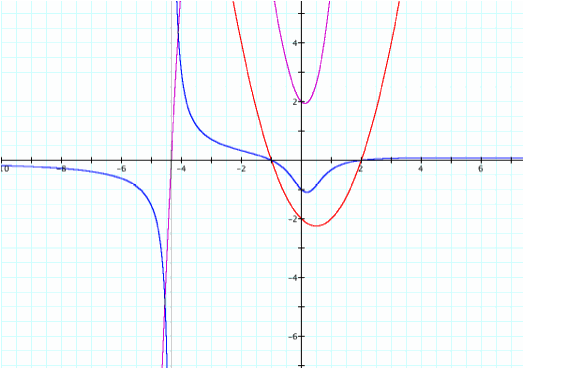
We
see a horizontal asymptote at y =0,
which is what one should expect when the degree of the denominator is larger
than the degree of the numerator. We
again see that h(x) and g(x) have the same x-intercepts.
Not
all asymptotes are vertical or horizontal lines. The majority of asymptotes students encounter
are, but we also have “slant asymptotes”.
A slant asymptote is exactly like what it sounds- an asymptote which is
slightly tilted rather than a vertical or horizontal line. Slant asymptotes are essentially the
resultant polynomial or the quotient of the two polynomials. Furthermore, slant asymptotes only result if
the degree in the numerator is greater than the degree of the denominator since
there cannot be a horizontal asymptote.
For
instance, let’s consider the rational function 
.
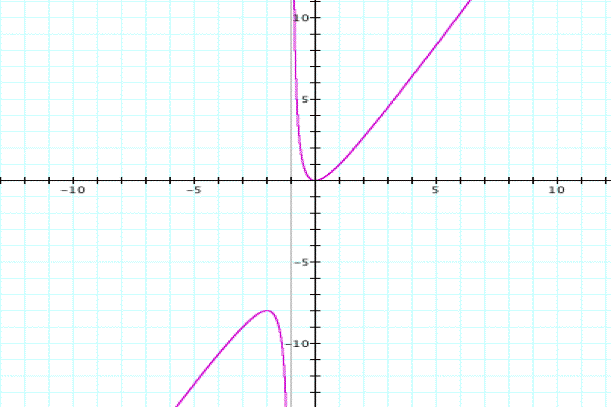
As
you can see in the picture, it appears that there is some sort of asymptote
guiding the path of this graph. To find
this slant asymptote, we must use long division (or synthetic division if
possible).
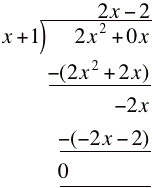
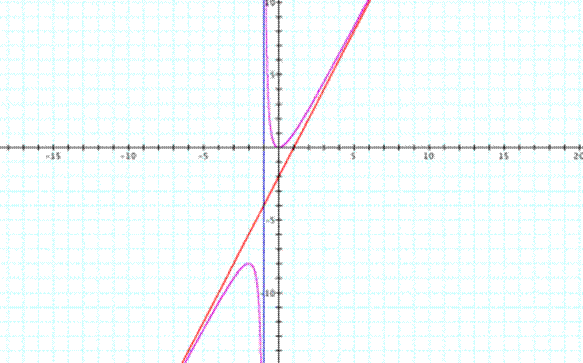
So,
our quotient is the equation of the slant asymptote: 
.