Carisa Lindsay

Final Project

Ceva’s Theorem

For any triangle ABC, and any point P within the boundaries of ABC, we can see rather informally that the ratio of the products of segments created by extending the lines is one.

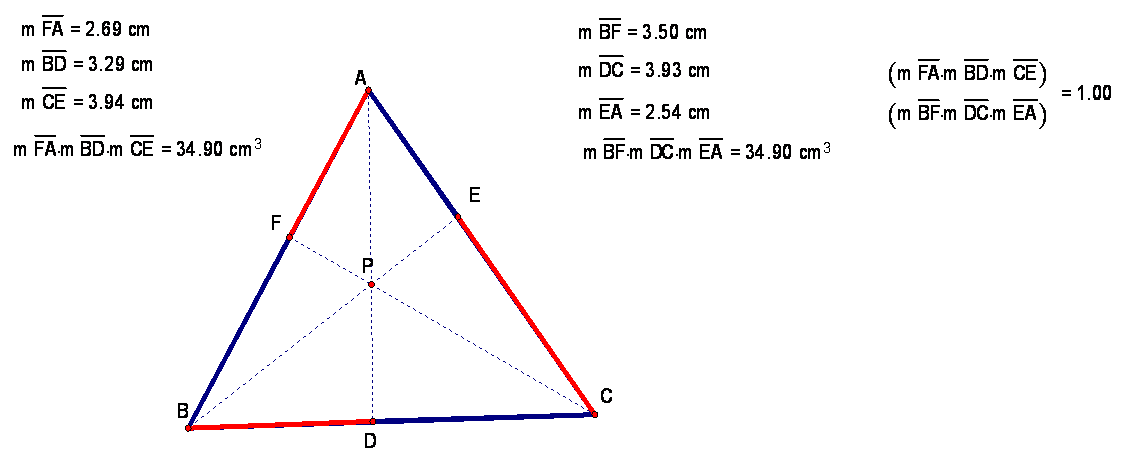
Regardless of where point P is within ABC, the ratio of the products of segment lengths remains a value of one.

Suppose we didn’t have access to numeric values and need to see that Ceva’s Theorem is true. We can prove Ceva’s Theorem through the use of similar triangles.

First, we can see that AD, BE, and CF are concurrent at point P.

To construct similar triangles, we first need to construct a transversal (a parallel line) through point A.

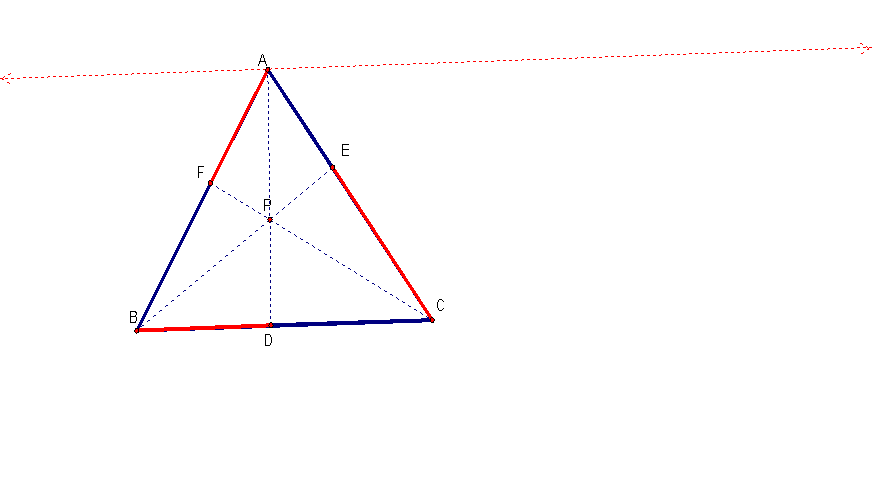
Then we can construct a similar triangle by extending BE until it intersects with the parallel line. From this construction, we can build similar triangles.

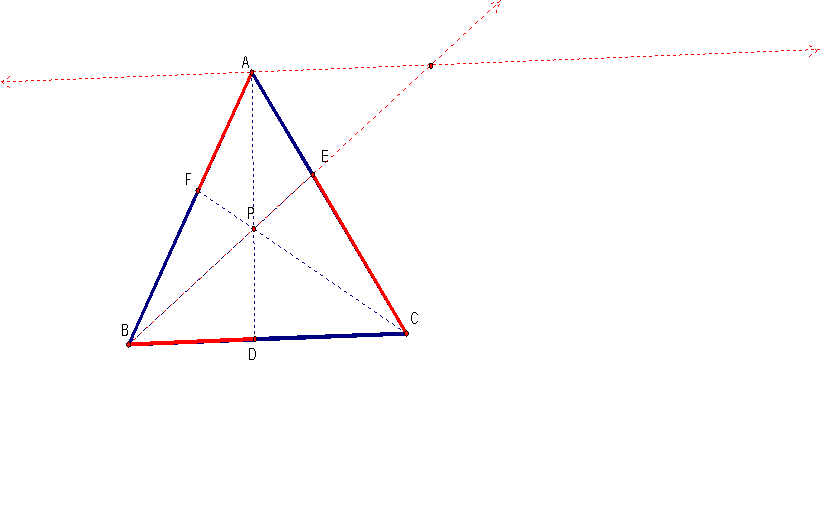
Triangle AEG is a similar triangle to triangle BCE because BG acts as a transversal and we can use alternate and corresponding angles to show that they are similar.

For similar reasons, triangle AFH is similar to ABE.

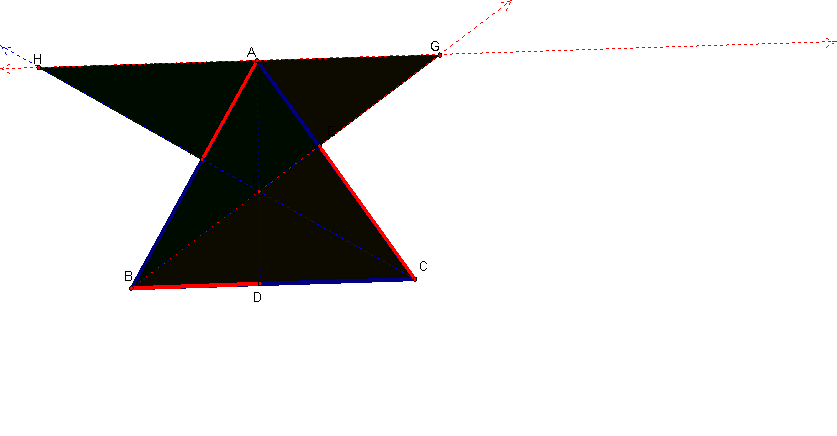
Since these triangles are similar, we know the following ratios are true:

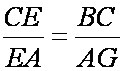and

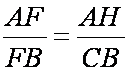
Because these triangles are similar, we can see from these ratios that their products are equal.

If we consider the products of these ratios, we can see that

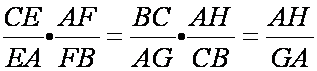
Furthermore, we can also see from the other pair of similar triangles that:

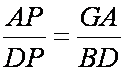and

Therefore,

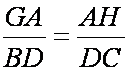
So, if we multiply the ratios, we will get a result of 1.

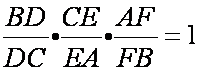
What happens when point P is outside of ABC?

If point P moves beyond ABC, we no longer have smaller triangles formed within ABC. Rather, we have only two triangles based on two of the remaining line segments. For instance:

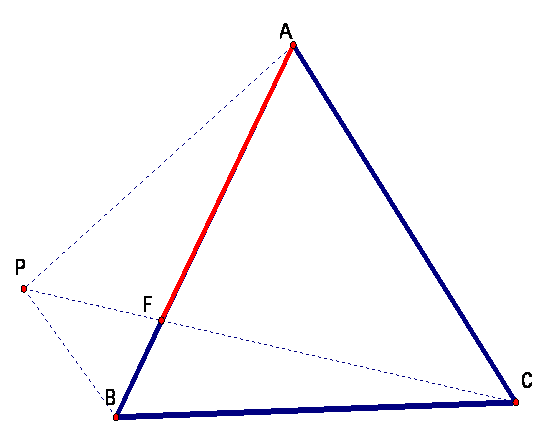
Furthermore, the ratio of these two line segments is not one.

Return to Lindsay’s Page