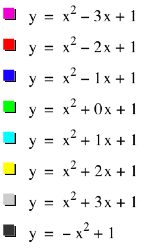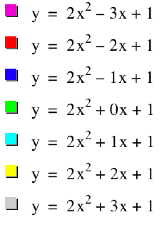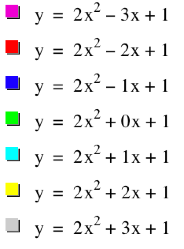Explorations of Quadratic Functions
By: Laura Lowe
It
has now become a rather standard exercise, with available technology, to
construct graphs to consider the equation 
and to overlay
several graphs of 
for different
values of a, b, or c as the other two are held constant. From these graphs
discussion of the patterns for the roots of 
can be followed.
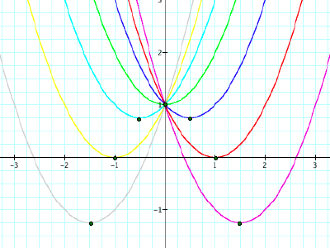
For example, if we set 
for b = -3, -2,
-1, 0, 1, 2, 3, and overlay the graphs, the following picture is obtained.
|
|
|
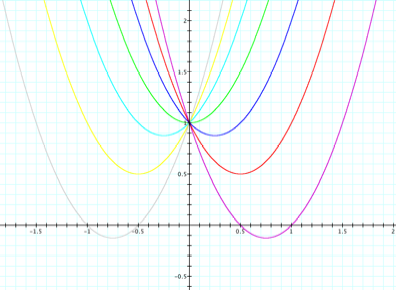
We
can discuss the "movement" of a parabola as b is changed. The parabola always passes through the
same point on the y-axis (the point (0,1) with this equation). For b < -2 the parabola will intersect
the x-axis in two points with positive x values (i.e. the original equation
will have two real roots, both positive).
For b = -2, the parabola is tangent to the x-axis and so the original
equation has one real and positive root at the point of tangency. For -2 < b < 2, the parabola does
not intersect the x-axis -- the original equation has no real roots. Similarly for b = 2 the parabola is
tangent to the x-axis (one real negative root) and for b > 2, the parabola
intersects the x-axis twice to show two negative real roots for each b.
Now consider the locus of the vertices of the set of parabolas
graphed from 
.
First
letŐs consider the set of vertices of the set of parabolas of the form 
.
|
|
|
From
the above graph we can see that the vertices of the graphs of the form 
are (-1.5, -1.25), (-1, 0), (-0.5, 0.75), (0, 1), (0.5, 0.75), (1, 0), and
(1.5, -1.25). The locus of these
points has the equation 
as seen below.
|
|
|
Now
suppose we start with the equation 
and we let b =
-3, -2, -1, 0, 1, 2, 3, and overlay the graphs, the following picture is
obtained.
|
|
|
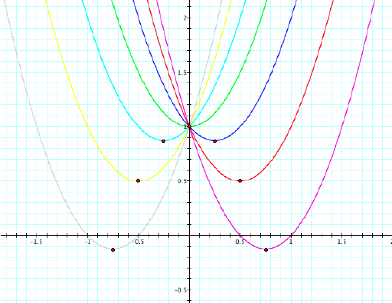
From
the above graph we can see that the vertices of the graphs of the form 
are (-0.75, -.125), (-0.5, 0.5), (-0.25, 0.875), (0, 1), (0.25, 0.875), (0.5,
0.5), and (0.75, -.125). The locus
of these points has the equation 
as seen below.
|
|
|
In
general, the locus of the vertices of the equations of 
, is the equation 
.
Click
here
to see a movie when a varies and c = 1.