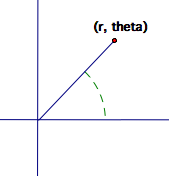
Polar equations define a relationship between an angle theta and a distance r. If we think about graphing a polar equation on the x-y plane, then the angle theta is measured from the positive x-axis and r is the distance from the point (0,0) on the x-y plane.

The equation of a circle centered at the origin in polar coordinate is very simple: r = a where a is the radius of the circle.

For example, below is the graph of r = 3.

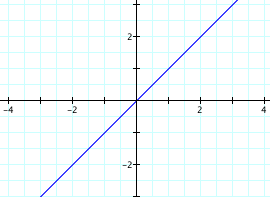
The equation of line through the origin is also very simple: Theta = b where b is an angle in radians.

For example, below is the graph of theta = pi/4.

Polar equations can also be used to graph some relationships with are not easily defined in rectangular coordinates.

Let's begin by looking at the equation 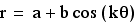when a = b. In this situation we get an n-leaf rose where k indicates the number of leaves.

a = 1, b = 1, k = 1

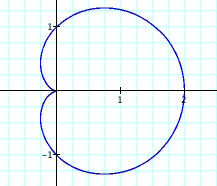
a = 1, b = 1, k = 2

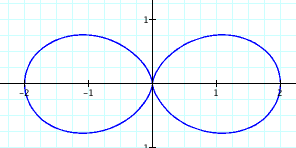
a = 1, b = 1, k = 3

When a = b, the sum of a and b gives us the "length" of our petals. If a = 0, we also get an n-leaf rose and the length of the petals is 1. In the above examples, the length of the petals is two. Below, we create a rose with petals of length 6.

a = 3, b = 3, k = 5

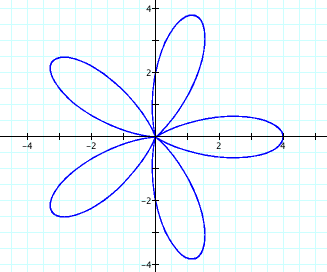
We can change the equation from cos to sin and we will get a similar graph, but rotated. Why does this change rotate the graph? (Discuss that cosine and sine equal 0 and 1 for different values of theta and why this results in a rotation.)

We can also alter the shape of the graph by choosing values of and b that are not equal. When a is greater than b, the petals are not tight (meaning they do not come back to (0,0) )but there are still k of them. The closer the value of a is to the value of b, the "tighter" the petals.

a = 2, b = 1, k = 3

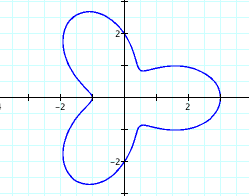
a = 5, b = 1, k = 3

Why are the petals not tight when a is greater than b? This question is equivalent to asking why r never equals 0 when a is greater than b. When a and be where equal, r = 0 when cos (theta) = -1. For example, a + b (-1) = a - b which = 0 when a and b are equal. When a is greater than b, a - b is always positive and in particular never equal to 0.

When a is less than b, the petals are tight and there are k large petals and k small petals. The larger the difference between a and b, the closer in size the small petals and the large petals become.

a = 1, b = 2, k = 3

a = 1, b = 5, k = 3

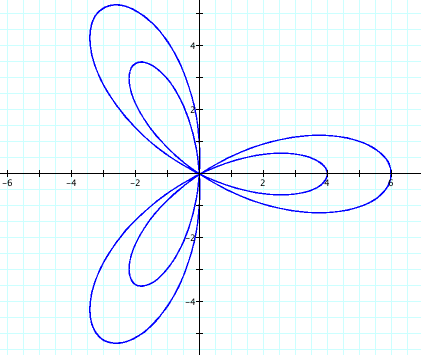
Why do we get twice as many petals when a <b? This question is equivalent to asking why r = 0 six times instead of three when a <b. If we look at 0 = a + b cos (theta) if we will get that b cos (theta) = a twice for each time cos (theta ) = -1. Once on each "side" of cos (theta) = -1. For example, if we look specifically at 0 = 1 + 2 cos(3 theta), we want to know when cos (3 theta) = -1/2. This will be when 3 theta = 120 degrees and when 3 theta = 240 degrees, as theta goes from 0 to 2pi each of these happens 3 times (because the 3 coefficient on the theta essentially takes us from 0 to 2 pi three times).