Exploration of Parabolas

By Thuy Nguyen

In this exploration we want to see what happens when we construct the graphs for the parabola y = ax² + bx + c with different values of a, b, and c. We’ll start first by constructing the graphs for y = ax² with different values of a. The following are graphs for a = -2, 1, 2, in blue, purple, and red respectively:

We first note that when a is a negative value, the graph is reflected across the x-axis and the vertex becomes the maximum point. Next, we note that as the value of a increases, the parabola gets narrower with respect to the x-axis.

Now we want to fix a (let a = 1) and examine the equation y = x² + bx. Let b = -2, 1, 3 in red, blue, and purple respectively in the following graph:

We see that the values of b have an effect on where the parabola intersects the x-axis. Now let’s see what happens when we vary both the values of a = 2, 4, 6 and b = -2, 1, 3:

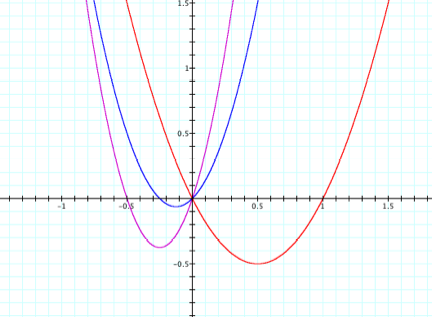
Ah ha! So the parabola has two points of intersections: (0,0) and (- (b/a),0).

Finally, we want to examine how the values of c can affect the parabola. We will fix a = 1 and b = 0 and vary c = -2, ½, 2 in red, blue, purple respectively:

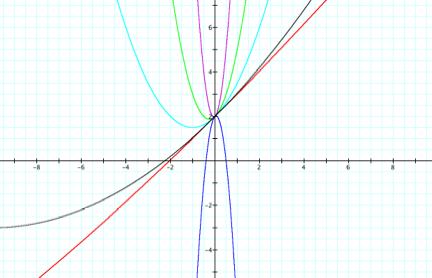
We see instantly that c determines the intersection of the parabola on the y-axis. That is, the parabola y = ax² + bx + c intersects the y-axis at y = c. Let’s see what happens when we vary the values of a:

y = 10 x² + x + 2, purple

y = -10 x² + x + 2, blue

y = 2 x² + x + 2, green

y = 1/2 x² + x + 2, teal

y = 1/20 x² + x + 2, dark grey

y = 1/100 x² + x + 2, red

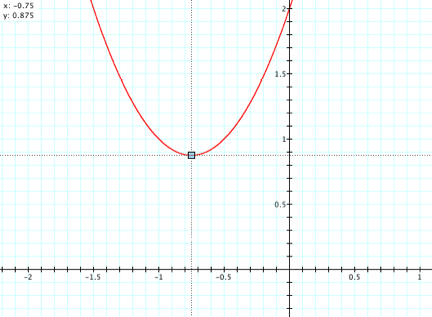
We note here that when c is introduced into the equation y = ax² + bx + c, it is no longer true that the parabola intersects the x-axis at x = 0 and x = -(b/a). But we are able to make a connection with b to the graph when c is introduced: The vertex of the parabola is (b/2a, b²/4a - b²/2a + c). We get the x-coordinate in the vertex by graphs examination and when x = -b/2a, then y = a(bb/4aa) + -bb/2a + c = bb/4a – bb/2a + c = b²/4a - b²/2a + c. Let’s do an example! Let a = 2, b = 3, c = 2. Then we should have

vertex = (-b/2a, b²/4a - b²/2a + c)

= (-3/4, 9/8 – 9/4 + 2)

= (-0.75, 0.875)

Graphing Calculator agrees with our answer:

Return