Gayle Gilbert & Greg Schmidt

Let be a point on a circle of radius

Consider the curve, which is traced out by the point as the circle rolls along the -axis. We will allow that our circle begins to trace the curve with the point at the origin.

Click here to see the animation in GSP.

Such a curve is called a cycloid.

Now, we can find the parametric equation fir the cycloid as follows:

Let the parameter be the angle of rotation of for our given circle. Note that when the point is at the origin.

Next consider the distance the circle has rolled from the origin after it has rotated through radians, which is given by

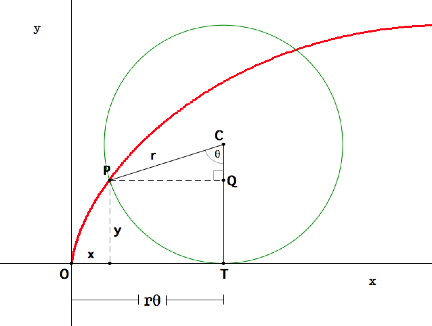
And so we can see that the center of the circle is given by .

Now, letting the coordinates of P be we have that

and

Hence, we have

which gives us the parametric equations of the cycloid.

Return