
Circles and
Lines
By Pei-Chun
Shih
1. Central Angle Theorem:
The central angle subtended by two points
on a circle is twice the inscribed angle subtended by those points.
á Definitions:
An
inscribed angle: an angle formed by two chords in a circle that have a
common endpoint. This common endpoint forms
the
vertex of the inscribed angle. For example, Angle BAC is an inscribed angle.
A
central angle: an angle whose vertex is at the center of the circle. For
example, Angle BOC is a central angle.
An
intercepted arc: the arc, such as BLC, inside a central angle or inscribed
angle is called the intercepted arc.

Click HERE to see the animation of point A.
á Proof of
the theorem:

á Discussion
about how to use technology to help students understand this theorem:
I would
open the Geometer's Sketchpad file linked above and ask my students to click
the animation bottom to observe the relationship of different inscribed angles
and the central angle with the same intercepted arc.
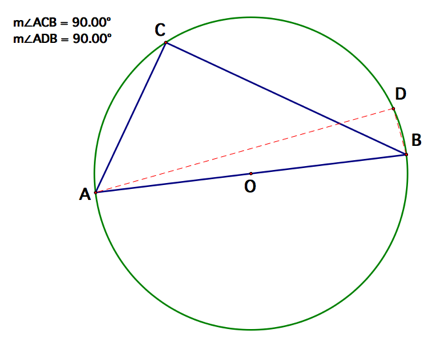
2. Inscribed Right Triangles:
(a) If a right triangle is inscribed in a
circle, then its hypotenuse is a diameter of the circle.
(b) If one side of a
triangle inscribed in a circle is a diameter of the circle, then the triangle
is a right triangle and the angle
opposite the diameter is the right angle.
Click HERE to see the animation of point C.
á Proof of
the theorem:
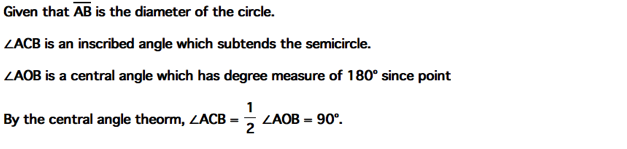
á Discussion
about how to use technology to help students understand this theorem:
It might be
a good idea to bring student to the computer lab and offer them opportunity to
construct relations (a) and (b) by themselves.

3. Intersecting Chord Theorem:
When two chords intersect each other
inside a circle, the products of their segments are equal.
á Definitions:
A
chord: a line that links two points on a circle or curve. It is a line segment
that only covers the part inside the circle.
A
diameter: a chord that passes through the center of the circle.
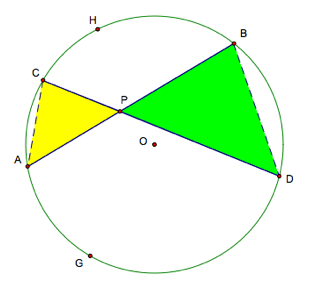
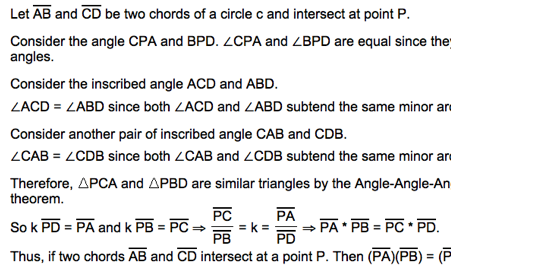
Let A, B,
C, and D be points on a circle, with A not equal to B,
and C not equal to D.
Suppose
that the lines AB and CD intersect at a point P. Then (PA)(PB) = (PC)(PD)
Click HERE to see the animation of point A.
á Proof of
the theorem:
á Discussion
about how to use technology to help students understand this theorem:
I would
open the Geometer's Sketchpad file linked above and ask my students to click
the animation bottom to observe that PA*PB is always equal to PC*PD no matter
where the chords are.
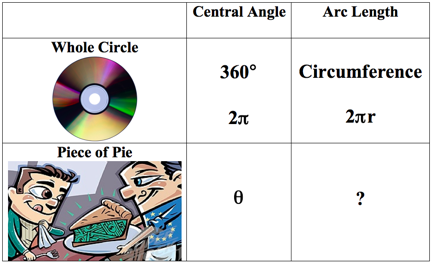
4. Length of an Arc:
The length of any circular arc s is equal
to the product of the measure of the radius of he circle r and the radian
measure of the central angle q that it subtends.
á Relationship
between a whole circle and a circular arc:

Click HERE to see the change of the arc length in different
angle measures.
á Proof of
the relationship:
Here, the
circumference of a circle is an arc length of the whole circle. By definition, p is the ratio of the
circumference of a circle to the diameter which equals two times of the radius,
r. Therefore, p =
circumference/2r.
In the
circle above, the arc length S is a portion of the circumference of the circle.
Therefore,
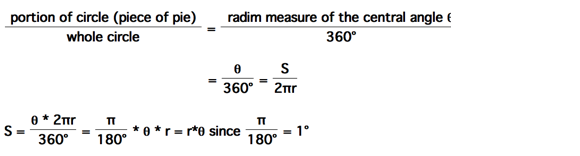
á Discussion
about how to use technology to help students understand this relationship:
Again,
using the Geometer's Sketchpad file to show different arc lengths with
different central angles is a good way to help students to understand this
concept. I also try to use some real-life examples, such as using a disk to
represent a whole circle and a piece of pie to represent a portion of circle,
to enhance studentsÕ understanding of the proof stated above.