Unit on Spherical Trigonometry

by Shawn D. Broderick

Day 2 Lesson Plan

Lesson Plan Title:

Introduction to Spherical Trigonometry

Concept/Topic To Teach:

Selected Explorations of Spherical Trigonometry

Standards Addressed:

Principles & Standards for School Mathematics Geometry Standard for Grades 9-12: “Analyze characteristics and properties of two-and three-dimensional geometric shapes and develop mathematical arguments about geometric relationships. “Specify locations and describe spatial relationships using coordinate geometry and other representational systems” (NCTM, p. 309, emphasis added).

General Goal:

Students will be able to use the Spherical Easel technology tool to investigate spherical trigonometric relationships and make comparisons to planar trigonometry. Through exploration, students will gain knowledge of the similarities and differences of trigonometric properties of segments, angles, and triangles in spherical geometry.

Specific Objectives:

1. To investigate conventional methods for measuring segment lengths and angles in spherical geometry.

2. To determine the maximum interior angle sum of a spherical triangle.

3. To construct a spherical triangle containing one, two, and three right angles.

4. To investigate the Law of Sines and Cosines and determine if they hold true for spherical trigonometry.

5. To explore spherical polygons and polyhedra and determine their characteristics.

Required Materials:

One computer is needed for each group of three students. The computer should be equipped with “Spherical Easel” which can be downloaded from http://merganser.math.gvsu.edu/easel/download.html. Pencils are also needed.

Anticipatory Set (Lead-In):

Open the class with an activity for the students to develop their own methods of measuring angles and segments on a sphere or in spherical geometry. Select several students to present their methods to the class. Facilitate a class discussion of the advantages and disadvantages of each method. From the presentations, identify the conventional methods for measuring angles and segments in spherical geometry. If no student has presented the conventional method, derive it for them.

Conventional Methods:

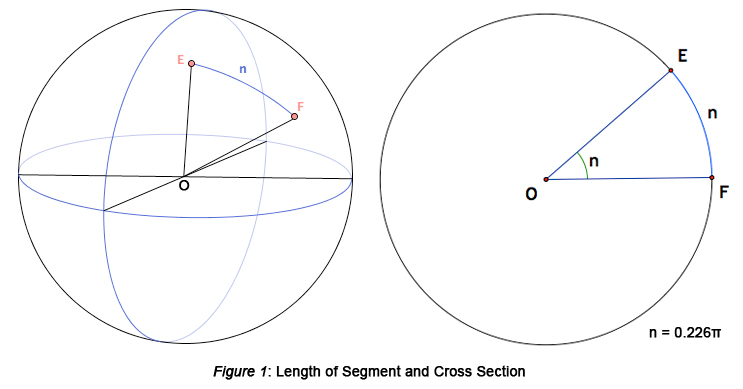
Measuring Angles is the same in radians. Measuring Segments is usually done using radians, because the segment is thought of as an arc of the circle resulting from the cross-section of the sphere (see Figure 1).

Have the students draw any spherical triangle. They might draw one similar to a planer triangle. Have the students label the segments and angles. Then ask the students how they would measure the segment lengths and angles. A conventional way to do this is shown in Figure 2:

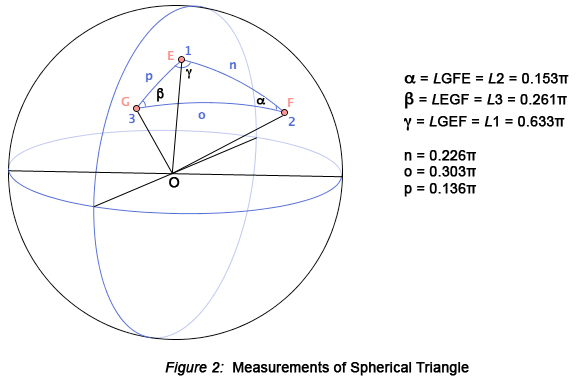
Notice that the sum of the angle measures in this example are: 0.153p + 0.261p + 0.633p = 1.047p. This is more than p or 180°. The students will discover this in their worksheet.

Step-By-Step Procedures:

1) Have the students form groups of three. Each group should relocate to a computer and complete the attached worksheet.

2) Tell the students that the whole class will discuss the answers to the worksheet and each group will present any discoveries that they have found. Each group will participate in discussing answers.

Plan For Independent Practice:

For homework, let students write half a page of information about spherical trigonometry based on their class work. They may also consult any sources of information including the Internet. The students will also find at least one real world application that can be solved by using Laws of Sines or Cosines of spherical trigonometry. They will also finish the worksheet if they did not in class.

Closure:

Using a whole class discussion format, students will discuss the answers to questions 1-6 on the worksheet. Students will present their solutions to the class using their computer on a projector or the board.

Assessment Based On Objectives:

Informal assessment will be performed by the educator. The educator will assess groups on the answers to the worksheet questions and the discoveries provided. Also, individuals will be assessed on participation in groups and whole class discussions.

Adaptations (For Students With Learning Disabilities):

Provide more time for the students with learning disabilities to complete the worksheet and to make discoveries. Provide all students with the Internet address for the Spherical Easel application so that they can have access at home.

Extensions (For Gifted Students):

Students are encouraged to explore and discover with Spherical Easel conjectures and theorems of spherical polygons and spherical polyhedra.

Possible Connections To Other Subjects:

Physical Science

Comments:

This lesson plan uses technology as a tool to encourage independent exploration and discovery of mathematics. Connections are also made between mathematics and real world applications by completion of the homework assignment in which students are asked to find a real world application of the law of sines and cosines for spherical trigonometry.

Reference:

National Council of Teachers of Mathematics. (2000). Principles and Standards for School Mathematics. Reston, VA: NCTM.