Chapter 1: Start with the Basics
I sat in the room waiting for something to happen, I am not particulary fond of the paranormal so it is little say that I was freaked out. Suddenly I heard something moving on the table, I jumped up and grabbed my lab top to recreate what I saw using GSP.
The chalk was moving in a line that is parallel to the base of the triangle created by the pocket watch and glasses. The ghost line as well seems to be parallel, but what special point of the triangle would move in such a way. I noticed first that the point is always on the interior of the triangle no matter how obtuse the triangle became. Since the points of the base of the triangle were not moving I decided to connect the chalk and the moving point of the ghost point with a rubber band, surprisingly the rubber band stayed (small freak out) on the ghost point.
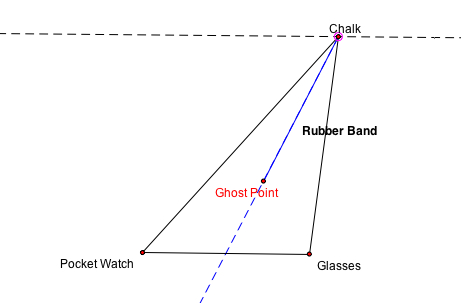I imagined the line would continue and would cross through the midpoint of the base. While the chalk moves back and forth we see that were the rubber band would cross the base does not change, so it cannot be the perpendicular line to the vertex, however, it does seem like it is the midpoint of the base. That would mean that the ghost point is the centroid or the place were all the medians intersect. So why was the line parallel to the base, simple, because the point is the median we know one of the main things about the centriod is that the distance from the centriod to the midpoint is one third the distance from the midpoint to the opposite vertex. So the distance between the midpoint and the centroid will always be the equal distance thus parallel.
I could hear my grandpa's laughter in the back of my head, it was never bad, his laughter was always one of pure satisfaction.
Feeling particulary proud of myself I asked for another, and of course my grandpa delivered.
This graph was particularly interesting because the ghost point not only moved inside of the triangle but it also floated outside of the triangle. In addition, this particular point passes through the pocket watch and the glasses. From looking at the track of the ghost point we can see that the path the ghost point follows is a parabola. To figure out which of the points of concurrecy I was looking for I needed to figure out a couple of more things first. I decided to find at what point the ghost point passes through the Pocket Watch and when the ghost point passes through the Glasses. Always ready with my handy dandy protractor, which I always carry with me since I am a professional mathematician, I was able to measure the the angle when the ghost point was at the pocket watch. When the ghost point passes through the Pocket Watch I found that the angle was 90 degrees. In the same manner when the ghost point passes though the Glasses it also was at a 90 degree angle. This was awesome, because that would mean that the ghost point was following the trajectory of the orthocenter of the triangle. The orthocenter is the point of concurrency for the altitudes of a triangle. Since the ghost point passed through both the Pocket Watch and the Glasses that means the line of symmetry for the parabola would go through both the vertex and the midpoint of the base of the triangle. This line would also be the perpendicular bisector of the base.
I heard a whisper say "impressive," but I was not sure if that was my ego or grandpa.
Until I saw the ghost point do something different.