Tangent circles

by

Hee Jung Kim

 

I. Given two circles C(1) and C(2) centered A and B respectively and an arbitrary point P on one of the circles, we can construct a circle tangent to the two circles.

1. When C(2) is included in C(1), and P is an arbitrary point of C(2), we can construct two tangent circles T(1) and T(2). In the construction it does not matter whether P is on C(1) (See GSP) or on C(2) (See GSP). Here are Script Tools for the tangent circles constructed.

1.2. The tangent circle T(2)
a. The locus of the center S2 of the tangent circle T(2) is an ellipse LT(2). (See GSP)

b. The locus of the midpoint M2 in the construction is a circle LM(2) in which the locus ellipse LT(2) is inscribed. (See GSP)

c. The locus of the line passing through the midpoint M2 and the center S2 of the tangent circle T(2) is in fact the locus of the tangent line of the ellipse LT(2). (See GSP)

To sum up, see GSP.

II. The two given circles C(1) and C(2) centered A and B overlap.

The GSP exploration shows that the locus of the center if one of the tangent circles is an ellipse and the locus of the midpoint in the construction is a circle. They are tangent each other at two points.
The locus of the center if the other tangent circle is a hyperbola and the locus of of the midpoint in the construction is a circle. They are tangent each other at two points.

III. The two given circles C(1) and C(2) centered A and B are disjoint.

The GSP exploration shows that the locus of the center if one of the tangent circles is a hyperbola and the locus of the midpoint in the construction is a circle. They are tangent each other at two points.
The locus of the center if the other tangent circle is a hyperbola and the locus of of the midpoint in the construction is a circle. They are tangent each other at two points.

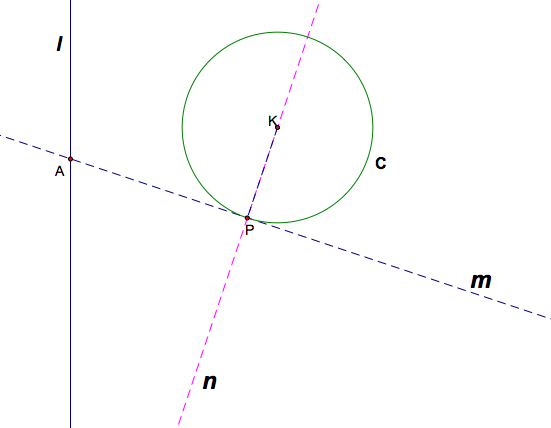
IV. Given a line l and a circle C with center K, and an arbitrary point P on the circle C, we will construct two circles T(1) and T(2) tangent to P and l.

The key idea of the following construction is that the center of the tangent circle should be equidistance with the point P and the line l.

1.1 Construction of the tangent circle T(1)

Step 1. Draw a tangent line m at P to the circle C. We note that a line tangent at a point to a circle is the line perpendicular to the segment from the center of the circle to the point on the circle. Let's denote the intersection of the line m and l by A.

Step 2. Because the tangent circle touches the circle C at P, the center which we are looking for should be located on the line through P and K. Therefore, we extend the line segment from K to P to a line n.

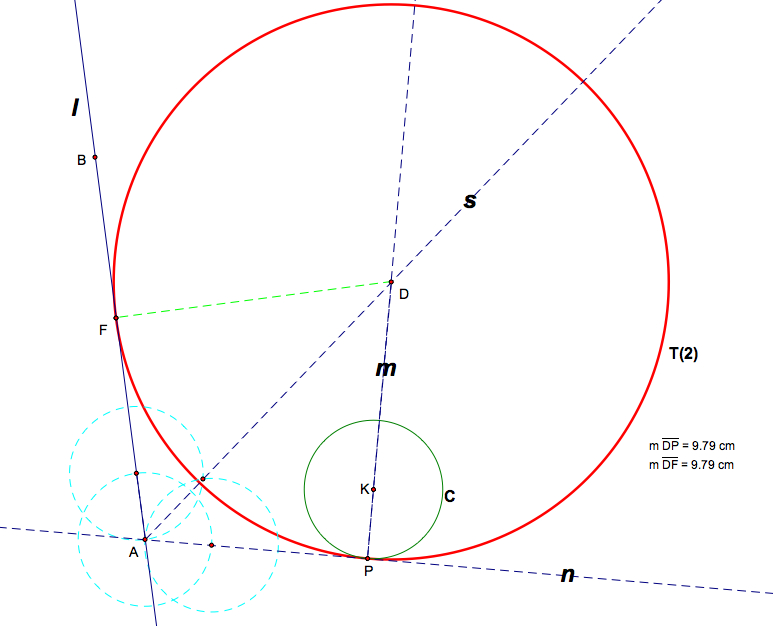
Step 3. Let B be any point on the line l. Draw the bisector s of the angle BAP since we are looking for a point equidistance from the line L and P. The bisector of an angle is the line that divides the angle into two equal parts, which is the locus of points equidistant from the two rays forming the angle. Let the intersection s and n be D.

Step 4. With the measure of the length of the line segment PD, we can construct a circle T(2) (centered at D and radius m(PD)) tangent to the line l and a given point P of the circle C.

2.2. Construction of the tangent circle T(2)

Step 1. Because the given circle and the tangent circle T(2) are tangent at P, the center K of C, the center of T(2), and P should be colinear. Draw a ray m from P to K.

Step 2. Draw a line n perpendicular to the line m and denote the intersection of the line n and the given line l by A. Let B be an arbitrary point on the line l.

Step 3. We need the bisector s of the angle BAP as the first construction. Let's denote the intersection of the angle bisector s and the ray m by D.

Step 4. With the measure of the length of the line segment PD, we can construct a circle T(2) (centered at D and radius m(PD)) tangent to the line l and a given point P of the circle C.

The loci of the centers of the tangent circles constructed are parabolars (see GSP), however, due to shortcoming of the construction, we can see only half of parabolas. Therefore the following GSP construction will show the correct loci. In other words, the loci of the centers of the tangent circles are paralolas and the loci of the midpoints in the construction are lines parallel to the given line and are tangent to the corresponding parabola which is the locus of the center of the tangent circle.