

The Pedal Point Goes Where and Does What?
by
Priscilla Alexander
This write-up is for students learning about the relationship of the pedal point and pedal triangle to the center of triangles.
How to create a pedal triangle.
First create a triangle on geometer sketchpad with the line option.
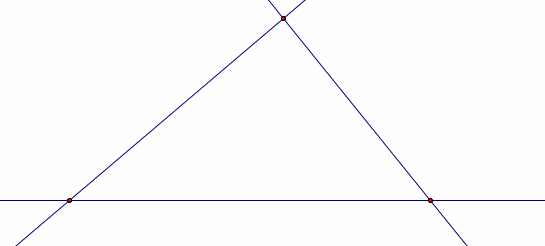
Next choose an arbitrary point outside of the triangle. Then make perpendicular lines from the point to all three sides of the triangle. Mark a point where the perpendicular lines intersects the side that it is perpendicular to.
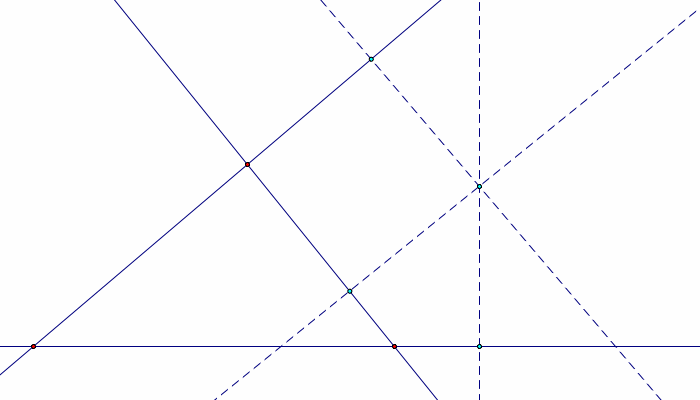
Lastly make segments of the points. We have a pedal triangle.
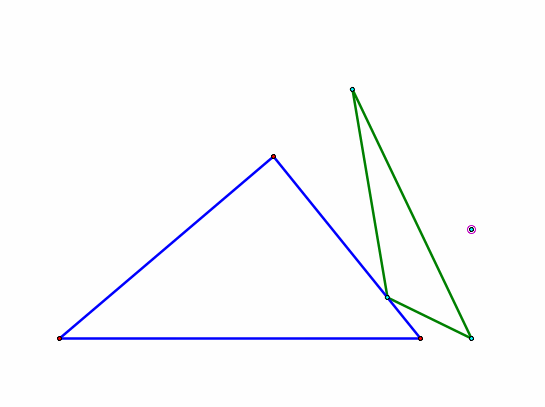
What happens when the pedal point is on the centroid?
Depending upon the type of triangle the pedal triangle may be inside or outside of the original triangle.
See sketches.

In this case two of the vertices of the pedal triangle touches two sides of the original triangle, while the other vertex is outside of the triangle and does not touch a side.

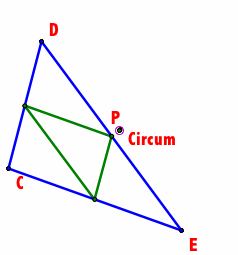
What happens when the pedal point is the circumcenter?
When the circumcenter is inside or outside of the triangle the pedal triangle always stays inside of the of the original triangle.
This happens because a medial triangle is formed. A medial triangle is formed by the midpoints of the sides of a triangle.
See sketches.
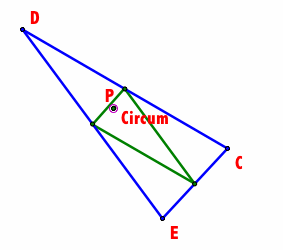
What happens when the pedal point is on the incenter?
The pedal triangle stays inside of the original triangle. This happens because the vertices of the pedal triangle are at the tangent points of the incircle.
See Sketch.
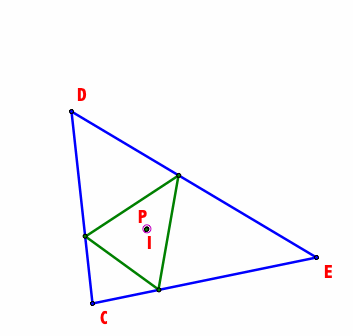
What happens when the pedal point is on the center of the nine point circle?
In one case, when the center of the nine point circle is outside of the triangle, the pedal triangle becomes degenerate.
See sketch
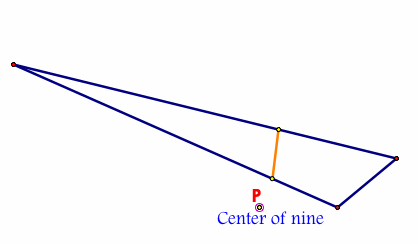
In another case, when the center of the nine point circle is outside of the triangle, part of the pedal triangle is inside of the original triangle. Two of the vertices of the pedal triangle are on the original triangle and the last one is on the outside of the triangle.
See sketch

In another case, when the center of the nine point circle is inside of the triangle the pedal triangle is always inside of the original triangle.
See sketch.
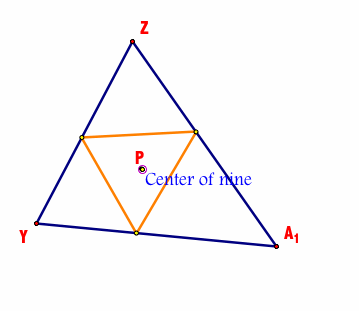
What happens when the pedal point is on the orthocenter?
When the orthocenter is inside of the triangle the pedal triangle is also inside of the triangle. The happens because the pedal triangle is the orthic triangle. A conclusion that could be drawn from this observaton is that the triangle CDE could be an acute triangle.
See sketch.
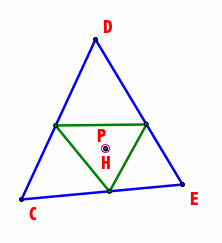
When the orthocenter is outside of the triangle, part of the pedal triangle is inside of the original triangle. Only one vertex touches a side of the original triangle.
See sketch.

What happens when the pedal point is on a vertex of the original triangle?
The pedal triangle becomes degenerate.
See sketches.
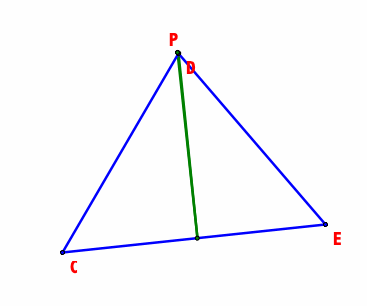

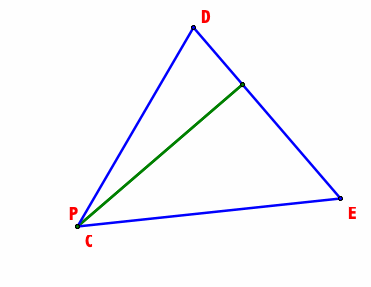
In conclusion, the pedal triangle behaviors differly amongst the triangle centers. Sometimes the pedal triangle is inside of the original triangle and sometimes it is part inside and part outside of the original triangle. In some cases it becomes degenerate.
To experiment more with pedal triangle click on the following GSP sketches.
Pedal Point and Nine point Circle