Orthocenters of Triangles
by
Tonya DeGeorge
The orthocenter of a triangle is the intersection of the three altitudes of a triangle. Remember, the altitude of a triangle is a perpendicular segment from the vertex of the triangle to the opposite side. For example, for the given triangle below, we can construct the orthocenter (labeled as the letter “H”) using Geometer’s Sketchpad (GSP):

In this investigation, we will see what happens to the orthocenter for different types of triangles. Does the orthocenter stay in one place or does it move depending on the type of triangle?
Equilateral Triangle:
To begin, let’s look at an equilateral triangle:
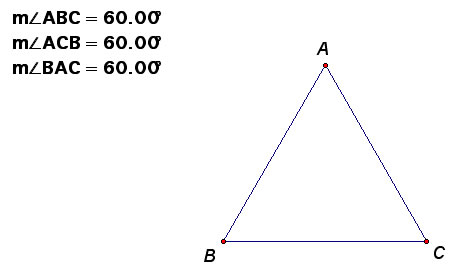
Given the equilateral triangle above (constructed by first creating a line segment and then constructing two circles with a radius equal to the length of the line segment to find the third vertex), we can construct the orthocenter, as follows:

From here, we can see that the orthocenter appears to be directly in the center of triangle, creating three equal triangles (with equal areas). To check this, first we can find the area of the larger triangle (ABC), and then find the area of each of the three smaller triangles:
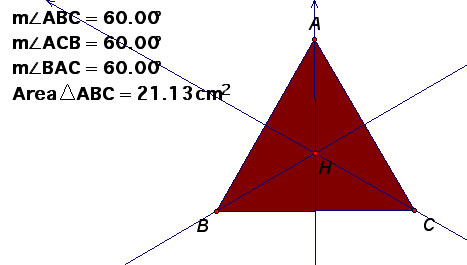
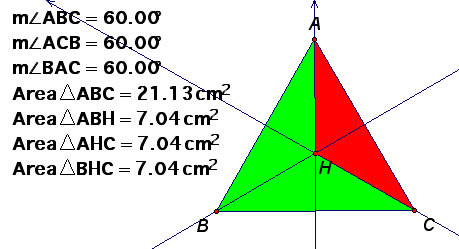
From above, we can see that, indeed, the orthocenter split up the triangle into three smaller equal triangles.
Acute Triangle:
Let’s look at other types of acute triangles and see if the orthocenter still remains inside the triangle. Suppose I have the following types of triangles (all acute):
And then I find the orthocenter of each one:
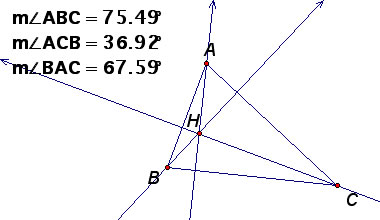
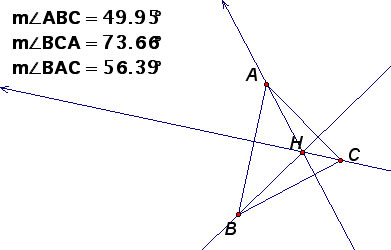
It appears that all acute triangles have the orthocenter inside the triangle. Depending on the angle of the vertices, the orthocenter can “move” to different parts of the triangle.
Isosceles Triangle:
Suppose we have the isosceles triangle and find the orthocenter (shown below):
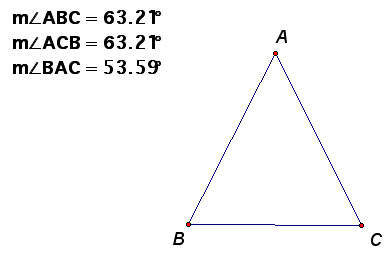
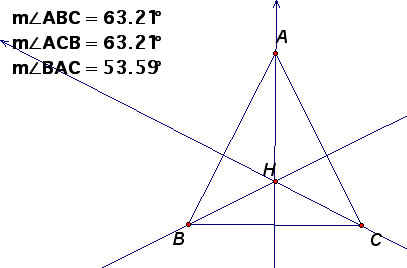
The orthocenter lies inside the triangle, as it does for all other acute triangles.
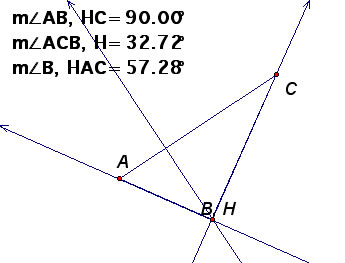
Right Triangle:
Let’s take a look at a right triangle. (We can construct this in GSP by creating a line segment and then creating a perpendicular line to that line segment.) Below is the right triangle created in GSP:
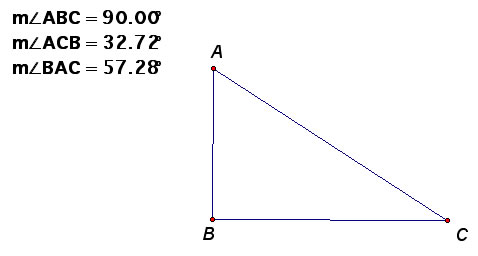
Again, we can create the orthocenter (H) by creating perpendicular line segments connecting each vertex to its opposite side:
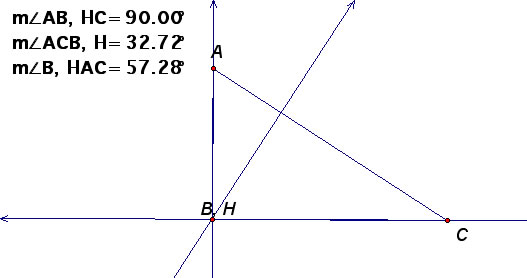
As you can see, the orthocenter has now “moved” to one of the vertices. In this case, the orthocenter lies on the vertex B, which contains the 90° angle. Even if we change the size or move any of the vertices of the triangle, the orthocenter remains on that vertex.
Obtuse Triangle:
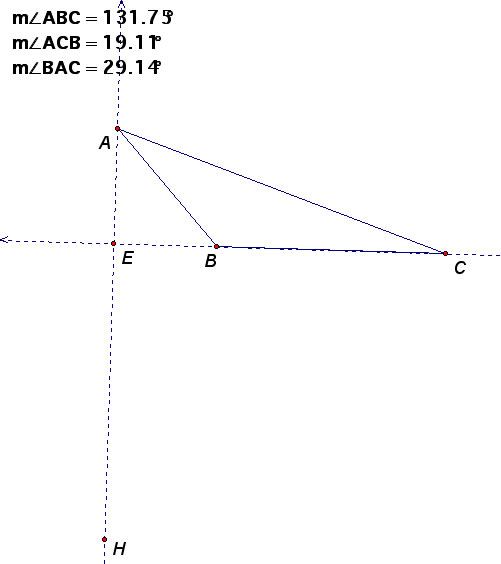
Suppose we have an obtuse triangle, as show below:

Where would the orthocenter be? We have already seen that the orthocenter can appear to “move” depending on the triangle. Let’s see what happens with this triangle. When we create the altitudes of each side, we get:

We can see that the orthocenter is now outside the triangle! Why does this happen? Well, it is a little bit harder to see how to construct the altitudes of an obtuse triangle because two out of the three altitudes cannot be drawn inside the triangle. For example, if we wanted to draw the altitude that connects the vertex A to the side BC, we would have to extend the segment BC (shown with a dashed line):

From here, we can see that the line segment AE and EC are perpendicular to each other.
We can clearly see that the orthocenter is on the same line as point E, which is the line that represents the altitude. Similarly, we can see the same thing when we construct the altitude that passes through the vertex C and the line segment AB:

Conclusions:
From this investigation, we can make some conclusions about the orthocenter. It seems that there are three overarching ideas:
1. If the triangle is an acute triangle, the orthocenter will always be inside the triangle. (Where inside the triangle depends on what type of triangle it is – for example, in an equilateral triangle, the orthocenter is in the center of the triangle.)
2. If the triangle is an obtuse triangle, the orthocenter lies outside the triangle.
3. If the triangle is a right triangle, the orthocenter lies on the vertex of the right angle.