

Final Assignment
Part 1
by
Margo Gonterman
Grazing Area
Farmer Jones had a goat on a tether. He tied the end of the tether not attached to the goat to a stake in a field. Over what area could the goat graze?
Of course you need to know something about the length of the tether and about the field.
There are two structures in the field: -- a shed that is 20 ft long and 20 feet wide (square) -- a silo that is 20 ft in diameter.
The center of the shed and the center of the silo are on a line and the distance apart is 92 feet. The distance from center to center is 112 feet.
The tether for the goat is 76.7 feet long.
The stake to which the tether is tied is somewhere along the line of centers between the shed and the silo.
Explore the area over which the goat can graze as the stake is moved along this line segment from the midpoint of the side of the shed to the edge of the silo.

Part 1: Tether Attached to the Center of the Shed (Square)
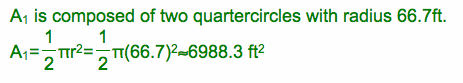
 |
 |


So the goat can graze over 7,915.3 square feet of field around the shed.
How much is the goat missing by having to go around the shed?
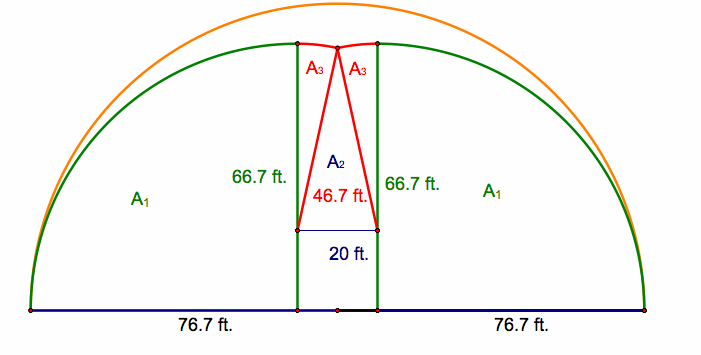
The orange circle represents the grass that the goat could have grazed on if the shed had not been in the field.

The goat is not able to graze on 925.5 square feet of field behind the shed.
Part 2: Tether between Silo and Shed (Rectangle)
The goat will be able to reach 76.7 feet on either side of the tether. The distance between the silo and the shed is 92 feet. Therefore, the goat will be able to graze in a rectangular area between the silo and the shed. The rectangle will have a length of 92 feet and a width of 2(76.7) feet or 153.4 feet.
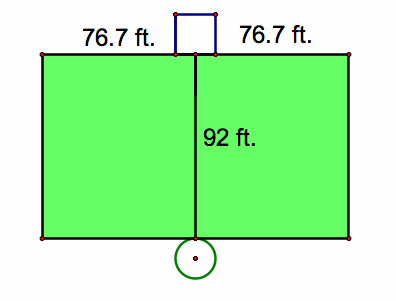
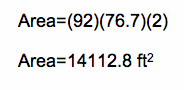
The goat will be able to graze an area of 14,112.8 square feet between the silo and the shed.
Part 3: Tether Attached to the Center of the Silo (Circle)
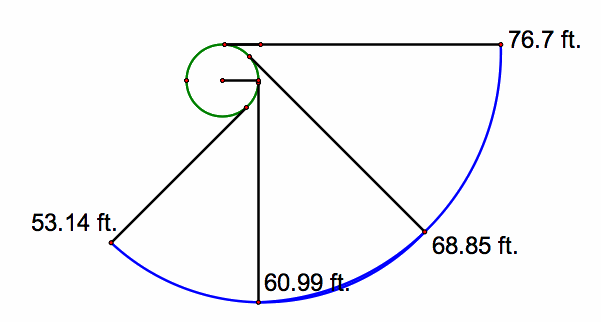
The length of the tether is calculated by subtracting the arc length from the original 76.7 feet at 4 different angles. The arc length is calculated by multiplying the central angle by the radius of 10 feet.
The calculations are shown below.
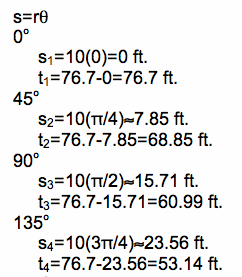
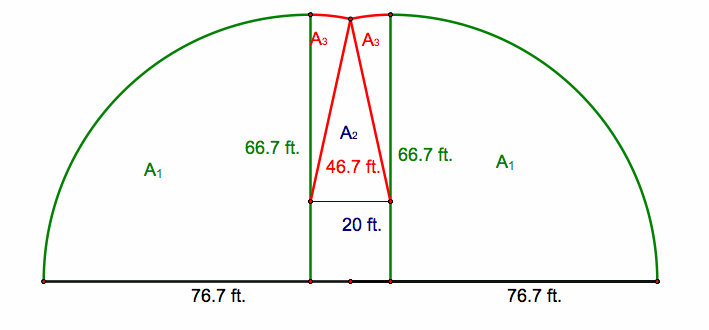
Notice that the picture above only shows half the area the goat can graze over. To get the full picture of the area over which the goat can graze, we will reflect this image.
The reflected measures are shown in blue.

Note that there is some overlap in the area that goat can graze.
Removing the overlap, the area over which the goat can graze is shown below.
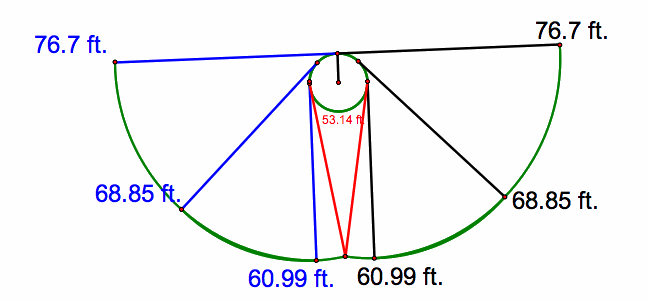
Now we want to estimate the area of this shape.

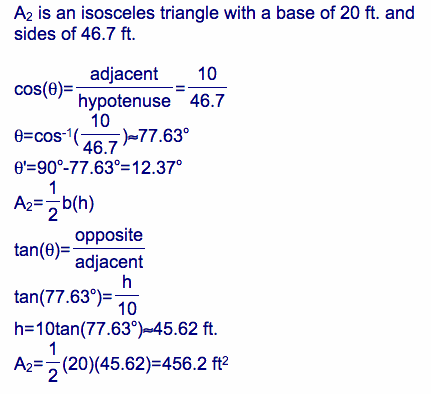
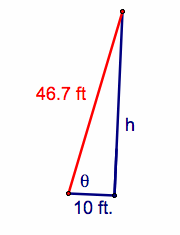
In order to figure out the area of the two small sectors, it is necessary to find the angle. In order to find the angle, drop a perpendicular to the base of the triangle. Because the triangle is isosceles, the perpendicular line will split the base into equal parts.
Considering only half of the triangle, it is possible to find the angle at the base by using cosine. Subtracting this angle from 90 degrees will give the central angle of the two small sectors.
Also, it will be necessary to find the height of the triangle to calculate the area. Again using only half the triangle, we can find the height by using the Pythagorean Theorem.
|
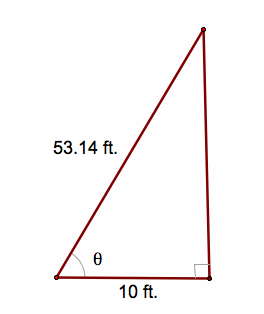 |
We now have all the information we need to estimate the area of this shape.
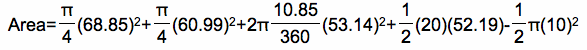
![]()
The goat has approximately 7,545.08 square feet to graze on behind the silo.
How much area is the goat missing by going around the silo?
If the silo was not in the field, the goat could graze over a semicircle with radius 76.7 ft. minus the area of the silo.
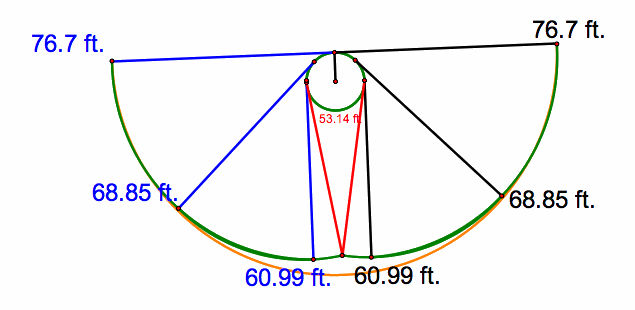
The orange circle represents the grass that the goat could have grazed on if the silo had not been in the field.

The goat is not able to graze on 1381.52 square feet of field behind the silo.
Total Area
The total area over which the goat can graze is equal to the sum of the three areas calculated above.
7915.3+14112.8+7545.08=29572.18
In total, the goat has 29,572.18 square feet (or .68 acres) over which to graze.