---

Explore problems of maximization such as the lidless box formed from a 5x8 sheet with a square removed from each corner.

Before biginning to solve this problem, imagine a lidless box.

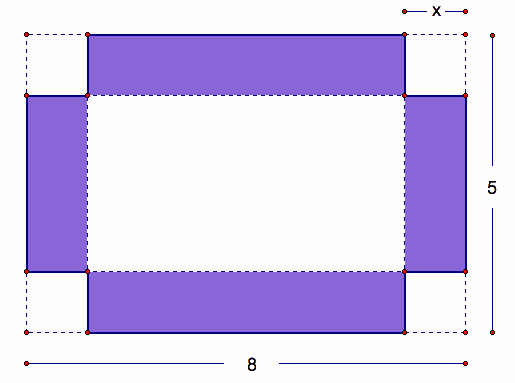
To get the volume of a lidless box, we have to know length, width and height.

We can know the maximum volume depends on height, so we have to explore the volume by various heights.

First, we can construct a formula for lidless box volume.

V=HxWxL

V(x)=x(5-2x)(8-2x)

Second, we have to consider the domain of x. Because the shortest side of the sheet is 5, we cannot remove greater than 2.5.

x must be less than 2.5 and be greater than 0 to form a box.

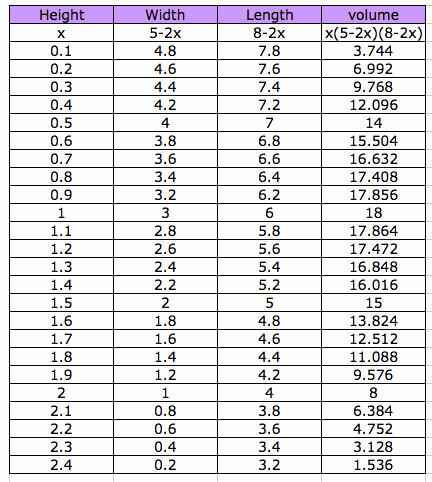
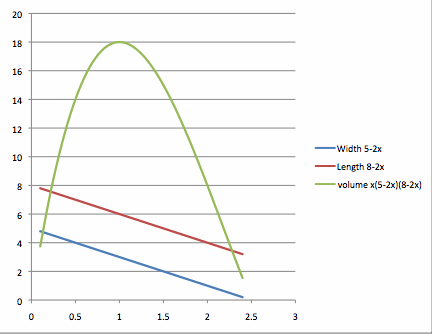
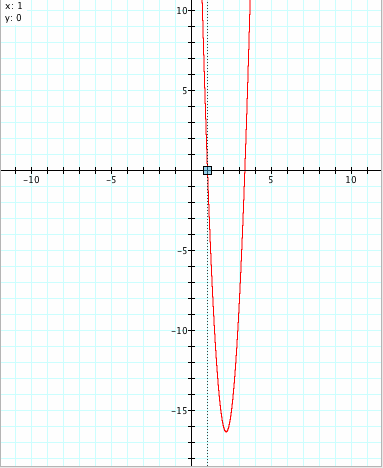
From the table and graph, when x=1, we can get the largest volume which is 18 cubic units.

We can also get the maximum volume by the derivation of V(x).

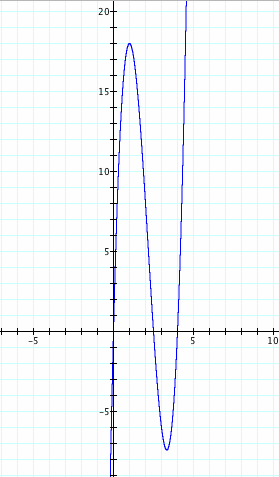
when

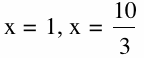
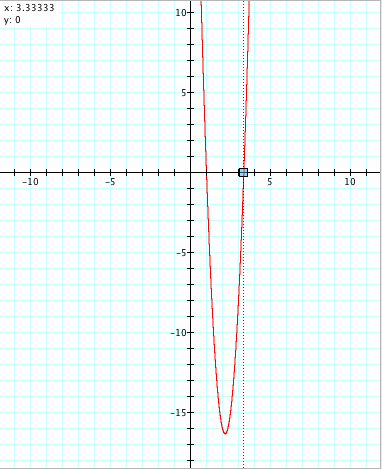
By finding derivative of V(x), we can confirm that x=1 is the maximum, and x=10/3 is the minimum.

Therefore, when x=1, we have the maximum volume which is 18 cubic units.