

Exploring Parametric Curves
by
Molade Osibodu
Objective: To show the various forms of a parametric equation.
A parametric equation is an equation defined using parameters such as x = f(t) and y = f(t). The most commonly used parameter is t. This exploration is going to focus on trigonometric functions and specifically sine and cosine functions.
We begin by graphing the equations x = cos (t) and y = sin (t) for 0 < t < 2pi
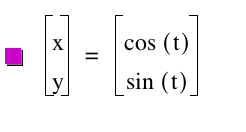

Figure 1: Graph of x = cos(t) and y = sin(t)
Notice that the graph of the equation has radius 1. Recall that the equation of a circle is
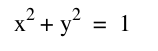
Therefore, the equation of our circle becomes
![]()
hence the radius being equal to 1
Next lets investigate the equations x = cos (at) and y = sin (bt) for 0 < t < 2pi
1st case: Let a = b. Notice that you still get a circle. Click here to investigate for different equal values
2nd case: Make a < b. Let a = 2b
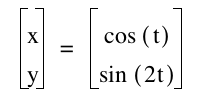
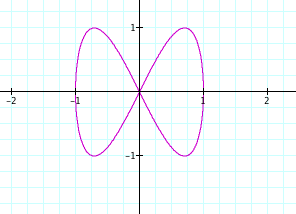
Figure 2: Graph of cos (t) and sin (2t)
Is the graph always the same as figure 2 when the ratio is 1/2? Click here to investigate. How about if the ratio is 2/3? After investigating, you should notice that equations with the same ratio of a/b produces the same graph.
3rd case: Make a > b
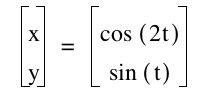
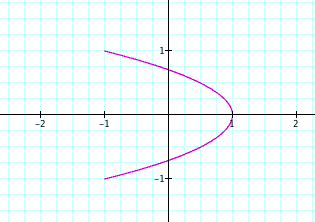
Figure 3: Graph of cos (2t) and sin (t)
Is the graph always the same as figure 3 when the ratio is 2? Click here to investigate. How about if the ratio is 3? After investigating, you should notice that equations with the same ratio of a/b produces the same graph.
Now, graph the equation with a=3 and b=1 and then with a=1 and b=3
|
|
Notice that the graph is just a reflection across the x-axis. Does this work is a=2 and b=1? Click here to investigate. You should discover that this only works when the number not equal to 1 is an odd number.
Finally, lets investigate the equations x = a cos (t) and y = b sin (t) for 0 < t < 2pi
1st case: Let a = b = 3.
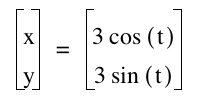
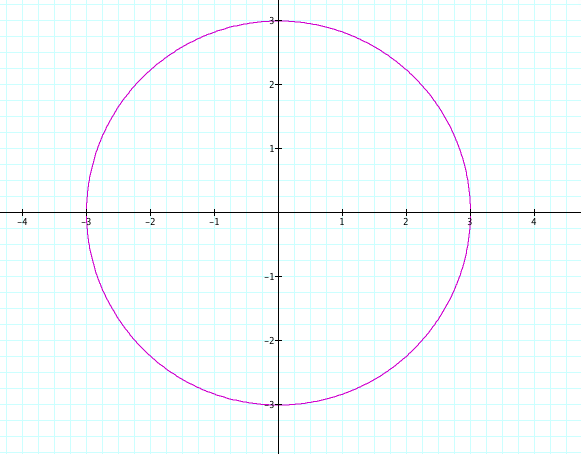
Notice that you still get a circle with a ratio of 3. Do you always get the same ratio as your value for a and b? Click here to investigate for different equal values
What happens when you make a=b=1 and then do the opposite? Do you still get the same reflection across the x-axis as previously stated? The answer is yes
Also notice that a always controls the radius while b controls the height. And finally, notice that when a is not equal to b, you get an elipse