Assignment 12: Problem 5
Jason P. Pickhardt
Problem:
Explore problems of growth, e.g. savings, interest compounded.
Ecologists develop predator-prey models to describe the relationship between certain species. Some systems developed by ecologists represent a variation of predator-prey models, namely, competing species models or predator-predator models. Consider the system in which there are two predators, owls and hawks, who share their food sources and whose populations are related by the system of dynamical systems:
What is the long term behavior of the system?
Note: This problem was retrieved from Dr. Conner’s EMAT6550 course during Fall ’09.
Exploration:
This problem involves both the potential for growth and decay which are mathematical situations that lend themselves to being modeled with Excel. It is not apparent however where to start this problem. It is up to us to choose starting values for the two populations in order to make observations on what will happen. Thus it is important to look at several cases for starting populations of hawks and owls in order to make some generalizations about the long term behavior of these dynamical systems. The first step I took in analyzing this problem was to see if I could manually calculate an equilibrium value for both populations. The equilibrium values are starting values for each population such that there is never any growth or decay in either population, hence there is equilibrium in the ecosystem. In order to do this we must understand what each term in the dynamic systems means.
: Starting population of Owls or nth iteration of our system
: Population after n+1
iterations
: Starting population of Hawks or nth iteration of our system
: Population after n+1
iterations
: Population growth of Owls
: Population decay of Owls due to interaction of Owls and
Hawks
: Population growth of Hawks
: Population decay of Hawks due to interaction of Owls and
Hawks
Now that we understand our terms, we must figure out how to find an equilibrium value. When we have equilibrium our original population will neither grow nor decay and thus we are looking for a starting value that gives us the situation where our n+1th term is the same as the nth term. More simply we are interested in the case when:
The hand calculation for finding these equilibrium values is as follows:
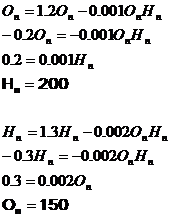
These calculations tell us that when the starting population of Owls is 150 and Hawks is 200 that the number of each will never change. The following clip from an Excel file shows us that this is true:
|
Owls |
Hawks |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
|
150 |
200 |
The first value underneath each predator type is the initial value, while the rest of the values were generated in Excel using the given dynamical systems described in the initial problem. It is worth while to note that this is not the only equilibrium that could exist in the ecosystem. Though trivial, if both populations began at zero then neither population would grow and obviously it could never decrease. However, this will be the only mention of this equilibrium given that the 150/200 equilibrium is far more interesting.
Now, it is interesting to consider all different populations of predators with respect to their equilibrium numbers. In exploring this problem I was able to come up with eight worth while cases to examine in terms of starting populations of the predators.
Starting Populations:
1) Both populations start below equilibrium.
2) Both populations start above equilibrium.
3) Owls start above equilibrium and hawks start below equilibrium.
4) Hawks start above equilibrium and owls start below equilibrium.
5) Owls start below equilibrium and hawks start at equilibrium.
6) Owls start above equilibrium and hawks start at equilibrium.
7) Owls start at equilibrium and hawks start below equilibrium.
8) Owls start at equilibrium and hawks start above equilibrium.
An Excel spreadsheet with examples of each case described can be found here. In the spreadsheet the cells highlighted in red indicate the iteration before that particular population will die out. Using this data it is possible to describe the long term behavior of the ecosystem described by the dynamical systems on a case by case manor.
End Behavior:
1) Owls die off and Hawks flourish.
2) Hawks die off and Owls flourish.
3) Hawks die off and Owls flourish.
4) Owls die off and Hawks flourish.
5) Owls die off and Hawks flourish.
6) Hawks die off and Owls flourish.
7) Hawks die off and Owls flourish.
8) Owls die off and Hawks flourish.
Conclusion:
In doing this exploration Excel proved to be a very powerful and useful tool. When examining the end behavior on a case by case basis we can conclude that the starting populations of both predators in the ecosystem have a direct influence on the end behavior. It is interesting to note that there are four cases where the owls die and four where the hawks die. It seems that whenever the population of Owls is above its equilibrium value they will flourish while the Hawks die off. In comparison whenever the Owl population is below its equilibrium value its population will die off. In the two cases where the Owls population is at equilibrium, the time they die of is when the Hawks start above their equilibrium value. The importance of this problem would be if an ecologist wants to set up an ecosystem in which both populations will remain. Using these dynamical systems and the analysis provided, there can be only one way for this to happen and that is at the equilibrium values.