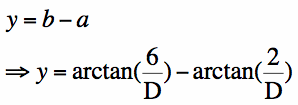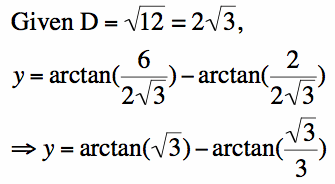Seeing the Full Picture
by
Mike Rosonet
A 4'x4' picture hangs on a wall in a museum such that its bottom edge is 2 feet above a person's eye level. How far back from the picture should the person stand, provided that he or she is standing directly in front of the picture, in order to view the picture under the maximum angle?
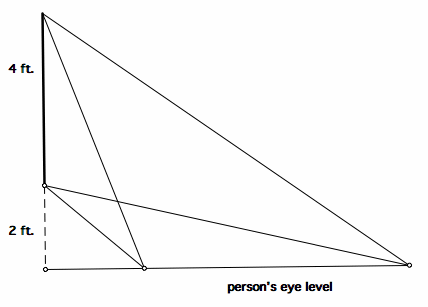
Consider the measures of the angles shown in the given figure.
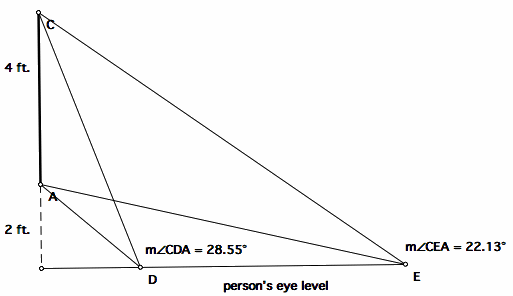
The angle measures seem to imply that a person would need to stand close to the picture in order to view the picture at the maximum angle.
Consider extending segment DE to F. Would the angle measure there be greater or less than the measure of angle CEA? What about a point G that is closer to the picture than D; would the angle measure be greater or less than the measure of angle CDA?
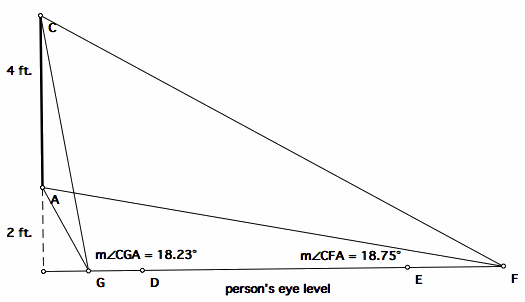
As seen in the figure to the right, any angle whose vertex is beyond E will have an angle measure less than that of angle CEA.
Also, any angle whose vertex is closer to the picture than D will have an angle measure less than that of angle CDA.
By comparing the above angle measures, the conjecture can be made that a person would need to stand close to the picture in order to view it at the maximum possible angle.
The question posed above was to find the exact distance that a person would need to stand from the picture in order to view it under the maximum angle. Therefore, a mathematical equation is needed in order to find the distance.
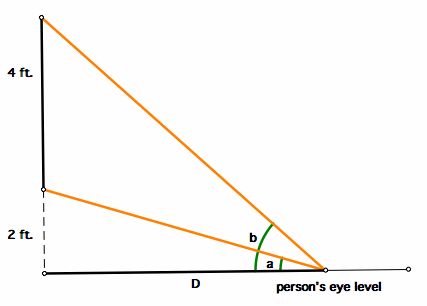Maximum angle = b - a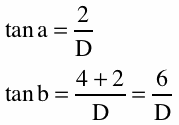
Let "D" be the distance between the wall and the vertex of the maximum angle in feet. Solving each of the above equations for a and b,
Since the maximum angle is b - a, set the maximum angle equal to y in order to visualize the equation.
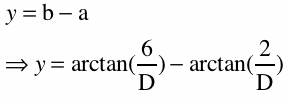
This is the graph of the function, with D = x, from x = 0 feet (the wall) to x = 16 feet.
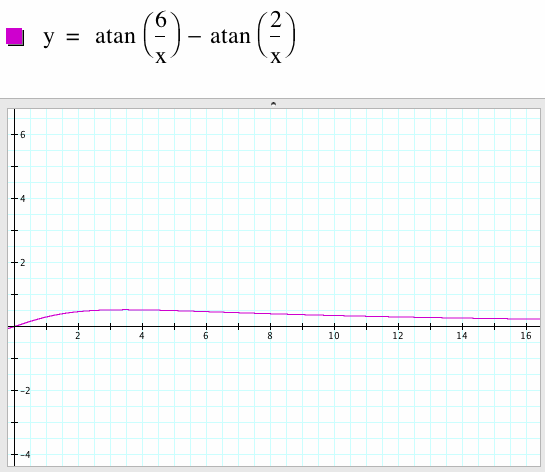
Thus, from the graph, it can be seen that the maximum angle is found within 5 feet of the wall.
Using calculus, find the maximum value of the function in order to find the distance from the wall where the maximum angle occurs.
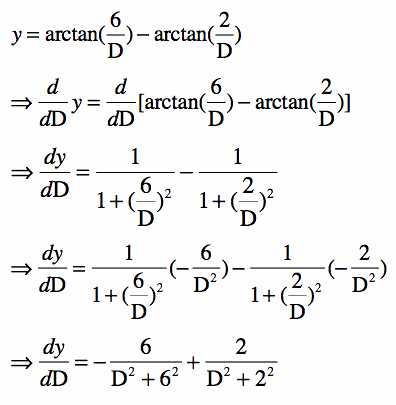
The maximum value is the point on the graph where the tangent line constructed through that point will have a slope of 0.
Thus, set
= 0 and solve for D.
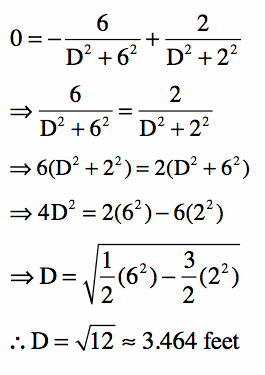
Hence, a person should stand approximately 3.464 feet from the picture in order to view it under the maximum angle.
EXTENSION 1
Now that the distance from the wall that will give the maximum viewing angle, what is the maximum viewing angle?
Remember, the maximum angle equation above:
Substitute the solution for D into the above equation and solve for y, the maximum angle:
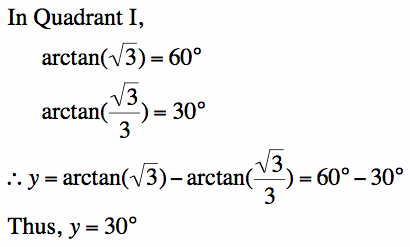
From the diagram above, it is safe to assume that both angles b and a are acute angles. Therefore, limit the angle measures to those only in Quadrant I. Hence,
The measure of the maximum viewing angle which is found approximately 3.464 feet away from the wall is 30 degrees.
Extension 2
Due to the popularity of the picture, the museum decided to place a bench in front of the picture. The bench is 2 feet tall. The bottom of the picture is 5.5 feet from the ground. Using your own height when sitting on the bench (assume that you are two feet shorter sitting down than when standing up), where does the front edge of the bench need to be positioned so that you will have the maximum viewing angle? What is your maximum viewing angle? (Assume that your eyes are in line with the edge of the bench.)