Polar Explorations

By: Brandie Thrasher

By exploring Polar equations, we can take a glance and construct the “n-Leaf Rose”. Let’s start off with the basic equation of r = b cos(kq)

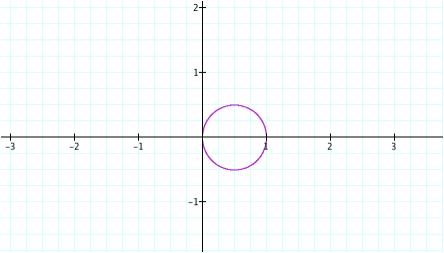
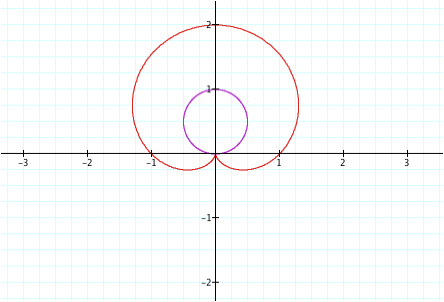
Having b and k equal 1, it appears that our equation yields a circle (with theta ranging from 0 to 2p). Lets overlay a similar equation, in which a constant “a” is introduced,

R = a + b cos (kq)

Again, we have b and k equal to 1 as well as a. We see that we no longer have a circle. Polar graphs usually have symmetry either at the polar axis, the pole or the line q = ^p/₂. Here the symmetry appears to occur at the polar axis.

Lets investigate various changes to a, b, and k, starting with k = 2.

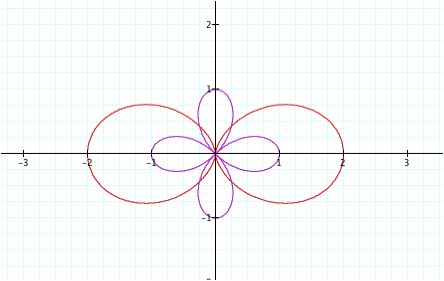
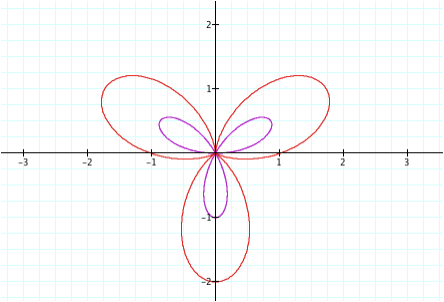
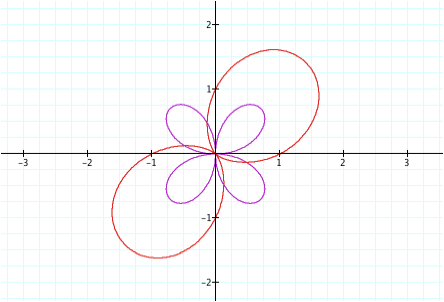
We can see that as k increases, we begin to see the pedals of the “n-leaf”. It appears that the equation r = a + b cos (kq) has exactly 2 symmetrical sides, while the equation r = b cos (kq) has symmetry that doubles the amount of k. It still appears that the symmetry rests along the pole. Will this pattern continue to hold true? Let’s increase k to 3 and compare.

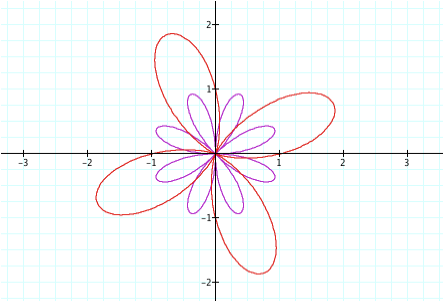
This assumption does not hold true, as both equations yield “pedals” of the same amount as k. Let’s again increase k to 4 and see the results.

It appears that with even values for k, the equation r = b cos (kq) will yield a graph that has the pedals the amount of 2k, while our equation r = a + b cos (kq) will always have pedals in he amount of k. (k increases by 1 each graph shown)

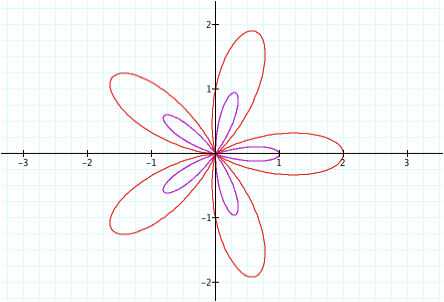
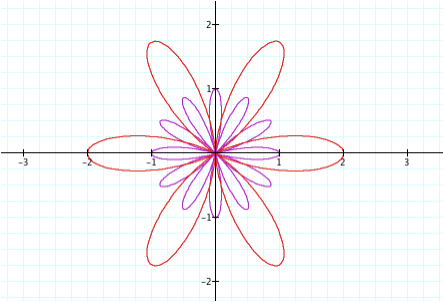
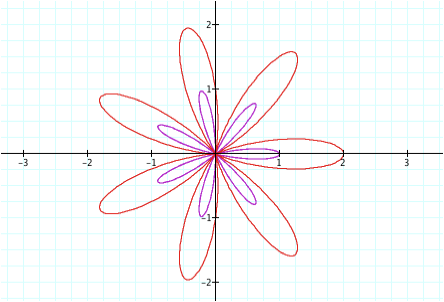
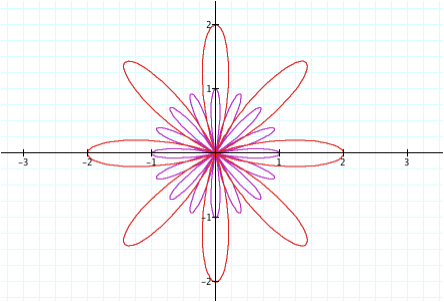
If we change the equations to r = b sin (kq) and r = a + sin (kq) or line of symmetry becomes q = ^p/₂

But just as before, the numbers of pedals are composed by the amount multiplying data, be it k or 2k (for odd or even).

Return