
T A N G E N
T C I R C L E
by
Jane Yun
LetÕs begin the investigation with the
following problem.
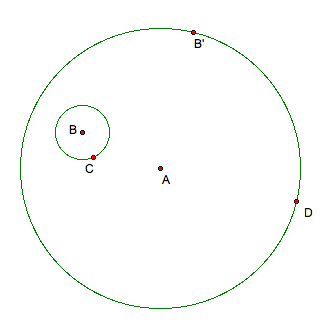
Let AD and BC be two
circles, and let BÕ be a point on the circle AD. Construct a circle tangent to the two (given) circles with
one point of tangency being the designated point.
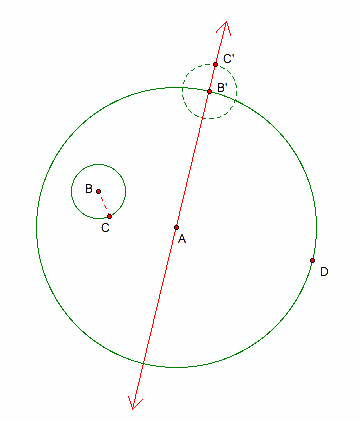
Draw a straight ABÕ and then, construct a
circle with center BÕ and radius BC. (The center BÕ of the desired circle BÕCÕ will lay along a line from the
center of the circle A with specified point BÕ.
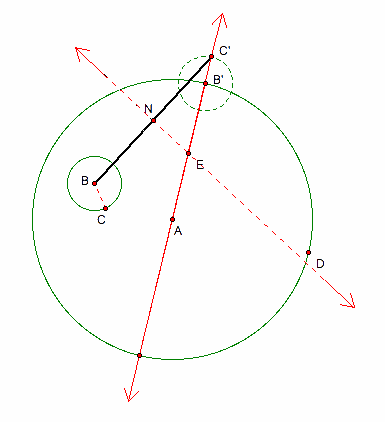
Draw a straight line BCÕ, bisect BCÕ at N, and draw a perpendicular line through N and the segment BCÕ. Then, let E be the intersection point of the perpendicular line and line ABÕ.
Next, construct a circle with a center E
and radius EBÕ.

Now consider the segments EB and EBÕ, which
is from the center E of the desired circle EBÕ to the center B of the second
given circle BC. These segments
are always the sum of length of EBÕ and BÕCÕ(or BC). The same distance lies off along the line through the given
point from the center of the desired circle.

Therefore, the center E of the tangent circle
EBÕ lies along the perpendicular bisector of the base BCÕ of the isosceles ÆBCÕE.
Summary
We have constructed a line through the
center of a circle of the same radius as the second of the given circles with
the designated point as center.
The intersection of the line and circle will allow construction of the
base of the isosceles triangle.
Hence, the intersection of the line and circle will allow location of
the center of the desired circle.
Given the construction, letÕs consider the
locus of the center of all circles tangent to the two given circles. By using GSP, we can animate around the
circle and trace the locus of the center.
Case 1: When the second circle is inside the first circle.
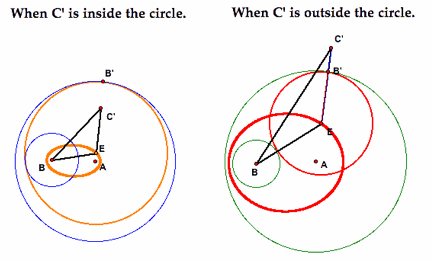
By animating point BÕ and tracing the locus
of the centers of the tangent circles for this case, I see that the loci of the
center of the tangent circles created an ellipse with the foci at the centers
of the given circle. Because the
sum of segments, EB & EA, is equal to the sum of radii of two given
circles, the sum is a constant. Therefore, the locus of the centers of the
tangent circles is an ellipse with foci at the centers of the given circles.
Case 2: When two given circles intersect.
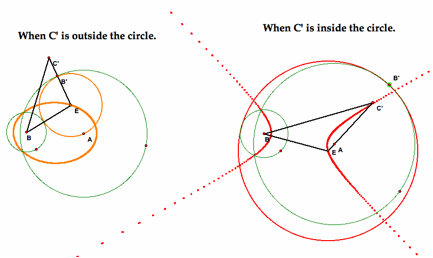
When CÕ is outside the circle, the locus of
the centers of the tangent is an ellipse with foci at the centers of the given
circles (by the same reason above).
But when CÕ is inside the circle, the locus
of the centers of the tangent created a hyperbola with foci at the centers of
the given circles. Because segment
BE - segment EA is equal to the segment ACÕ, the difference is constant.
Notice that the segment ABÕ is the radius
of the given circle ABÕ, so the radius is constant.
Then, it was previously shown that BÕCÕE is
an isosceles triangle. It follows
that segment BE = segment CÕE (by definition of isosceles triangle).
Then, segment EBÕ = segment CÕE + segment
BÕCÕ. By subtracting segment BÕCÕ,
you get segment CÕE = segment EBÕ – segment BÕCÕ.
Since segment BE = segment CÕE and segment
CÕE = segment EA + segment ACÕ, segment BE = segment EA + segment ACÕ.
By subtracting segment EA, segment BE
– segment EA = segment ACÕ, which is constant.
Therefore, the locus of the centers of the
tangent circles is a hyperbola at the centers of the given circles.
Case 3: When two given circles are disjoint
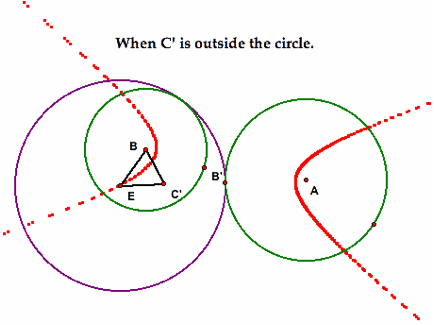
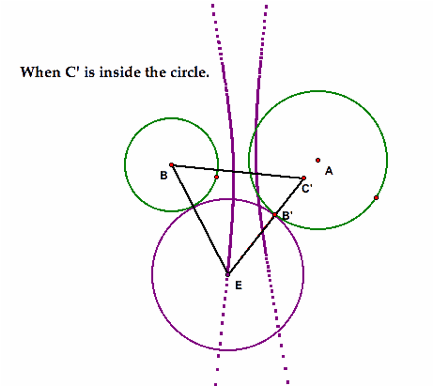
When CÕ is either inside or outside the
circle, the locus of the centers of the tangent is a hyperbola with foci at the
centers of the given circles (by the same reason above).