Assignment #11 – Polar Equations

Jackie Gammaro

This investigation involves polar equations. For this write-up, I am going to investigate some different polar equations and the differences in their graphs.

The three polar equation investigated are:

#1

#2

#3

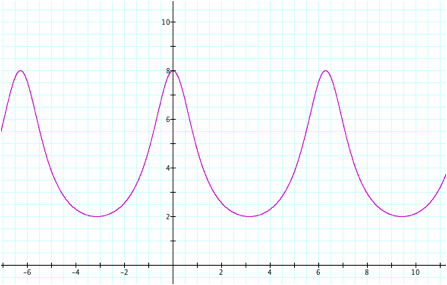
#1. First lets look at graph of .

Click on the link below to investigate the graph in Graphing Calculator 3.5.

Graph of #1

From the graph we can estimate:

1) The graph has a period of 2 and an amplitude of 3, this comes from the trig function 3cosq, which also has an amplitude of 3 and a period of 2.

2) The minimum value looks to be 2, while the maximum value is 8. We can prove this by taking the derivative of the function, setting it equal to zero then solving for theta. Then solve for r, for all values of theta.

Let

Let .

Let

#2. Now lets look at the graph of .

Click on the link below to investigate the graph in Graphing Calculator 3.5.

Graph of #2

One should notice this function is quite similar in both equation form and graphically to the polar equation: . It would be advantageous to compare graphs simultaneously on one set of axis, which sure enough Graphing Calculator allows.

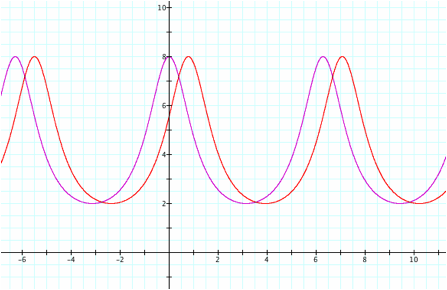
Here is a snapshot of the two functions on one coordinate plane.

Click on the link below to investigate the graph in Graphing Calculator 3.5.

Graph #1 and #2

One graph is the other graph shifted p/4 units. The graphs have the same period of 2p and an amplitude of 3. The minimum and maximum values of each graph are the same but not at the same values for theta. Again, we can take the derivative to find min and max values of the function.

Let , then .

Let

Let .

Let

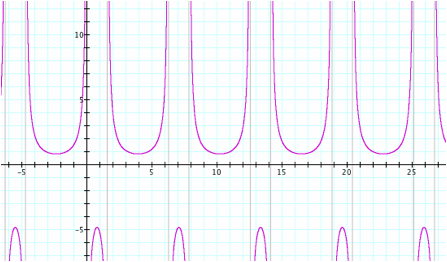
#3. Lets investigate now.

To get a better look at the graph and investigate independently click on the link below to view the curve in Graphing Calculator 3.5.

Graph #3

To determine the period of the graph, I look at values of q where there is an asymptote, values of theta where sin q - cos q = 1.

From the graph, one can see at q = 0, 2p there are vertical asymptotes.

Also, notice the period of the function is 2p.

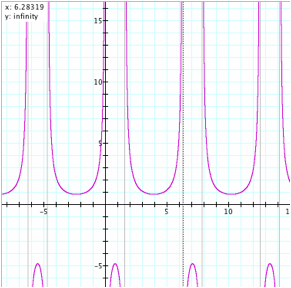
Again to find intervals of increase and decrease, minimum and maximum values, take the derivative of the function, set it equal to zero and solve for q.

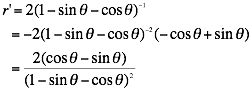
Let r’= 0, q = ,

Maxima at , n Î Z

Minima at

This lesson is great for a Calculus class, i.e., using the derivative to have students find max, min, and intervals of increase and decrease. Also, students have the opportunity to review trig functions and the idea of period, amplitude, and values of sine and cosine along the unit circle. Students will also be able to explore basic function ideas, e.g. asymptotes.

RETURN