
Jackie Gammaro
Assignment 6 - #1
1. Construct a triangle and its medians. Construct a second triangle with the three sides having the lengths of the three medians from your first triangle.
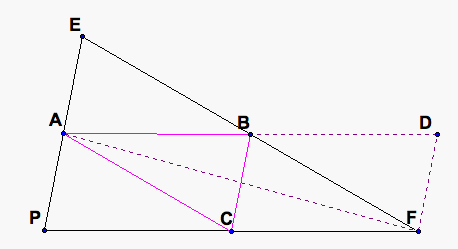
Find some relationship between the two triangles. Questions to Ponder:
(a) Is median triangle BCA congruent to the original triangle PEF? Clearly NO!
(b) Is median triangle BCA similar to the original triangle PEF? Yes.
(c) Does median triangle BCA have the same area as the original triangle PEF? If they're not congruent, they don't have the same area.
(d) Does median triangle BCA have the same perimeter as the original triangle PEF? Again, clearly NO!
(e) What is the ratio of areas for the two triangles; median triangle BCA to original triangle PEF? The ratio of areas is 1:4.
(f) What is the ratio of perimeters for the two triangles; median triangle BCA to original triangle PEF? The ratio of perimeters is 1:2.
All these I will prove, starting with part (b) -
![]()

So now, using parts of this proof, I will prove the rest of my hypothesis above.
a) From line 30, one can see corresponding sides are not congruent, thus if corresponding sides are not congruent, then triangle BCA is not congruent to triangle PEF.
c) Using the above justification that BCA is not congruent to PEF, then the areas of the two triangles are not congruent.
d) Again using line 30, since sides are the medial triangle to the original triangle are in the ratio 1:2, they're perimters will not be the same.
e) Using line 29, that all four triangles that make up the triangle PEF are congruent, and one of those triangles is medial triangle, then the ratio of areas of the medial triangle to the given triangle is 1: 4.
f) Finally, using line 30 again, if we know the sides are in the ratio 1:2, thus 2(BC+AC+AB) = (PE + EF + PF). Thus the perimeters of the medial triangle to the original triangle are in the ratio 1:2.