Roxanne Kerry


I chose to do my first writeup on the following:


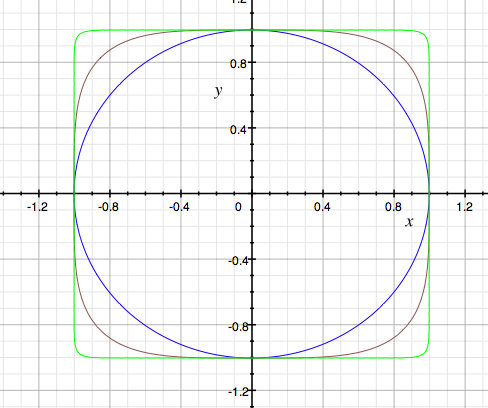
After graphing and analyzing the first equations, we can see that if we graph the even exponents, the graphs are transforming from the unit circle to a square of sides 1, a “unit square”, it seems. By graphing x24 + y24 = 1 alongside the first two graphs with even exponents, my hypothesis seemed to be confirmed (see below figure). The equation with squared exponents is the unit circle, represented by the blue graph, and the equation with the 4th power exponents is represented by the brown graph, and the equation with the 24th power exponents is represented by the green graph.



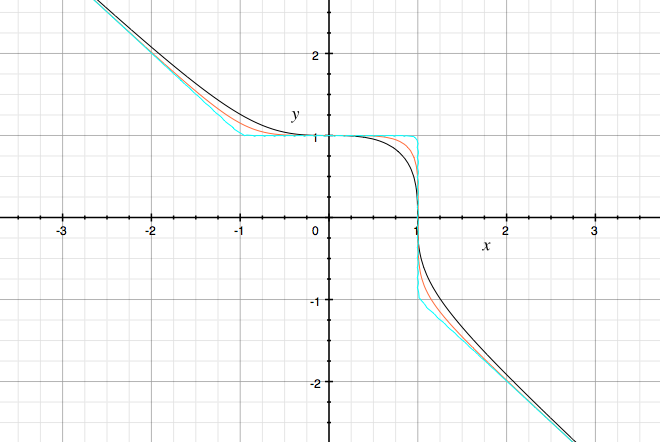
I would think that the equations with odd exponents would be somewhat of an inverse or complement to the graphs with even exponents, and in a way, they are, but not exactly as I had expected. It makes sense that the graphs with odd exponents would have the ends of the graphs approaching different directions, since any odd polynomial graphs do. These graphs with odd exponents look sort of like a linear function with slope of one going through the origin (or x=y) except for the area around the origin, where it “bubbles up” as if it were trying to wrap around the the unit circle. Just as the equations with even exponents expanded from the unit circle towards a unit square as the powers increased, with these equations with odd exponents consist of a graph resembling x=y with a middle “bump” around the origin wrapping around the unit circle approaching the unit square as the powers increase here as well (see below). The equation with exponents to the 3rd power is represented by the black graph, the equation with exponents to the 5th power is represented by the orange graph, and moving up to the equation with exponents to the 25th power the graph seems to be getting closer to wrapping around the perimeter of the unit square, and is represented by the graph in light blue.



I think this would be a good activity for high school students to explore exponents in a different way than polynomial or exponential equations. I would consider also taking this exploration one step further and see what happens when the equation is equal to a value other than one. I would expect for a similar event to happen as did with the equations equal to one, but for xn+yn = t, I would expect the graphs with even exponents to approach a square centered at the origin with side lengths of t as the values of n got larger (expanding from a circle centered at the origin with a radius of √t ) and I would expect the graphs with odd exponents to approach a graph like x=y with a “bubble” approaching a square going around the limit of the square formed by the even exponents, similar to the original problem. The graph of x2+y2 = t did give a graph of a circle with radius of √t, as expected, however the limit of these even exponent graphs still approached the unit square, as demonstrated below. The odd exponent graphs had a similar trend, approaching a graph resembling the line x=y approaching a “unit square bubble” around the origin, rather than a “t square bubble” as I predicted (also shown below).


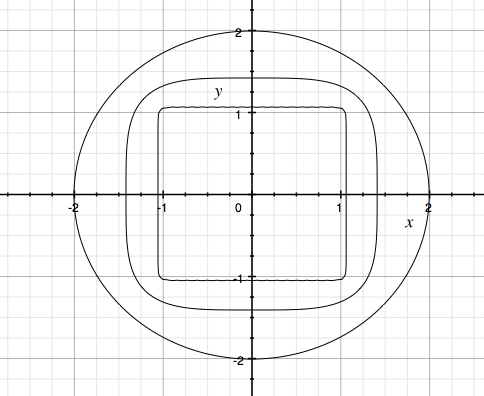
The graph of functions with even exponents



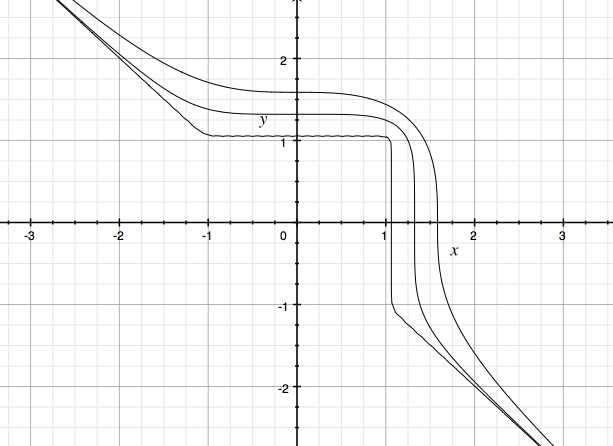
The graph of functions with odd exponents



So why does this happen with these graphs? I can't say for sure, although the conclusion we can draw from this is that the limit of any xn+yn = t (for positive n and t, at least) will approach the unit square if n is even or the line x=y with a “bubble” around the unit square if n is odd. The larger t is, the further out the graphs will start for small values of n, but as n increases, these graphs will all have the same limits, as described above. Although I would not expect my future high school students to have a good explanation as to why these graphs behave the way they do when t changes, it does help to introduce two important mathematical topics. Firstly, it distinguishes between even and odd powers. This idea comes up in many different ways, and the students will have an example here that is not polynomial as to how even and odd powered functions behave differently. This also could introduce the idea of a limit somewhat informally to the students so they will be familiar with the idea when they begin their precalculus and calculus classes.