Roxanne Kerry

For this write-up number 9, I am exploring pedal triangles and the relationship between pedal triangles of pedal triangles and the original triangles. For some reason, I had a really difficult time understanding what a pedal triangle even was, so I will begin by explaining what a pedal triangle is and how to construct one.

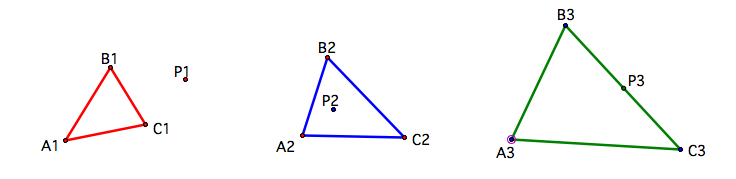
For starters, you begin with a triangle, any triangle, and a point, any point, on the plane. This point will be known as your Pedal Point. See in my illustrations below, as the triangles and their corresponding pedal points are in the same color. Notice that the pedal point can be inside the triangle, on one of the edges of the triangle, or outside of the triangle:

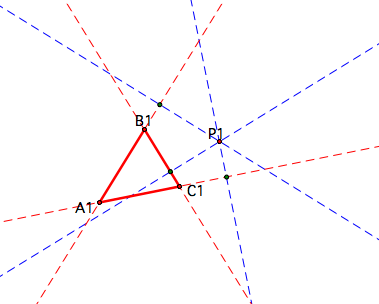
Next, you extend the segments of each side of the triangle to be lines, and construct perpendicular lines from the pedal point to each line each side of the triangle lies on, and mark those points. I will demonstrate this with the first construction, where the pedal point is outside of the triangle, but you do the same thing in any of these three cases, as shown below, where the red lines are the extensions of each of the sides, and the blue lines are the perpendicular lines from P to each side:

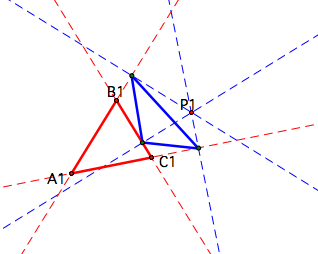
Now, we simply connect the three points we made at the intersection of the perpendicular lines, to create our pedal triangle, as follows:

The blue triangle then, from this illustration, is the pedal triangle to the original triangle, in red, given pedal point P1. Let's see what happens in our other two cases, where P1 lies inside the triangle or on one of the sides of the triangle.

Now that we see that we can construct pedal triangles given any pedal point, let's consider the pedal triangle of the pedal triangle. It is constructed in the same way as the original pedal triangle is constructed, given the first pedal triangle as the new “original triangle” for our new pedal triangle. Here are two sample constructions, one with the pedal point outside of the triangle and one with it inside the original triangle:

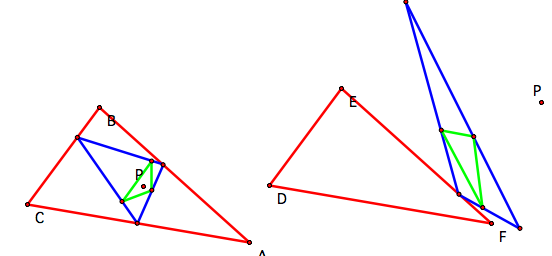
Here, the red triangles are the original triangles, the blue triangles are the pedal triangles given the pedal point, P, and the green triangles are the pedal triangles of the pedal triangles. We can do this a third time to find the pedal triangle of the pedal triangle of the pedal triangle, as follows:

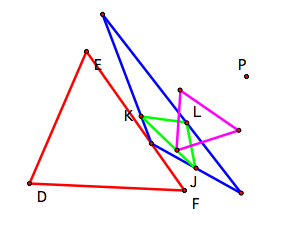
The purple triangle is now the pedal triangle of the pedal triangle of the pedal triangle of the original triangle, represented in red. Whew, that is a lot of triangles! But wait, do you see what I see? It looks as if this purple pedal triangle is similar to the original red triangle! Can it be? Let's take side and angle measures of each using GSP and see if we can confirm our suspicions:

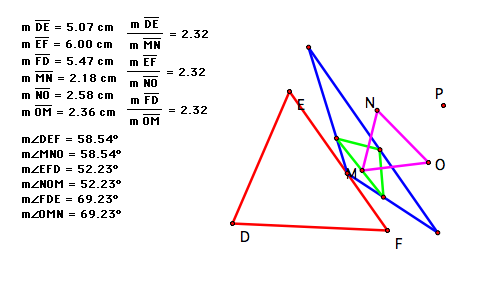
As you can see by the calculations, the sides all have the same ratio when looking at corresponding sides of the two triangles, and the corresponding angles are the same as well. Moving the point P around still gives us the same ratios and the same corresponding angle measures, thus these triangles are similar!