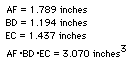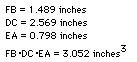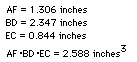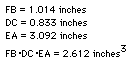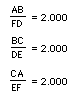EMT 668 - FINAL WRITE-UP
by
Kimberly N. Bennekin
PART I
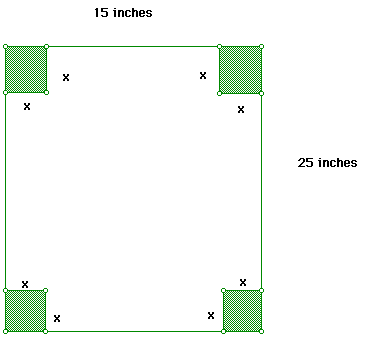
2) Given a rectangular sheet of cardboard 15 in. by 25 in. If a small
square
of the same size is cut from each corner and each side folded up along the
cuts to form a lidless box,
-What is the maximum volume of the box?
-What size(s) of the square would produce a box with a volume of 400 cubic
inches?
We want to create a box from a 15 x25 inch sheet of paper by cutting
out the corners and folding up the sides. Consider the following diagram.
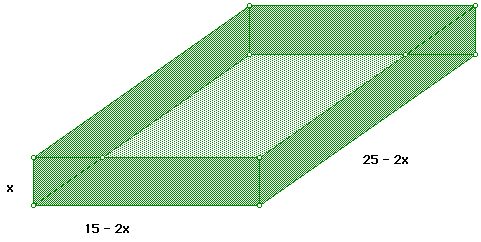
x represents the amount in inches cut in on each side.
When taking 2x units from each side, the dimensions of our box are the following:
Volume = (length)(width)(height) = (25 - 2x)(15 - 2x)(x)
The volume of a box V is found by the following formula:
V = (length)(width)(height)
Thus,
V = (25 - 2x)(15 - 2x)(x).
We want to maximize this equation. We can see when this equation is maximized
by looking at various values for x. We know that in order for our
equation to make any sense, x only exists within the interval (0,
7.5), otherwise volume will be negative. Consider the following table:
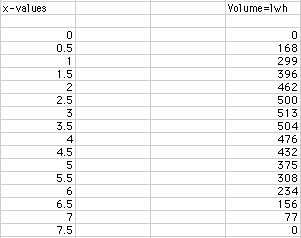
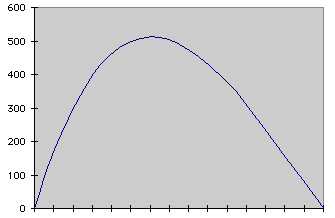
According to the table, a value of x = 3 inches will maximize the volume
of the box. If we take a look at the graph of these values we can easily
see that the maximum volume of the box is 513 cubic inches.
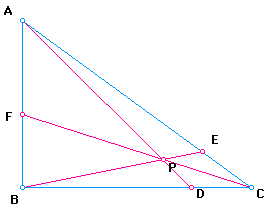
3) Consider any triangle ABC. Select a point P inside the triangle
and draw lines AP, BP, and CP extended to their intersections with the opposite
sides in points D, E and F respectively.
Consider the following triangle:

1) Explore (AF)(BD)(EC) and (FB)(DC)(EA) for various triangles and various
locations of P.
Let's consider (AF)(BD)(EC) and (FB)(DC)(EA) for the above triangle.
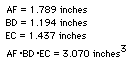
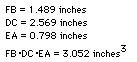
The product of the lengths of the sides are almost exact. Let's consider
a different triangle.
Let's consider the ratio of these of these products:
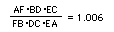
The ratio is almost 1:1.
To see if this is true for all triangles,
let's consider another triangle.
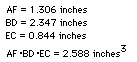
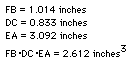
Again, the measures are almost exact (if we round to the nearest tenth,
they would be exact).
Consider the ratio of the products:
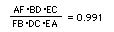
Again, we receive similar results. The ratio is almost 1:1. After exploring
other triangles using GSP, the result was the same.
PART II
Select one item from the assignments that you have not written up
for a "write-up" and that you think is representative of your
"best work" in the course.
I feel that my best write-ups
were part of the assignments already submitted. So I will select an assignment
that I learned a great deal from.
ASSIGNMENT 4, #6
Take any triangle and construct the MEDIAL triangle. Compare the Medial
triangle to the original and compare G,H, C and I of both triangles. Consider
the triangle below:
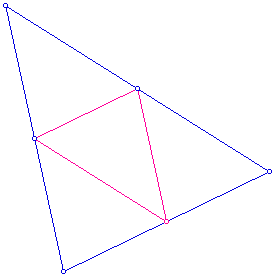
Let's begin by comparing their areas:
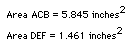
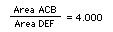
The area of the Medial triangle is exactly one-fourth of the area of
the original triangle.
Let's consider the lengths of the sides of both triangles:
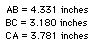

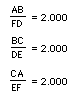
The ratio of all the sides of the triangles is 2:1. Thus, they are similar
triangles.
Let's compare G, H, C and I for both triangles. Consider the
figures below (with and without labels):

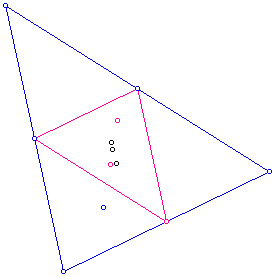
RESULTS
The Centroid (G) for both triangles are the same point. The Orthocenter
for the Medial triangle is the same point as the Circumcenter for the original
triangle. The Orthocenter for the original triangle is the only point which
is not located within the Medial triangle.
Let's consider these points for other triangles.
1) Consider the equilateral triangle below:
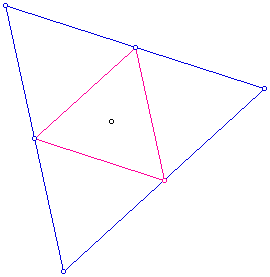
G, H, C and I are the same point for both triangles.
2) Consider the
right triangle:
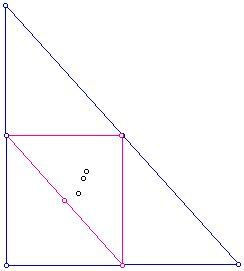
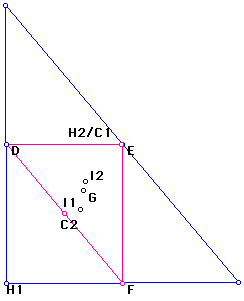
Note that the Centroid is the same point for both triangles. The Orthocenter
for the original triangle is also a vertex of the original triangle. The
Orthocenter of the Medial triangle and the Circumcenter of the original
triangle are a vertex of the Medial triangle. The Circumcenter of the Medial
triangle lies on the hypotenuse of the Medial triangle.
After many explorations, I discovered that the only points which traveled
outside of either triangle were the Circumcenters and Orthocenters of both
triangles. These explorations can be easily seen using GSP. I encourage
you to explore further.
PART III
A) Which assignment did you feel you learned the most mathematics...Why?
I felt that I learned the most mathematics/geometry from Assignment #4 (
and many others involving the use of GSP). I have not had any experience
teaching geometry and I have not taken a geometry class since high school.
I was challenged not only by having to learn the geometry, but by learning
to use GSP to explore each topic.
B) Which assignment did you feel most satisfied/happy about...Why?
I feel most happy about Assignment #2. I teach College Algebra and Business
Calculus and explore the graph of quadratic equations in much detail. I
feel that this assignment gave me many ideas on how to incorporate the explorations
of graphs (in Algebra Expressor) within explorations of various quadratic
functions.
C) Which assignment do you feel shows my most thorough effort during
the quarter?
This would be Assignment #10 - Parametric Curves and Assignment #11
- Polar Equations. I became cosumed with the interesting results I was receiving
when graphing these curves using Theorist. I feel that I explored each possible
situation and maybe some that were not needed, just to satisfy my own curiosity.
D) Which assignment opened up new elements of mathematics for you?
Again, that would be all assignments using GSP. For the same reasons
stated in part A.