Polar Equations by Jiyoon Chun
What is a polar coordinate system, and why we need to know it?
We live on the sphere. Euclidean geometry is awesome, but it does not work on the sphere. For example, the parallel axiom does not work on the sphere. Thus, we need to redefine the coordinate system which fits the situation. On the sphere, the central angle and the radius are keys of locating a point such as x and y coordinates on Cartesian plane.
Since it is based on the radius and angle, it is very convenient to express circles, and some several curves.
Investigation of some basic concepts
1. Circles of radius a, center at origin
THis is just r=a. The definition of a circle is a set of all points which are equidistant to a center. Therefore, all we have to do is finding all points which have the same radius for every angle θ. Thus, we do not mention the angle such as we do not mention y coordinate when we illustrate x=a. Take a look at the flash below; 0<r<3
2. Rays from origin
It is θ=b. In polar equation, there is no line through pole but there are rays from pole. We know that the slope of a line in cartesian plane is tangent of the angle which is measured from the x-axis to the line counterclockwise. In polar equation, the length is related to the radius, and the slope is related to the angle. Therefore, the polar equation of the ray whose angle is b is θ=b. Take a look at the flash below.
Why polar?
WHy is the name of this system polar? If we google this, we can find a lot of different historical background of this. In my opinion, I think we started to call this system polar because it look like it.
The picture above is polar plane. Let's think that we are at north pole and look down the earth. Well, just think about that we become small enough so that we can clime the earth sphere in the room. when we at north pole, we can look down the earth. Then all the longitude and latitude will look like the picture below!
Explorations
r=cosθ
Before we see the graph, I want to anticipate how the graph will be like. I think spread sheet will help understanding the shape of the graph.
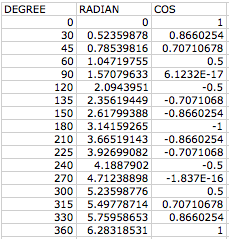
OK. Before I investigate all things, what does 6.1232E-17 mean? If you see the table above, there are two strange numbers; one is 6.1232E-17, and the other one is -1.837E-16. Well, I think this is a small disadvantage when we use calculator or spread sheet since they have some errors when they operate irrational number or 0. As we know, Actually, E in calculator means times ten to the some power. For example, 6.1232E-17=6.1232*![]() . Thus, 6.1232E-17 is a very small number, and so does -1.837E-16. I do not want to see these ugly numbers, and I changed them to 0 as below.
. Thus, 6.1232E-17 is a very small number, and so does -1.837E-16. I do not want to see these ugly numbers, and I changed them to 0 as below.
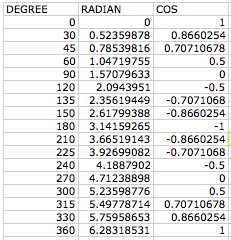
Well, we can see some negative values above, and how do we represent r=k when k<0?
Orientation
It is all about orientation. In the case of angles, when we want to represent negative value, we goes clockwise. If we want to represent negative r, then we have to go to the left. The picture below are the two points; (r,θ)=(1,60°) and (r,θ)=(1,-60°)

Now, let's investigate the curve r=cosθ.
As we see the table, five numbers are repeated: 1, 0.86, 0.7, 0.5, 0 (rounded values). I do not think it is proper to use "quadrant" since it is not cartesian system. Well, to make sense, I will just use it, but I will put the word quadrant in " ".
From 0 to 90 degree, we see the values are decreasing.
From 90 to 180, the values are negative. Therefore, the points will be plotted on " the 4th quadrant". Since the absolute values are the same, the points will be reflection of the points of the first 4.
From 180 to 270 degree, the points will be located on "the 1st quadrant" since the values are negative. Since the absolute values are the same, the points will be on the points of the first four.
From 170 to 360 degree, none of the values are negative. Therefore, they will be on "the 4th quadrant". Since the absolute values of the points from 90 to 180 are the same with the points from 270 to 360 degree, the points will be exactly on the previous four points.
Let's see the graph.
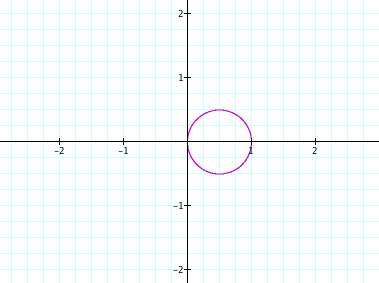
Now, we only see one circle, but we know that there are two circles which are exactly overlapping each other.
r=ncosθ
So, what does n do in front of cosθ?
It stretches out the curve. Since it simply multiplies the length, we will have a circle of diameter n. See the flash below for the better understanding. This is when -2<n<2.
What if n is negative?
The shape is not be changed, but the location will be changed. Since we have the negative value first, the points from 0 to 90 degree will be on "the 3rd quadrant". Then, as we observed above, the other points will be located on "the 2nd quadrant".
r=cos2θ
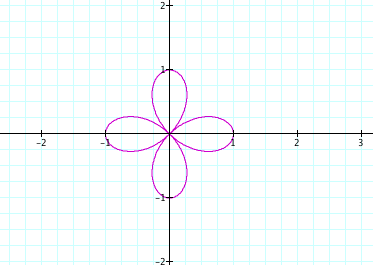
Honestly, I did not expect this shape. I thought it will be a circle which is four times repeated. However, it really makes sense if we carefully consider a period. Since the period will be π, the curve should come back to the origin for every 90k degree. In addition, when θ=π/4, 2θ=π/2. Therefore, the curve starts at (1,0) and first comes back to origin when θ=π/4. It really cannot be over the any ray of θ=kπ/4, k=0,1,2,3,4,5,6,7.
See the flash.
Does drawing order matter? Yes. as we see in the flash, the bottom leave is the second one to be drawn.
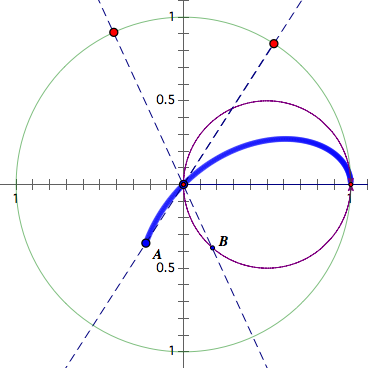
Look at the point A. It is (cos2θ, θ) where θ is between π/4 and π/2. Point B is (cos2θ, 2θ). Since cos 2θ is negative when θ is between π/4 and π/2, point B is plotted on the opposite part of the ray. The point A has the same distance from origin with point B because the angle is the same. However, it should be plotted on "the third quadrant" because it is negatively oriented.
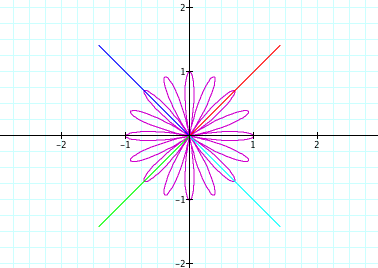
r=cos 2kθ, k is integer
In the same sense, it will be a flower of 2*2k flower leaves. The picture below is r=cos8θ.
r=cos3θ
Why does this curve only has three leaves instead of six? As you noticed it, (cos3θ,θ) follows the existing trace from 180 to 360 degree. Why?
To answer this question, we have to investigate the polar coordinate system little bit more. I want to investigate when n is 2 or 3 of nθ.
1. n=2
In case of the curve r=cos2θ, we know that the curve is a set of points which satisfies (cos 2θ, θ) I d not think we have problem when θ is from 0 to 180 degree, so I want to focus when θ is between 180 and 360 degree.
Let θ=180+α , where 0°<α<180°.
Then, (cos2θ, θ)=(cos2(180°+α°), 180°+α°)=(cos 2α°, 180°+α°) .
Now, where is (cos 2α°, 180°+α°), and how is this point related to (cos 2α°,α°)?
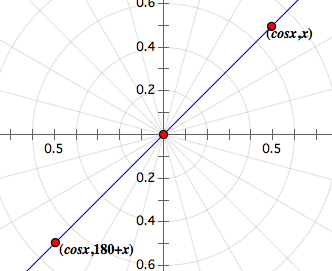
As we see the picture above, we know that two points are on the same line, but on the opposite sides.

This is why cos2θ has four leaves instead of two. We can conclude that (cosα°,α°)=(-cosα°, 180°+α°). Since they are opposite sides, we can see all the leaves.
2. n=3
Let θ=180+α , where 0°<α<180°.
Then, (cos3θ, θ)=(cos3(180°+α°), 180°+α°)=(cos 180°+3α°, 180°+α°) .
Now, where is (cos 180°+3α°, 180°+α°), and how is this point related to (cos 3α°,α°)?
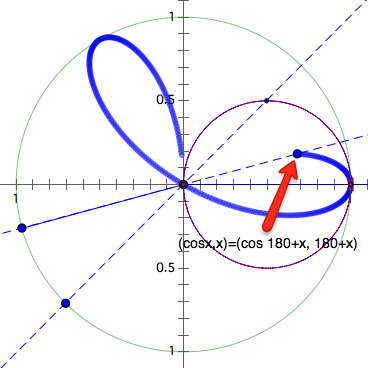
Since cos(180°+3α°)=-cos3α°, (cos 180°+3α°, 180°+α°)=(-cos3α°, 180°+α°). As we confirmed above, (-cos3α°, 180°+α°)=(-(-cos3α°), α°)=(cos3α°, α°). Therefore, the points will follow the existing point. This is why we only see half of the leaves.
Observation
Let n=2k, k be integer.
Then, 2k(180°+α)=360°k+2kα°=2kα°
When n=2k+1, (2k+1)(180°+α)=360°k+180°+α°=180°+(2k+1)α°.
Therefore, the direction will be changed once when n is even, and twice when n is odd by the property of polar system, and cos.
It is interesting that adding and subtracting π changes the orientation, and acts like a negative sign on polar system. In Cartesian plane, we see that sin and cos curves have certain periods, and similar shapes. Polar coordinate system enable us to see they have the exact same shape of the graph with different starting points. It even helps us understanding why the sign of sin stays and cos is changed when π is added to the angle. I think this activity could be pretty good for the students learning trigonometry.
r=2cos3θ
Now, it is easy to anticipate the shape of the graph. Since 2 will do only on the size, and 3 will affect the number of leaves, it will be three-leave flower which is twice bigger than cos3θ.
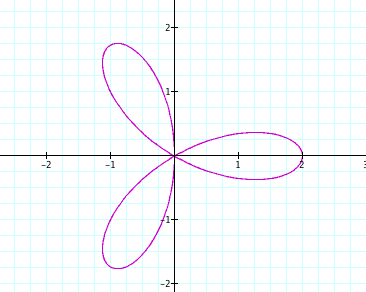
Now we have idea about acosbθ. Let's investigate r=a+bcoskθ.
r=a+bcoskθ
CASE 1: r=n+cosθ
1. r=0.1+cosθ
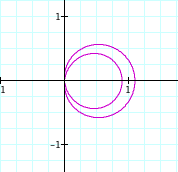
As we observe r=cosθ, it is two identical circle. In the case of r=0.1+cosθ, we can see the two circles because the value of r is slightly changed according to the angles. We see the smaller circle which is inside the bigger circle, and it happens because r is negative when the angle is between π and 2π. It was pretty predictable.
2. r=1+cosθ

Since -1<cosθ<1, 0<cosθ<2. Therefore, there will be no repeating circle because r is always positive or zero. the shape will be rounder as the adding number increases.
3. r=3+cosθ
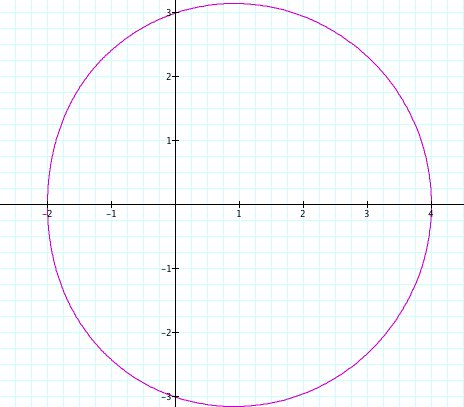
Like I mentioned above, it looks like a circle. When θ is 0 and π, it hits 4 and 2 since the value of the cos.When θ is π/2 and 3π/2, because the value of cos becomes 0. Here is the flash of r=n+cosθ, where -3<θ<3. Notice that the negative value does not affect the shape at all because it simply changes the directions in polar system.
CASE 2: r=1+cos nθ
1. r=1+cos 2θ
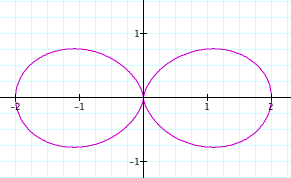
Where are other two leave?
We know that r=cosθ has four leaves, then where did other two leaves go?
Well, the answer is simple. Since 1+cos2θ is always positive, the leaves which were on the negative orientation will disappear. It means for every θ, exactly one positive r corresponds.
2. r=1+cos 3θ
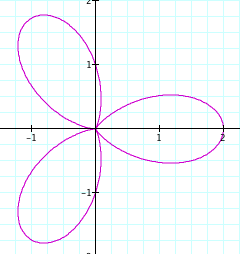
The shape of the graph is predictable because we know that the points between π<θ<2π are the same with the points between 0<θ<π, there is no change of the shape. However, the order which the leaves being drawn will be changed because 1+cos3θ is always positive. Therefore, it will be drawn in this order in the picture below.
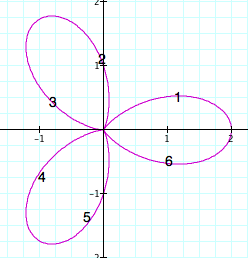
Note that r=cos 3θ was drawn 1→4→5→2→3→6.
CASE 3: r=1+cos nθ
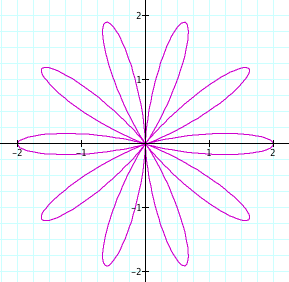
This is r= 1+cos 10θ. Thus, 1+cos nθ is a n-leaf flower. The flash below shows that r=n+ncos 10θ. all the curves have the same shape, but different sizes.
CASE 4: r=a+bcos kθ
1. r=1+2cos 3θ
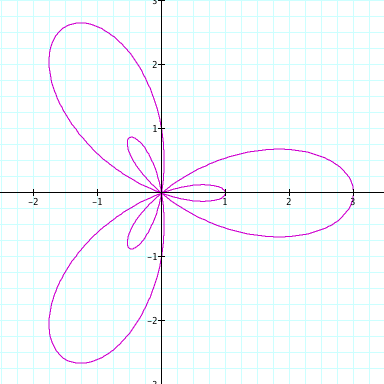
It was pretty predictable.
1) When a=b
When a=b, there are only three leaves because it will be always positive or always negative.
2) When a≠b
Then it could be negative and positive. Therefore, we have the negative oriented part of the curve. For example, 1+2cos 3θ<0 when π/6<θ< π/2. See the picture below.
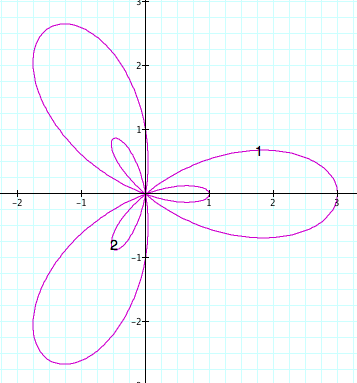
Thus, the small curve which is marked 2 will be drawn counterclockwise where π/6<θ< π/2.
Let's see the flash below.
These are the curves of r=1+ncos3θ.
When n=1, we have only three leaves.
When n>1, we see six leaves.
When n<1, we see one curve. Less the value of n is, the rounder the curve we get. The reason is pretty simple. Since the effect of cos3θ is reduced, the shape will gets similar to r=1.
I attach the flash of r=1+ncos4θ. Please pay attention to the number of leaves. The reason is pretty the same with above.
