---

Assignment 11

By: Erica Fletcher

---

 

---

 

We will first look at when a and b are equal and k is an integer. Using the equation below we will investigate what happens when we keep a and b the same and change different values for k (an integer).

Observe when a = b = k = 1 in the graph below, we see that the graph is symmetric about the x-axis.

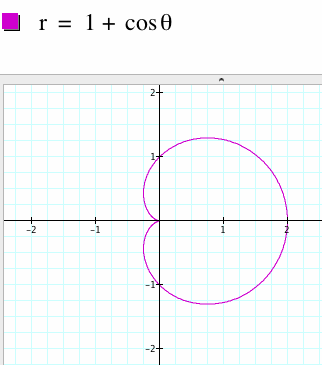
Now let's look at a = b = 1 but k = 2 this time.

Now let's investigate when a = b = 1 but k =3, then k = 5, then k = 8.

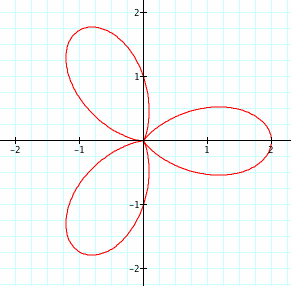
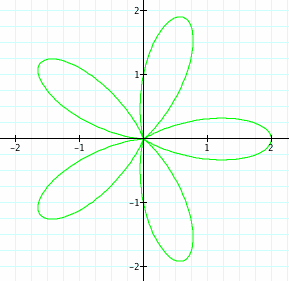
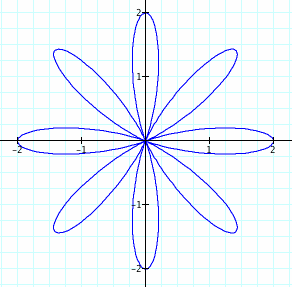
We begin to see that as the value of k increases, the number of petals on the pictures increases. Lastly as we graph more polar equations we see that the number of leaves is equal to the k value for each equation.

---

Now let's look at a = b = k = 2 and a = b =5 but the value of k stays 2. What happens as the value of a and b increases?

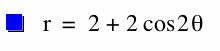
So we notice that as the value of a and b increase the graph gets larger.

---

Now let's observe when a > b.

Examine what happens when a = 3, b = 1, and k = 5.

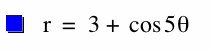
In this graph we can see that the number of leaves is still equal to k.

---

Now let's see what happens when b > a.

Take a = 1, b = 3, and k = 5.

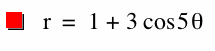
Even when b is greater than a we see that there are still 5 leaves. However, it's cool because there are 5 smaller leaves inside of the 5 larger leaves..

---

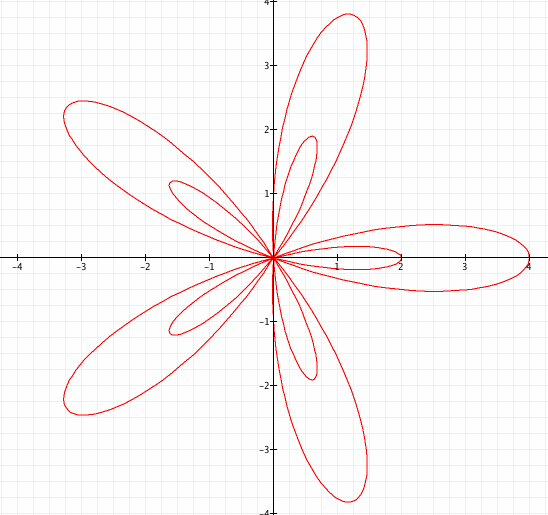
Now let's examine what happens if we add a phase shift. Take a = 1, b = 1, k = 5, and -10 < n < 10.

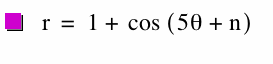
Click on the following video and observe what happens.

---

Lastly let's see what happens when we replace cos ( ) with sin ( ).

Detect what happens when a = 1, b = 1, and k = 5.

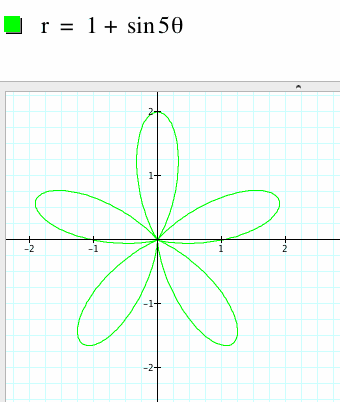
We notice that our graph looks very similar to the cosine one. The only difference one may notice right away that it is symmetric about the y-axis now as opposed to the x -axis.

---

Return to Fletcher's Home Page