
Assignment 11:
Polar Graphs
By Bennett Lewis
In this assignment we will investigate the graph of We will explore when a and b are equal such that k is an integer.
Then we will compare and contrast this with this equation.
Lastly, we will investigate the changes, if any, that occur if cosine is changed to sine.
The standard graph for this equation, when a = b = k = 1 is seen below.
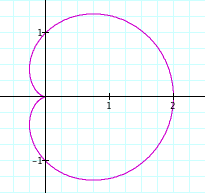
Since our variables all equal one, the standard cosine equation would result.
Now let’s investigate when a = b = 2, with various k values as an integer.
When k = 2, what should we expect? When k = 1, the graph showed one “loop”.
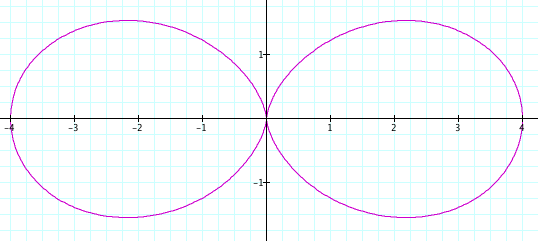
Here we have two “loops” when k = 2. Notice that the graph resembles a “figure 8”.
What will happen if k = 3? How many loops should be expect? Below is the graph, with k = 3. Notice there are 3 loops
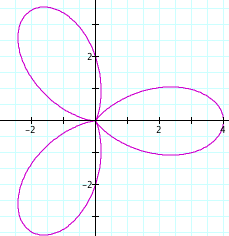
The pattern is simple. Whatever k is equal to (as an integer), correlates to the number of loops that will exist.
k = 4 |
k = 5 |
k = 6 |
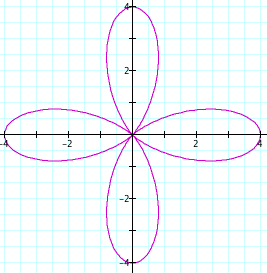 |
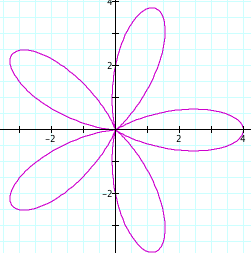 |
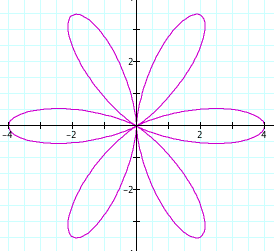 |
Click the link here to see what happens as k increases.
Now, we want to know how a and b affect the graph. If we set a parameter on a for one equation and b for another, what should we expect to see? Check out this video here to see what happens as the two parameters vary.
The purple graph shows the parameter a varying and b = 2 and k = 3. The red graph shows the parameter b varying and a = 2 and k = 3.
We notice from these two graphs that as a increases for the purple equation, the shape of the graphs acts as a “barrier” to the red graph and looses the loops. We notice the shape of the red graph expands, but keeps the loops intact. The red graph helps compare equation to
![]()
to
![]()
When n = 0 for the red equation, then we have this new polar equation. In this new equation we can see how b and k affect the shape of the polar graph. From this animation here, we can see the role that negative and positive integers have on the transformation of the original parent graph.
In contrast to the original equation, this polar equation keeps k loops as b increases where varying a changes the shape of the graph entirely.
Now, we will investigate what happens when we replace cosine with sine. What do we think will happen?

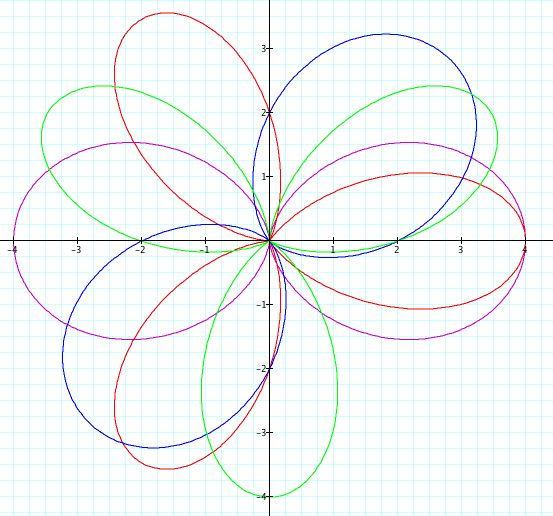
The difference between the sine and cosine graphs is the direction of shape of the graph varies. For example, the blue and the purple graphs are still for k = 2, but notice that the blue graph is rotated. The same is true for the red and green graphs, but k = 3. If we add a translation to our first polar equation in which π/2 is added inside the parenthesis, then our original graph with cosine appears.
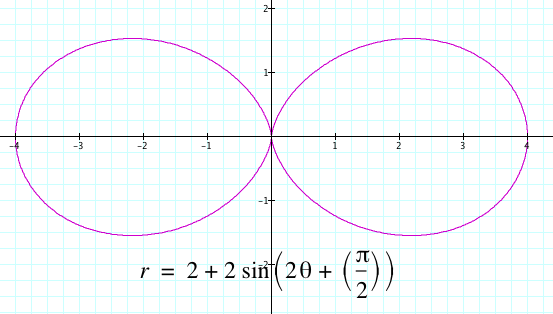

So we see that the difference between the sine graph and the cosine graph is a matter of rotation by π/2.