Polar Equations
By Leighton McIntyre
Goal : to investigate the polar equations 
and 
with a=b=k, and k having integer values and show the effects of changing the cos in the equations to sin.
Polar Equations
Consider the equation with a=b and k having integer values. The graphed form of this equation will look like an n-leaf rose. We will investigate how the shape changes as we consider different values of a, b and k.
First Equation
For the first equation we select the following values to graph of
a=b=k=1,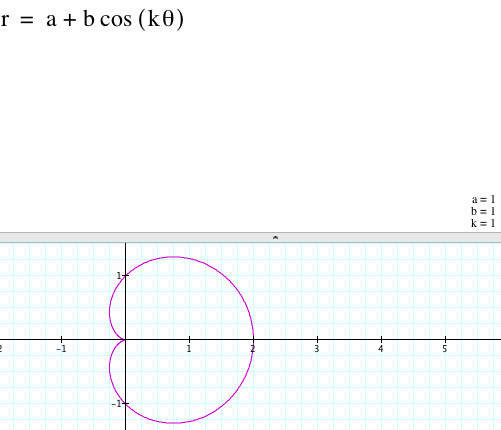 
Lets look at the graphs of more odd values of k.
a=b=k=3, a=b=k=5, a=b=k=9.
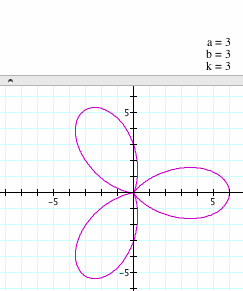
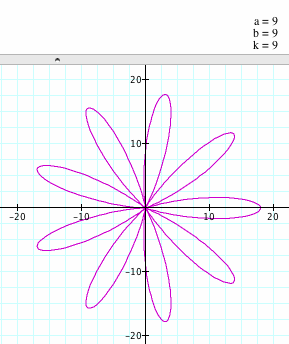
Notice that the graphs are symmetric about the x-axis and the number of loops from the center equals the values of k.
Lets look the graphs of even values of k.
a=b=k=2, a=b=k=4, a=b=k=6, a=b=k=8, a=b=k=10
.
,
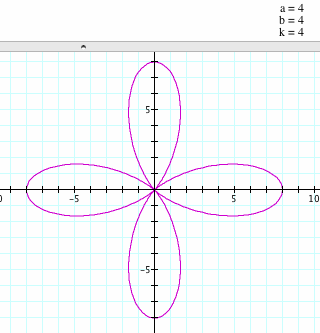
Again, the graphs are symmetric about the x-axis and the number of loops from the center equals the values of k. These numbers were not chosen for any specific reason but merely to show that the number at a=b=k=n, n will represent the number of leaves on the rose or the number of loops form the center of the graph. All the graphs are symmetric along the x-axis.
Second Equation
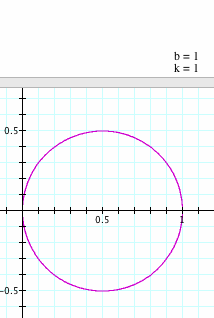
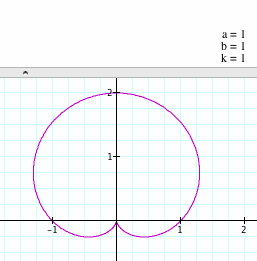
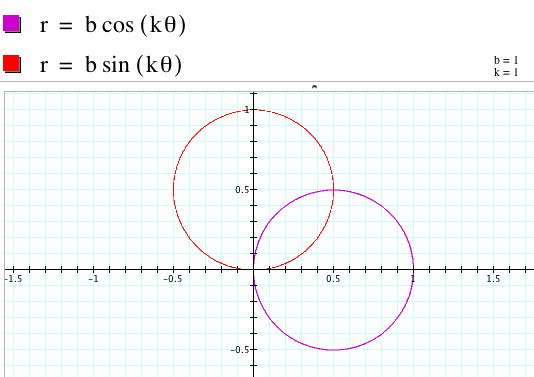
We now consider the equation with b=k and k having integer values we start with b=k=1. the figure is symmetric in the x-axis.
We now look at the graph of this function for other odd integer values of k: b=k=3, b=k=5, b=k=7
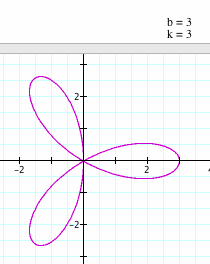
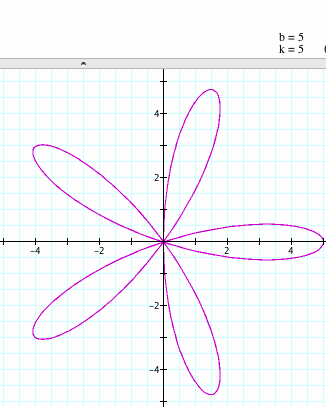
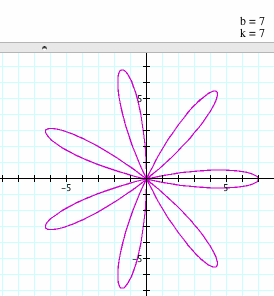
Note that in these cases the number of leaves or loops from the center equals the values of k. The graphs are symmetric about the x-axis.
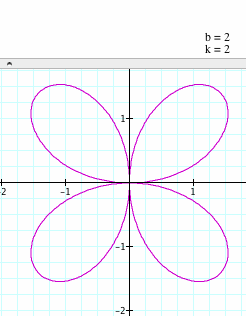
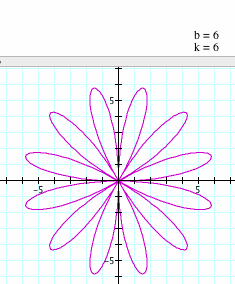
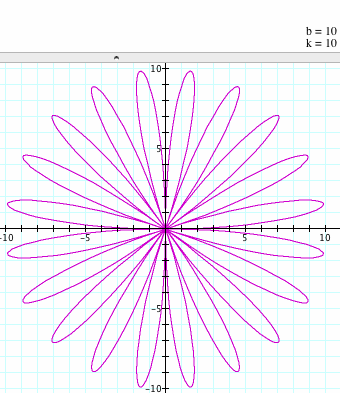
We now look at the graph of this function for even integer values of k: b=k=2, b=k=4, and b=k=10.
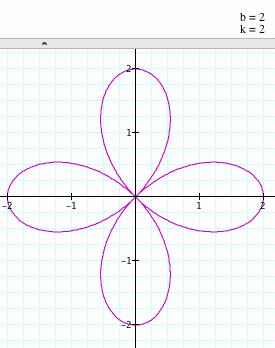
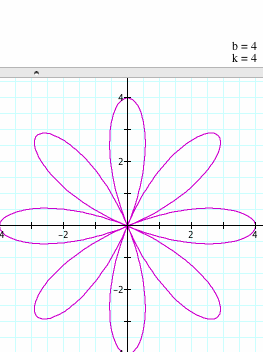
Notice here that the number of leaves or lops for the center doubles the values of k in each of the diagrams. The diagrams are symmetric in the x and y axes.
Third Equation 
We now consider a situation where the cos in the above equations is replaces by sin. Thus we investigate a third equation corresponding to the first equation of the form
Notice that this leaf is symmetric along the y-axis. and looks like a rotation of 90 degrees counter clockwise of the figure in equation 1.
We then proceed to look at the cases where k consists of even numbers and see for the graphs where a=b=k=2, a=b=k=6, and a=b=k=10.
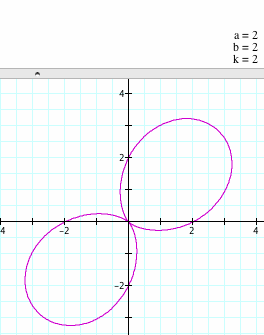
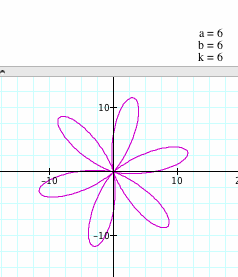
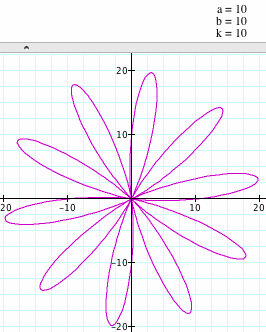
Notice here that the shapes have the numbers of leaves or loops from the center corresponding to the value of k=n. None of the leaves lie exactly on the x-axis or the y-axis.
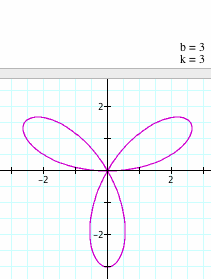
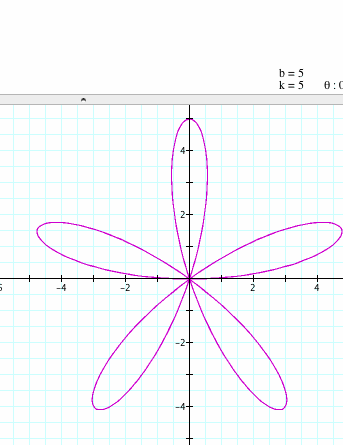
We now investigate the equation for the other odd values of k.
a=b=k=3, a=b=k=7, a=b=k=9
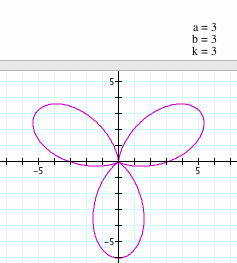
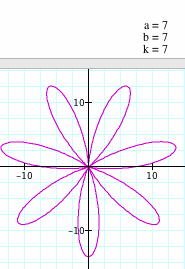
Notice that the number of leaves correspond to the value of k and the figure is symmetric in the y-axis.
Fourth Equation r = 
We finally investigate the equation r = and show how the figures change relative to the equation .
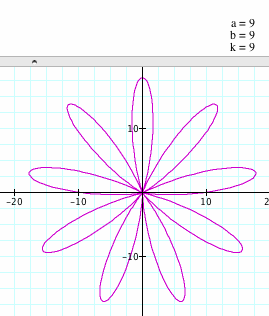
When the cos changes to sin then the figure rotates from being symmetric in the x-axis to being symmetric in the y-axis.
Investigations with the odd numbered k's show symmetry in the y-axis and the number of loops from the center n=k.
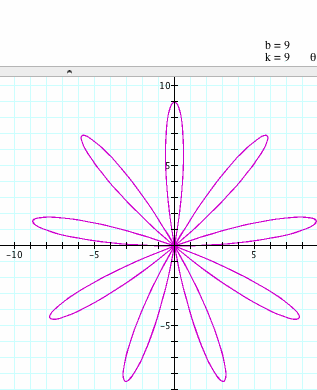
Investigations with the even numbered k's show that the number of loops from the center doubles for even numbered k's. The shapes are symmetric along the y-axis.
These investigations with nice integer values for k, and where a=b=k and b=k, do not give the full picture of the actual rotations that occur as the values change. You can get a more vivid picture when the graphs are allowed to change dynamically. Check out these investigations further using the following links.
Dynamic equation 1, Dynamic equation 2, Dynamic equation 3, Dynamic equation 4.
Summary
1. For investigations with equation we find that the shapes are symmetric along the x-axis and the value of k determines the number of loops from the center where the value of k equals the number of loops from the center.
2. For investigations with equation we find that the shapes are symmetric along the x-axis and the value of k determines the number of loops from the center where the value of k equals the number of loops from the center for odd values of k only. For even values of k the number of loops from the center doubles that of the value of k.
3. For investigations with equation we find that the shapes are symmetric along the y-axis for odd values of k only, and the value of k determines the number of loops from the center where the value of k equals the number of loops from the center.
4. For investigations with equation r = we find that the shapes are symmetric along the y-axis and the value of k determines the number of loops from the center where the value of k equals the number of loops from the center for odd values of k only. For even values of k the number of loops from the center doubles that of the value of k.