Square inside a Semi-Circle

by

Hieu Huy Nguyen

Given a semicircle with an inscribed square of side s. Let a be the length of the diameter on each side of the square. We want to find the ratio of s to a. What is ?

The illustration of the figure is displayed below.

We notice that there is a side of the square located on the diameter of the semicircle. Since the length of the diameters a area equal on both sides of side s on the diameter, we can conclude that the midpoint on that particular side s is also the midpoint of the diameter of the circle. So the radius r of the semi circle can be determined as follows r = (1/2)s + a.

We also know that a segment formed from the midpoint of the semi circle to the upper right vertex of the square is also the radius of the semi circle. Therefore, it is possible to form a right triangle and generate another expression for the radius based on the Pythagorean theorem. The picture of the radius is illustrated below.

Using the Pythagorean theorem, another equation for radius r was derived below:

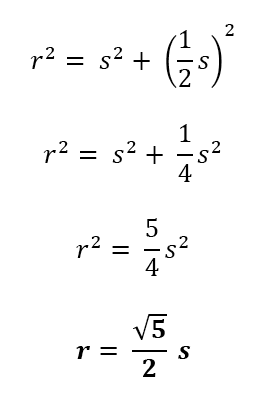
We know have two equations for the radius. We can set them equal to each other and solve for the ratio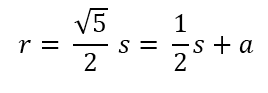

The derivation is as follows:

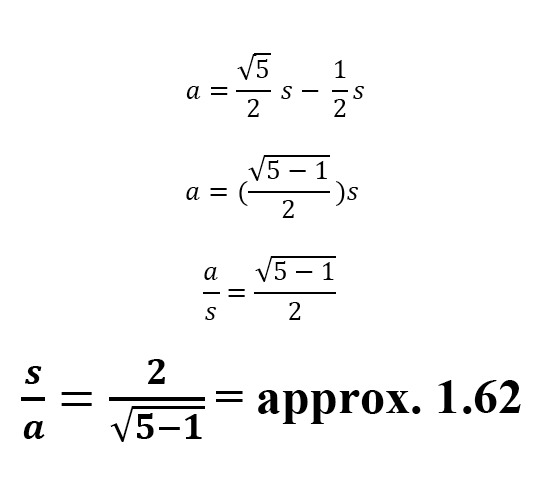
Go back to Hieu's HOME PAGE

Go back to EMAT 6680 HOME PAGE