Assignment 9: Pedal Triangles
By:
Jonathan Sabo
For
this writeup we will explore what happens to the pedal triangle if the
pedal point is the centroid, incenter, circumcenter, or orthocenter of
a traingle.
We will start by observing what happens if pedal point P is the centroid of triangle ABC.
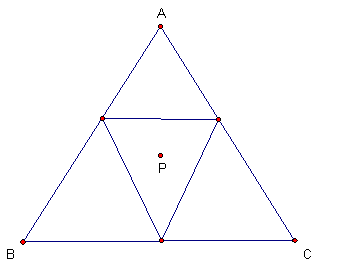
We will start by observing what happens if pedal point P is the centroid of triangle ABC.

We
notice that when the triangle is an acute triangle, the pedal triangle
remains inside of the original triangle. This is similar to the
actual centroid. Whenever the triangle is acute the centroid is
going to stay inside of the triangle.
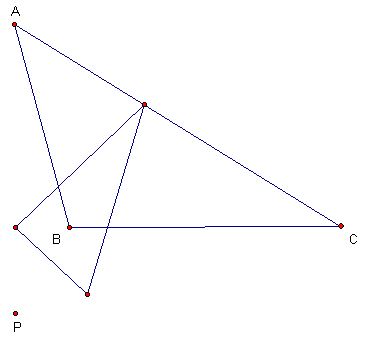

When
the original triangle is an obtuse triangle, part of the pedal triangle
will move outside of the original triangle. This is happening
because the centroid is leaging the triangle, so the pedal triangle is
following the centroid out of the triangle. Both the centroid and
the pedal triangle leave through the vertices of the triangle.
Now we will observe what happens if pedal point P is the incenter of triangle ABC
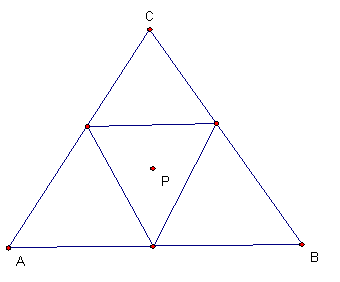 .
.
Now we will observe what happens if pedal point P is the incenter of triangle ABC
 .
.We
can see that if pedal point P is the Incenter of the triangle then the
pedal triangle will lie inside of the original triangle. This
will happen for all cases if the original triangle is right, acute or
obtuse. The pedal triangle will never leave the original triangle.
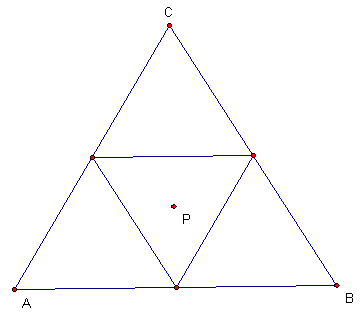
Now we will observe what happens if pedal point P is the circumcenter of triangle ABC.
Now we will observe what happens if pedal point P is the circumcenter of triangle ABC.

If
the original triangle is an acute triangle we can see that the pedal
triangle stays inside of the original triangle. All of the
vertices of the pedal triangle are at the midpoints of the sides of the
original triangle and will always stay inside of the original triangle.


If
the original triangle is an obtuse triangle the pedal point P will be
on the outside of the original triangle. Whenever the pedal point
leaves the original triangle it will pass through the midpoint of the
side that it is going through.
Now we will observe if the pedal point P is the Orthocenter of triangle ABC.
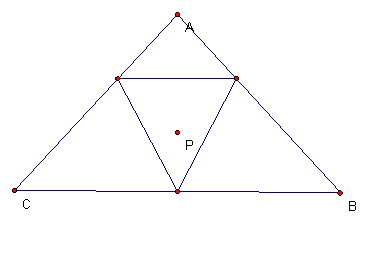
Now we will observe if the pedal point P is the Orthocenter of triangle ABC.

We
can see that if pedal point P is the Orthocenter it will always lie
inside of the original triangle. If the original triangle is an
acute triangle the pedal triangle will always lie inside of the
original triangle. There are some cases where the pedal triangle
will be outside of an obtuse triangle.
Back to Home Page
Back to Home Page