by: Doris Santarone

Assignment #4: The Nine Point Circle

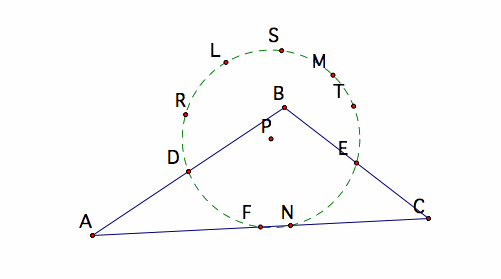
The Nine Point Circle is the circle that is formed by passing through 9 significant points. The points are: the 3 midpoints of the sides of a triangle, the 3 feet of the altitudes of the triangle, and the 3 midpoints of the line segment from the vertex to the triangle's orthocenter.

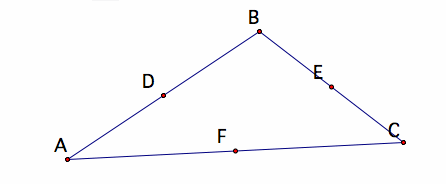
First, I want to construct the midpoints of the sides of triangle ABC. I will call these points D, E, and F.

 

 

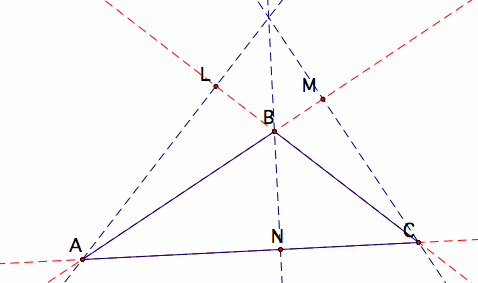
Next, I will construct the altitudes of the triangle ABC. I will call the feet of these altitudes points L, M, and N.

 

 

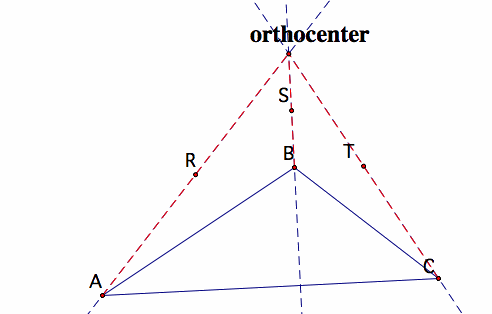
Now, I will construct the line segments from each vertex to the triangle's orthocenter. I will call the midpoints of these line segments points R, S, and T.

 

 

Let's bring all 9 points together on triangle ABC.

 

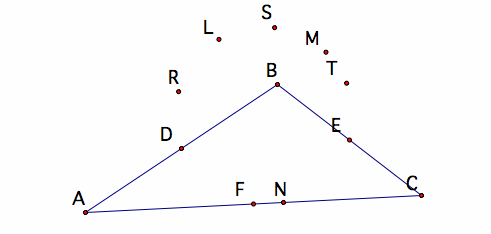
In order to construct the circle that passes through these 9 points, I need to find the triangle's center. Below are the steps that will obtain the center of the circle, which I will call P.

1) Construct a segment between two points on the circle. I will construct segment RL.

2) Construct the perpendicular bisector of segment RL. All points on the perpendicular bisector are equidistant to the points R and L.

3) Construct another segment between two different points on the circle. I will construct segment TE.

4) Construct the perpendicular bisector of segment TE. All points on the perpendicular bisector are equidistant to the points T and E.

5) Construct the point of intersection of the perpendicular bisectors. Since this point is on both perpendicular bisectors, then it is equidistant to points R, L, T, and E.

6) Since this point, which I will name P, is equidistant to four points on the circle, then it is the center of the circle.

 

Finally, I will construct the circle, with center P, and radius PR (or PL, or PS, etc.)

 

 

Click here for the GSP Sketch, which includes a script for the Nine Point Circle.

---

Back to Homepage

---